Ex 7.2, 38 - Integration 10x9 + 10x loge 10 / x10 + 10x

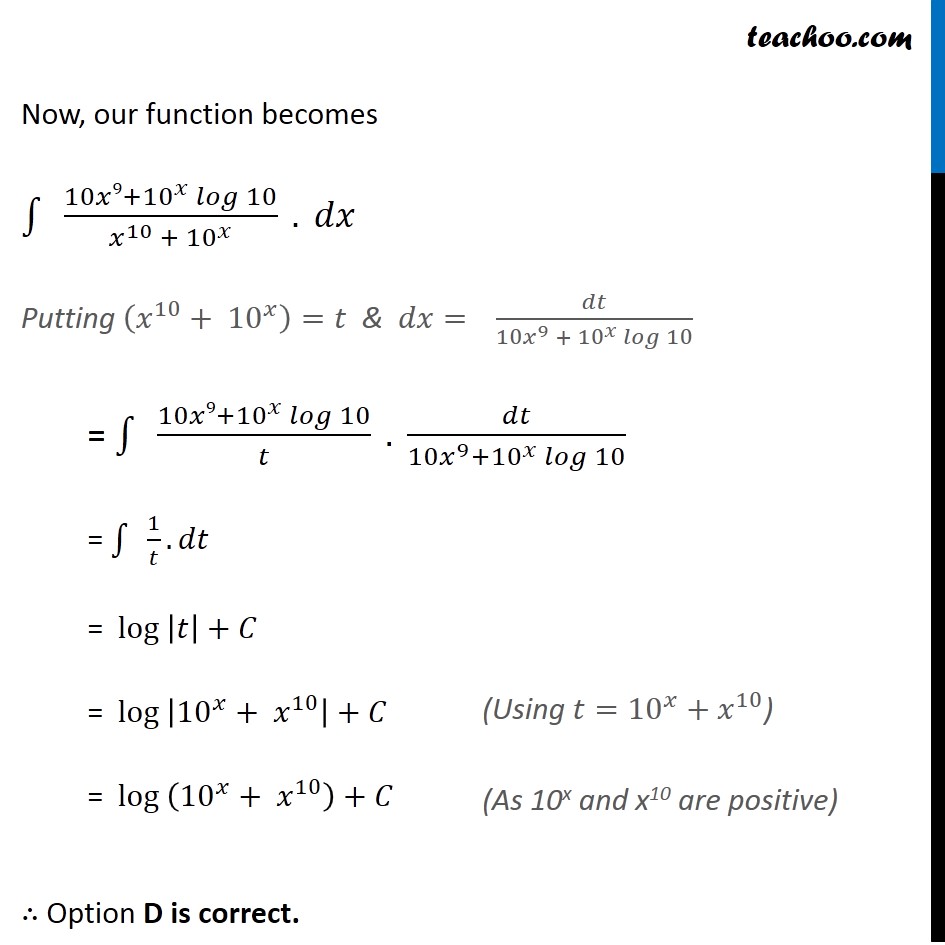
Ex 7.2, 38 ∫1▒(〖10𝑥〗^9+〖10〗^𝑥 log_𝑒⁡10)/(𝑥10+ 10𝑥) dx equals (A) 10𝑥 – 𝑥^10 + 𝐶 (B) 10𝑥+𝑥^10+𝐶 (C) (10𝑥 – 𝑥^10 )^(−1) + 𝐶 (D) log⁡(10𝑥+𝑥10) + 𝐶 Let 𝑥10+ 10𝑥= 𝑡 Differentiating both sides 𝑤.𝑟.𝑡.𝑥 〖10𝑥〗^(10−1)+〖10〗^𝑥 𝑙𝑜𝑔⁡10= 𝑑𝑡/𝑑𝑥 〖10𝑥〗^9+〖10〗^𝑥 𝑙𝑜𝑔⁡10= 𝑑𝑡/𝑑𝑥 𝑑𝑥= 𝑑𝑡/(〖10𝑥〗^9 + 〖10〗^𝑥 𝑙𝑜𝑔⁡10 ) (Using (𝑎^𝑥 )^′=𝑎^𝑥 𝑙𝑜𝑔⁡𝑎) Now, our function becomes ∫1▒〖" " (10𝑥〖9+10〗^𝑥 𝑙𝑜𝑔⁡10)/(𝑥^10 + 〖10〗^𝑥 )〗 . 𝑑𝑥 Putting (𝑥^10+ 〖10〗^𝑥 )=𝑡 & 𝑑𝑥=" " 𝑑𝑡/(〖10𝑥〗^9 + 〖10〗^𝑥 𝑙𝑜𝑔⁡10 ) = ∫1▒〖" " (10𝑥〖9+10〗^𝑥 𝑙𝑜𝑔⁡10)/𝑡〗 . 𝑑𝑡/(〖10𝑥〗^9+〖10〗^𝑥 𝑙𝑜𝑔⁡10 ) " " = ∫1▒〖" " 1/𝑡〗.𝑑𝑡 = log |𝑡|+𝐶 = log |〖10〗^𝑥+ 𝑥^10 |+𝐶 = log (〖10〗^𝑥+ 𝑥^10 )+𝐶 ∴ Option D is correct. (Using 𝑡=〖10〗^𝑥+𝑥^10) (As 10x and x10 are positive)

Ex 7.2, 38 - Chapter 7 Class 12 Integrals