Misc 1 - Chapter 7 Class 12 Integrals

Transcript

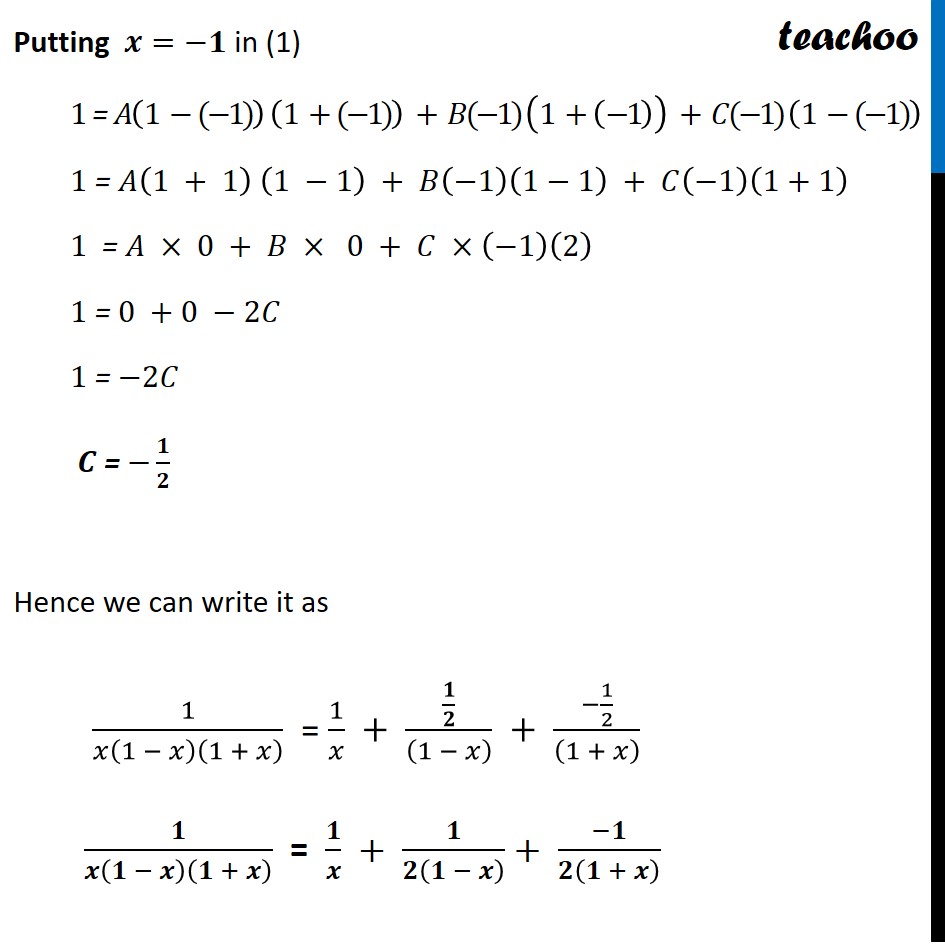
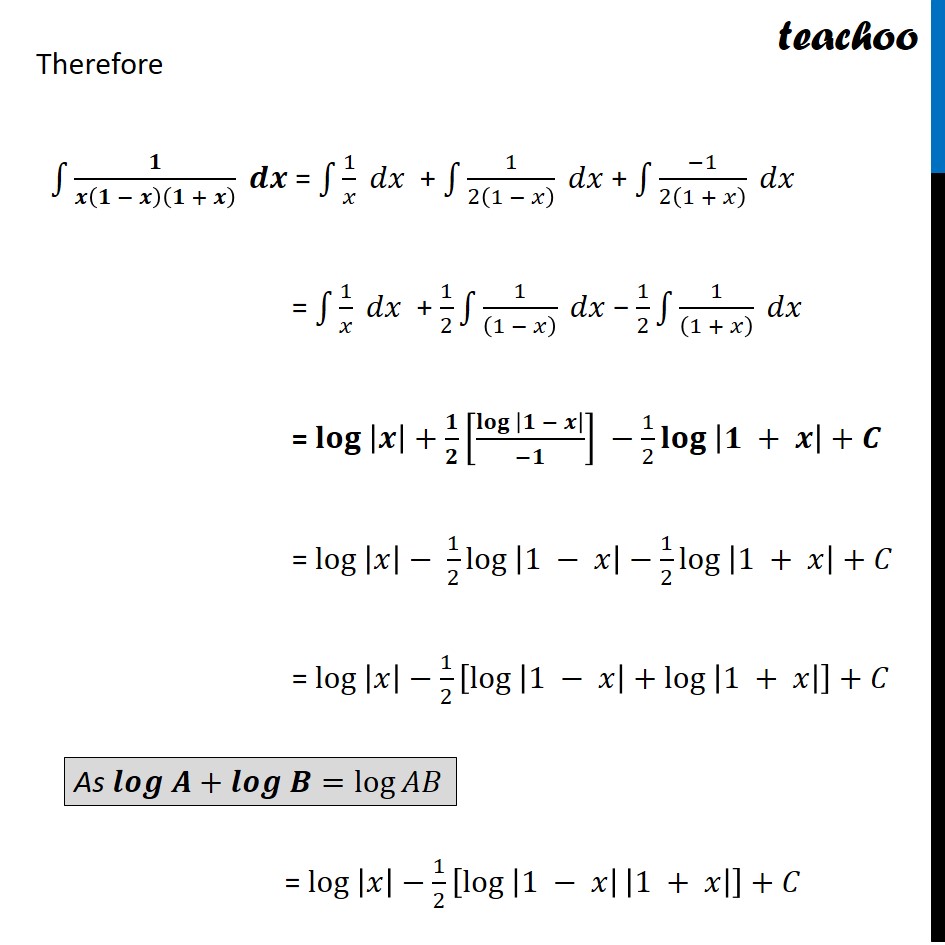
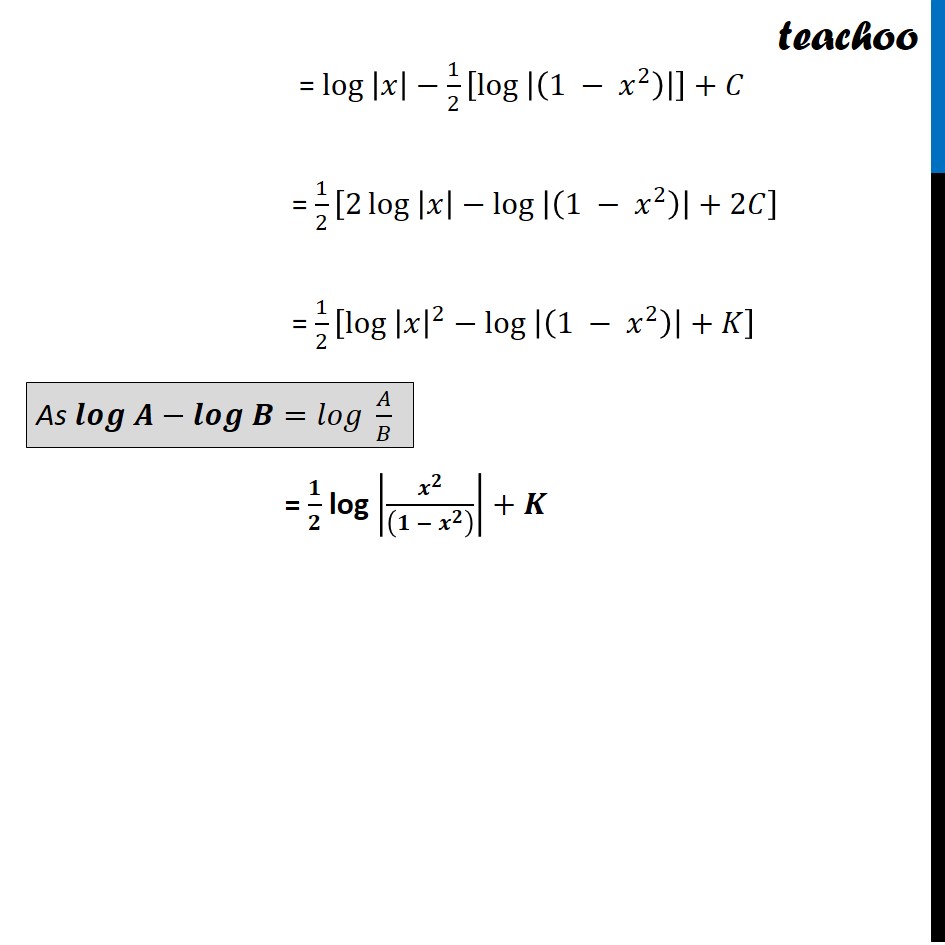
Misc 1 (Method 1) Integrate the function 1/(𝑥 − 𝑥^3 ) Solving integrand 1/(𝑥 − 𝑥^3 )=1/𝑥(1 − 𝑥^2 ) =𝟏/𝒙(𝟏 − 𝒙)(𝟏 + 𝒙) We can write it as 𝟏/𝒙(𝟏 − 𝒙)(𝟏 + 𝒙) = 𝑨/𝒙 + 𝑩/((𝟏 − 𝒙) ) + 𝒄/((𝟏 + 𝒙) ) 1/𝑥(1 − 𝑥)(1 + 𝑥) = (𝐴(1 − 𝑥) (1 + 𝑥) + 𝐵𝑥 (1 + 𝑥) + 𝐶𝑥 (1 − 𝑥))/( 𝑥 (1 − 𝑥) (1 + 𝑥) ) Cancelling denominator 𝟏 = 𝑨(𝟏 − 𝒙) (𝟏 + 𝒙) + 𝑩𝒙 (𝟏 + 𝒙) + 𝑪𝒙 (𝟏 − 𝒙) Putting 𝒙=𝟎 in (1) 1=𝐴(1 − 0) (1 + 0) + 𝐵(0) (1 + 0) + 𝐶(0) (1 − 0) 1=𝐴 × 1 × 1 + 𝐵 × 0 + 𝐶 × 0 1=𝐴+0+0 𝑨=𝟏 Putting 𝒙=𝟏 in (1) 1 = 𝐴(1 −1) (1 +1) + 𝐵(1) (1 +1) + 𝐶(1) (1 −1) 1 = 𝐴 × 0 + 𝐵 × (1) × (2) + 𝐶 × 0 1 = 0 +2𝐵 + 0 𝑩 = 𝟏/𝟐 Putting 𝒙=−𝟏 in (1) 1 = 𝐴(1 −(−1)) (1 +(−1)) + 𝐵(−1) (1 +(−1)) + 𝐶(−1) (1 −(−1)) 1 = 𝐴(1 + 1) (1 −1) + 𝐵(−1)(1−1) + 𝐶(−1)(1+1) 1 = 𝐴 × 0 + 𝐵 × 0 + 𝐶 ×(−1)(2) 1 = 0 +0 −2𝐶 1 = −2𝐶 𝑪 = −𝟏/𝟐 Hence we can write it as 1/𝑥(1 − 𝑥)(1 + 𝑥) = 1/𝑥 + (𝟏/𝟐)/((1 − 𝑥) ) + (−1/2)/((1 + 𝑥) ) 𝟏/𝒙(𝟏 − 𝒙)(𝟏 + 𝒙) = 𝟏/𝒙 + 𝟏/𝟐(𝟏 − 𝒙) + (−𝟏)/𝟐(𝟏 + 𝒙) Therefore ∫1▒𝟏/𝒙(𝟏 − 𝒙)(𝟏 + 𝒙) 𝒅𝒙 = ∫1▒1/𝑥 𝑑𝑥 + ∫1▒1/2(1 − 𝑥) 𝑑𝑥 + ∫1▒(−1)/2(1 + 𝑥) 𝑑𝑥 = ∫1▒1/𝑥 𝑑𝑥 + 1/2 ∫1▒1/((1 − 𝑥) ) 𝑑𝑥 − 1/2 ∫1▒1/((1 + 𝑥) ) 𝑑𝑥 = 〖𝐥𝐨𝐠 〗⁡|𝒙|+𝟏/𝟐 [〖𝐥𝐨𝐠 〗⁡|𝟏 − 𝒙|/(−𝟏)] −1/2 〖𝐥𝐨𝐠 〗⁡|𝟏 + 𝒙|+𝑪 = 〖log 〗⁡|𝑥|− 〖 1/2 log 〗⁡|1 − 𝑥|−1/2 〖log 〗⁡|1 + 𝑥|+𝐶 = 〖log 〗⁡|𝑥|−1/2 [〖log 〗⁡|1 − 𝑥|+〖log 〗⁡|1 + 𝑥| ]+𝐶 = 〖log 〗⁡|𝑥|−1/2 [〖log 〗⁡|1 − 𝑥| |1 + 𝑥|]+𝐶 As 𝒍𝒐𝒈 𝑨+𝒍𝒐𝒈 𝑩=log⁡𝐴𝐵 = 〖log 〗⁡|𝑥|−1/2 [〖log 〗⁡|(1 − 𝑥^2 )| ]+𝐶 = 1/2 [2 〖log 〗⁡|𝑥|−〖log 〗⁡|(1 − 𝑥^2 )|+2𝐶] = 1/2 [〖log 〗⁡〖|𝑥|^2 〗−〖log 〗⁡|(1 − 𝑥^2 )|+𝐾] = 𝟏/𝟐 log |𝒙^𝟐/((𝟏 − 𝒙^𝟐 ) )|+𝑲 As 𝒍𝒐𝒈 𝑨−𝒍𝒐𝒈 𝑩=𝑙𝑜𝑔 𝐴/𝐵 Misc 1 (Method 2) Integrate the function 1/(𝑥 − 𝑥^3 ) Now, ∫1▒〖1/(𝑥 − 𝑥^3 ) 𝑑𝑥〗 Taking x3 common from the denominator =∫1▒1/(𝑥^3 (𝑥/𝑥^3 − 1) ) 𝑑𝑥 =∫1▒𝟏/(𝒙^𝟑 (𝟏/𝒙^𝟐 − 𝟏) ) 𝒅𝒙 Let t = 𝟏/𝒙^𝟐 −𝟏 Differentiating with respect to 𝑥 𝑑/𝑑𝑥 (1/𝑥^2 −1)=𝑑𝑡/𝑑𝑥 (−2)/𝑥^3 =𝑑𝑡/𝑑𝑥 𝒅𝒙=(𝒙^𝟑 𝒅𝒕)/(−𝟐) Putting the value t and dt in the equation ∫1▒〖𝟏/(𝒙^𝟑 (𝟏/𝒙^𝟐 −𝟏) ) 𝒅𝒙〗=∫1▒〖1/(𝑥^3 (𝑡) ) × (𝑥^3 𝑑𝑡)/(−2)〗 =∫1▒〖𝟏/(−𝟐) 𝒅𝒕/𝒕〗 =(−1)/( 2) ∫1▒𝑑𝑡/𝑡 =(−1)/( 2) 𝑙𝑜𝑔|𝑡|+𝐶 Putting back 𝒕=𝟏/𝒙^𝟐 −𝟏 =(−1)/( 2) 𝑙𝑜𝑔|1/𝑥^2 −1|+𝐶 =(−1)/( 2) 𝑙𝑜𝑔|(𝟏 − 𝒙^𝟐)/𝒙^𝟐 |+𝐶 = 1/2 log |(1 − 𝑥^2)/𝑥^2 |^(−𝟏)+𝐶 = 𝟏/𝟐 log |𝒙^𝟐/(𝟏 − 𝒙^𝟐 )|+𝑪 (As a log b = log 𝑏^𝑎)