Ex 7.2, 1 - Chapter 7 Class 12 Integrals
Last updated at Dec. 16, 2024 by Teachoo
Ex 7.2
Ex 7.2, 1
You are here
You are here
Transcript
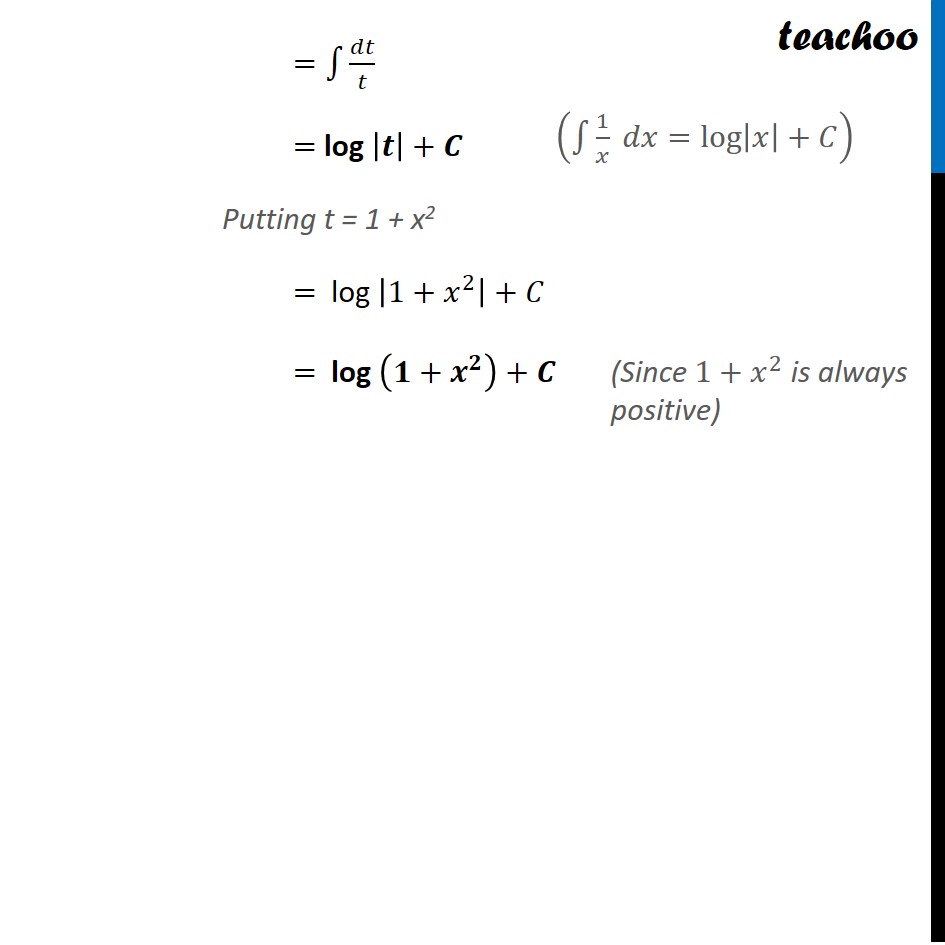
Ex 7.2, 1 Integrate the function: 2𝑥/(1 + 𝑥2) We need to find ∫1▒𝟐𝒙/(𝟏 + 𝒙𝟐) 𝒅𝒙 Let 𝟏 + 𝒙𝟐 = 𝒕 Differentiating 𝑤.𝑟.𝑡.𝑥 2𝑥=𝑑𝑡/𝑑𝑥 𝒅𝒙=𝒅𝒕/𝟐𝒙 Thus, our equation becomes ∫1▒𝟐𝒙/(𝟏 + 𝒙𝟐) 𝒅𝒙 =∫1▒2𝑥/𝑡 . 𝑑𝑡/2𝑥 =∫1▒𝑑𝑡/𝑡 = log |𝒕|+𝑪 Putting t = 1 + x2 = log |1+𝑥^2 |+𝐶 = log (𝟏+𝒙^𝟐 )+𝑪 (∫1▒〖1/𝑥 𝑑𝑥〗=log|𝑥|+𝐶) (Since 1+𝑥^2 is always positive)
