Misc 18 - Chapter 7 Class 12 Integrals (Term 2)

Transcript

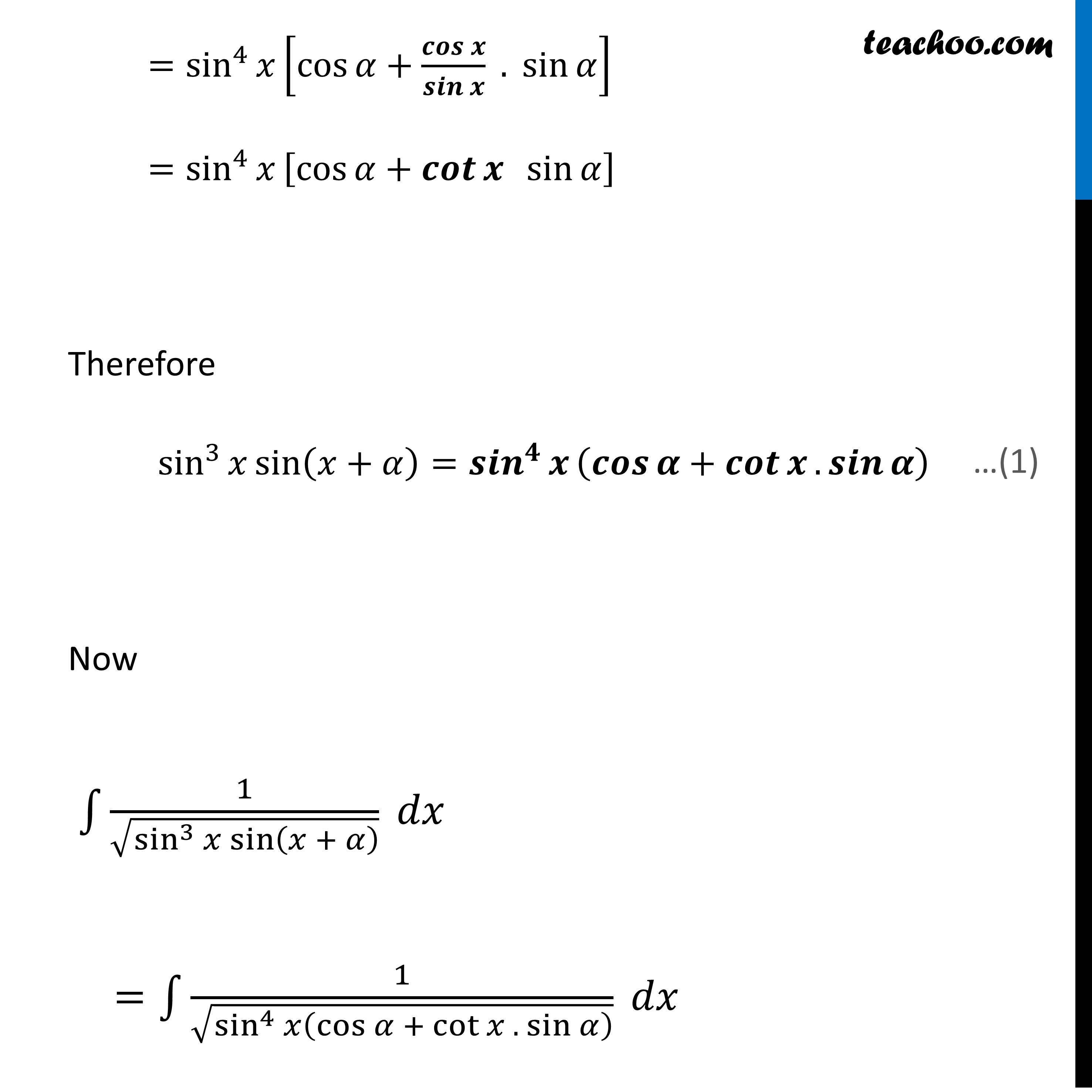
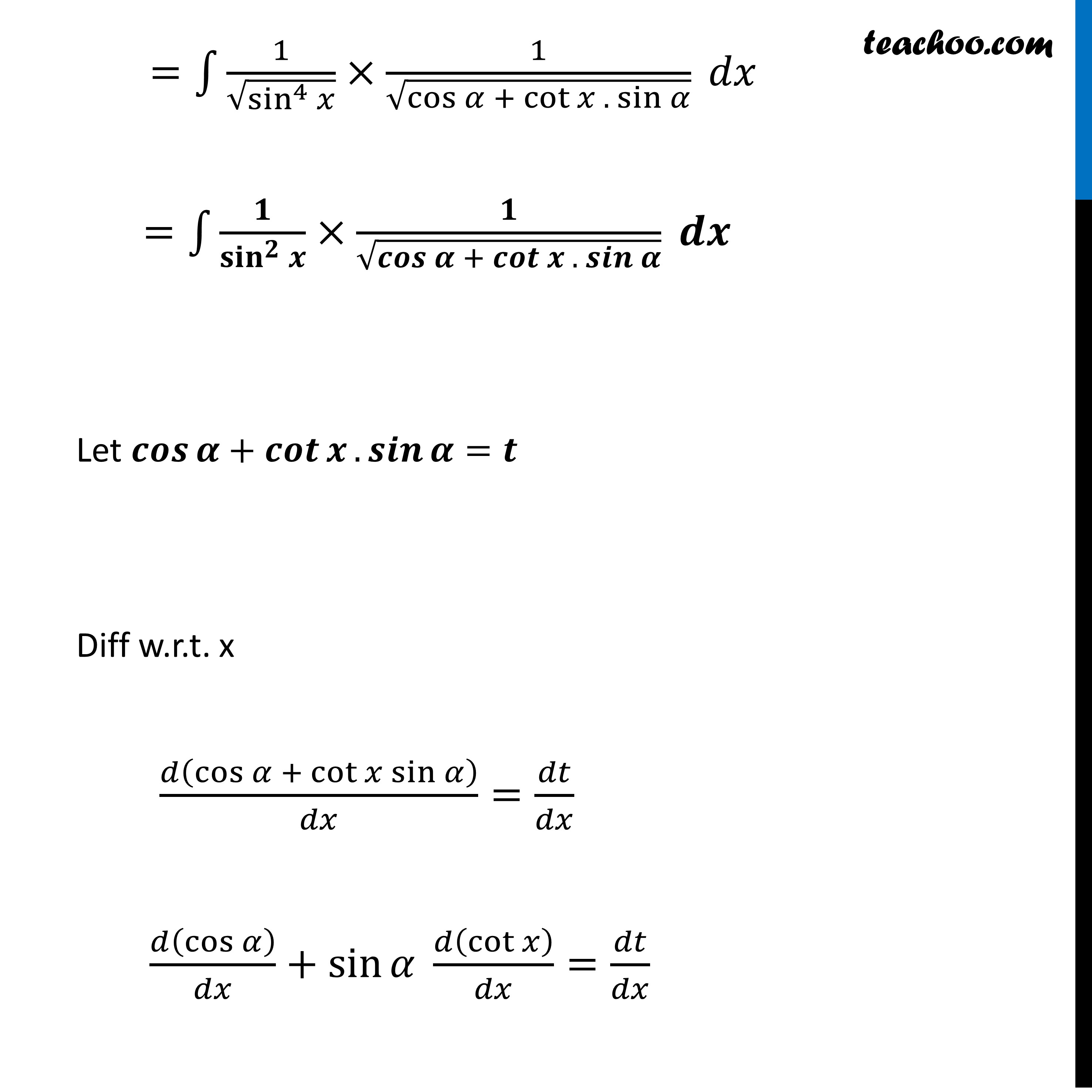
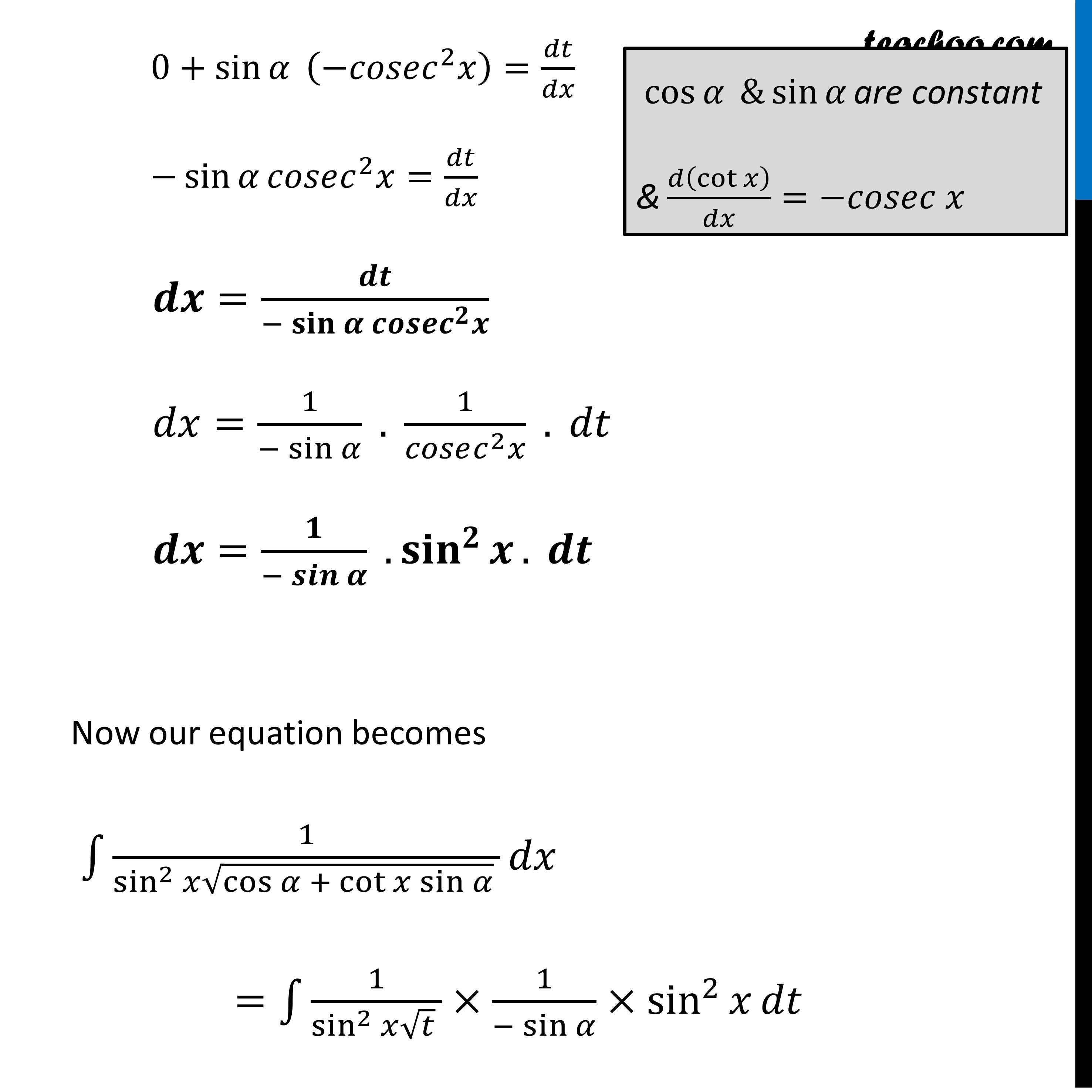
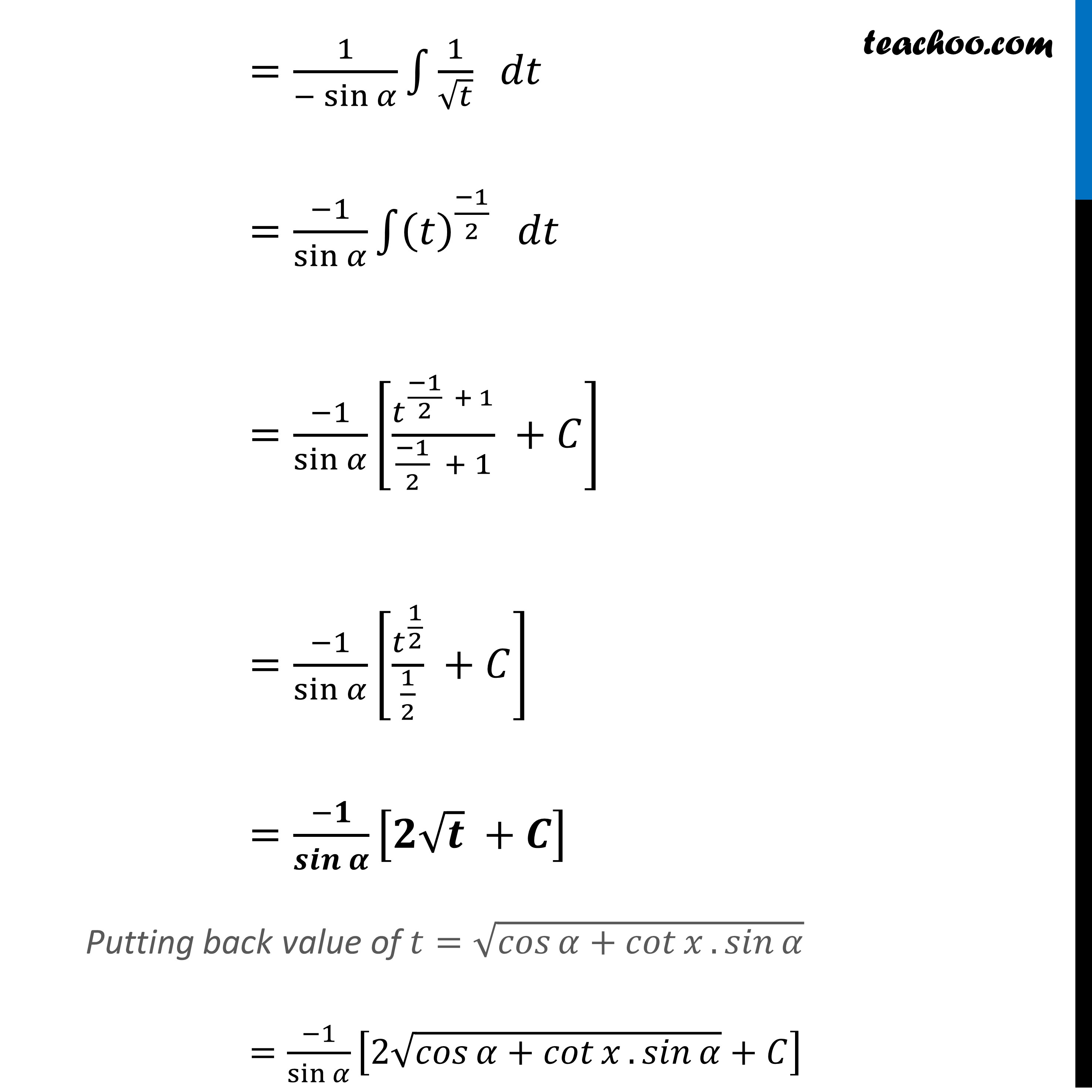
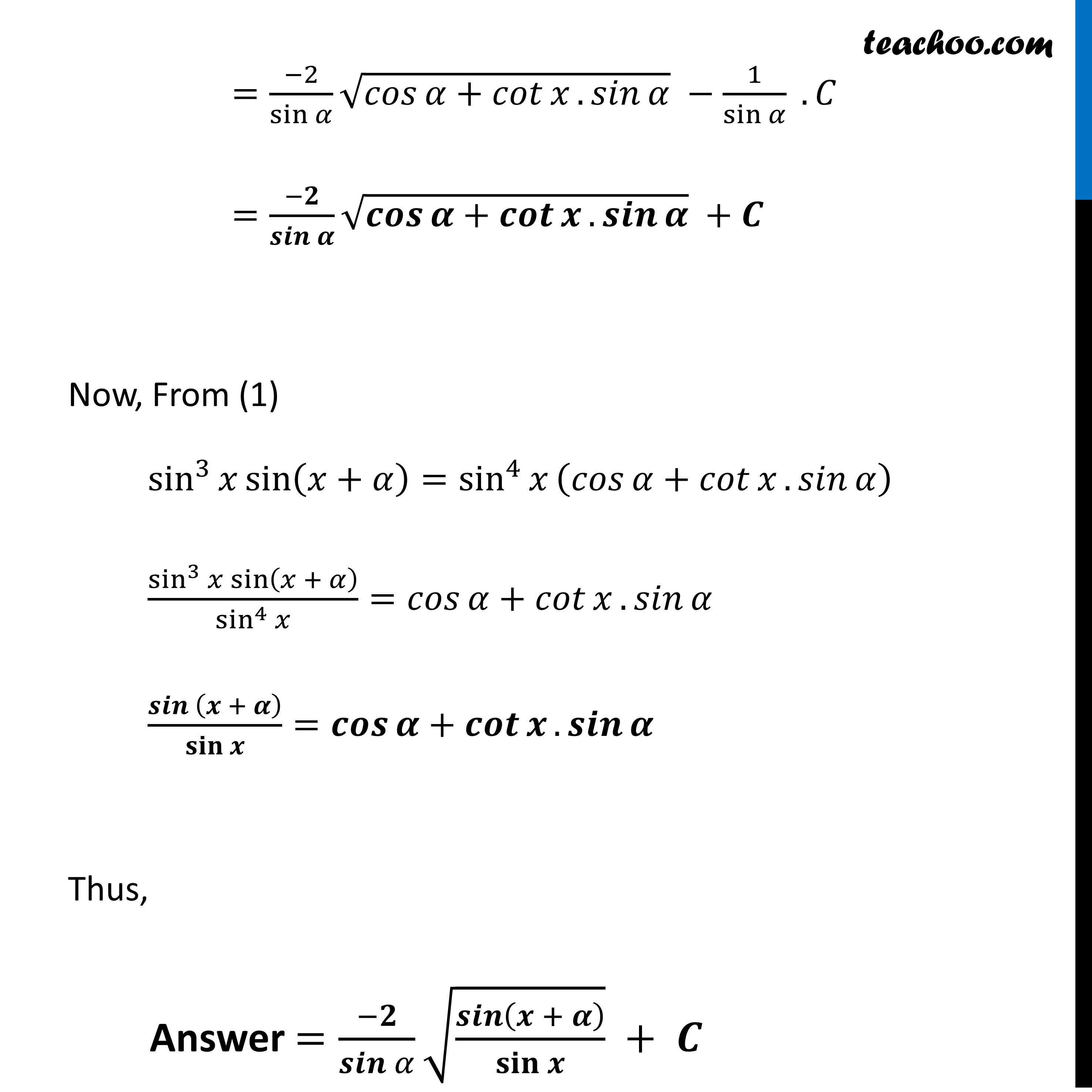
Misc 18 Integrate the function 1/√(sin^3⁡𝑥 sin⁡(𝑥 + 𝛼) ) Solving sin^3⁡𝑥 sin⁡(𝑥 + 𝛼) =sin^3⁡𝑥 [sin⁡𝑥 cos⁡𝛼+cos⁡𝑥.sin⁡𝛼 ] =〖sin^4 𝑥〗⁡cos⁡𝛼 +cos⁡𝑥.sin^3⁡𝑥 sin⁡𝛼 =〖sin^4 𝑥〗⁡cos⁡𝛼 +cos⁡𝑥.sin^3⁡𝑥 sin⁡𝛼×sin⁡𝑥/sin⁡𝑥 =sin^4⁡𝑥 [cos⁡𝛼+cos⁡𝑥 . sin⁡𝛼.1/sin⁡𝑥 ] Hence Using 𝑠𝑖𝑛⁡(𝐴+𝐵)=𝑠𝑖𝑛⁡𝐴 𝑐𝑜𝑠⁡𝐵+𝑐𝑜𝑠⁡𝐴.𝑠𝑖𝑛⁡𝐵 =sin^4⁡𝑥 [cos⁡𝛼+cos⁡𝑥/sin⁡𝑥 . sin⁡𝛼 ] =sin^4⁡𝑥 [cos⁡𝛼+cot⁡𝑥 sin⁡𝛼 ] Therefore sin^3⁡𝑥 sin⁡(𝑥+𝛼)=sin^4⁡𝑥 (cos⁡𝛼+cot⁡𝑥.sin⁡𝛼 ) Now ∫1▒1/√(sin^3⁡𝑥 sin⁡(𝑥 + 𝛼) ) 𝑑𝑥 =∫1▒1/√(sin^4⁡𝑥 (cos⁡𝛼 + cot⁡𝑥 . sin⁡𝛼 ) ) 𝑑𝑥 =∫1▒〖1/√(sin^4⁡𝑥 )×1/√(cos⁡𝛼 + cot⁡𝑥 . sin⁡𝛼 )〗 𝑑𝑥 =∫1▒〖1/sin^2⁡𝑥 ×1/√(cos⁡𝛼 + cot⁡𝑥 . sin⁡𝛼 )〗 𝑑𝑥 Let cos⁡𝛼+cot⁡𝑥. sin⁡𝛼=𝑡 Diff w.r.t. x 𝑑(cos⁡𝛼 + cot⁡𝑥 sin⁡𝛼 )/𝑑𝑥=𝑑𝑡/𝑑𝑥 𝑑(cos⁡𝛼 )/𝑑𝑥+sin⁡𝛼 𝑑(cot⁡𝑥 )/𝑑𝑥=𝑑𝑡/𝑑𝑥 =∫1▒〖1/√(sin^4⁡𝑥 )×1/√(cos⁡𝛼 + cot⁡𝑥 . sin⁡𝛼 )〗 𝑑𝑥 =∫1▒〖1/sin^2⁡𝑥 ×1/√(cos⁡𝛼 + cot⁡𝑥 . sin⁡𝛼 )〗 𝑑𝑥 Let cos⁡𝛼+cot⁡𝑥. sin⁡𝛼=𝑡 Diff w.r.t. x 𝑑(cos⁡𝛼 + cot⁡𝑥 sin⁡𝛼 )/𝑑𝑥=𝑑𝑡/𝑑𝑥 𝑑(cos⁡𝛼 )/𝑑𝑥+sin⁡𝛼 𝑑(cot⁡𝑥 )/𝑑𝑥=𝑑𝑡/𝑑𝑥 0+sin⁡𝛼 (−𝑐𝑜𝑠𝑒𝑐^2 𝑥)=𝑑𝑡/𝑑𝑥 −sin⁡𝛼 𝑐𝑜𝑠𝑒𝑐^2 𝑥=𝑑𝑡/𝑑𝑥 𝑑𝑥=𝑑𝑡/(−sin⁡𝛼 𝑐𝑜𝑠𝑒𝑐^2 𝑥) 𝑑𝑥=1/(−sin⁡𝛼 ) . 1/(𝑐𝑜𝑠𝑒𝑐^2 𝑥) . 𝑑𝑡 𝑑𝑥=1/(−sin⁡𝛼 ) . sin^2⁡𝑥. 𝑑𝑡 Now our equation becomes ∫1▒1/(sin^2⁡𝑥 √(cos⁡𝛼 + cot⁡𝑥 sin⁡𝛼 ) ) 𝑑𝑥 =∫1▒1/(sin^2⁡𝑥 √𝑡 )×1/(−sin⁡𝛼 )×sin^2⁡𝑥 𝑑𝑡 cos⁡𝛼 &sin⁡𝛼 "are constant" █(" " @"&" 𝑑(cot⁡𝑥 )/𝑑𝑥=−𝑐𝑜𝑠𝑒𝑐 𝑥) =1/(−sin⁡𝛼 ) ∫1▒1/√𝑡 𝑑𝑡 =(−1)/sin⁡𝛼 ∫1▒(𝑡)^((−1)/2) 𝑑𝑡 =(−1)/sin⁡𝛼 [𝑡^((−1)/2 + 1)/((−1)/2 + 1) +𝐶] =(−1)/sin⁡𝛼 [𝑡^(1/2)/(1/2) +𝐶] =(−𝟏)/𝒔𝒊𝒏⁡𝜶 [𝟐√𝒕 +𝑪] Putting back value of 𝑡=√(𝑐𝑜𝑠⁡𝛼+𝑐𝑜𝑡⁡𝑥. 𝑠𝑖𝑛⁡𝛼 ) =(−1)/sin⁡𝛼 [2√(𝑐𝑜𝑠⁡𝛼+𝑐𝑜𝑡⁡𝑥. 𝑠𝑖𝑛⁡𝛼 )+𝐶] =(−2)/sin⁡𝛼 √(𝑐𝑜𝑠⁡𝛼+𝑐𝑜𝑡⁡𝑥. 𝑠𝑖𝑛⁡𝛼 ) −1/sin⁡𝛼 . 𝐶 =(−2)/sin⁡𝛼 √(𝑐𝑜𝑠⁡𝛼+𝑐𝑜𝑡⁡𝑥. 𝑠𝑖𝑛⁡𝛼 ) +𝐶 Now, From (1) sin^3⁡𝑥 sin⁡(𝑥+𝛼)=sin^4⁡𝑥 (𝑐𝑜𝑠⁡𝛼+𝑐𝑜𝑡⁡𝑥. 𝑠𝑖𝑛⁡𝛼 ) (sin^3⁡𝑥 sin⁡(𝑥 + 𝛼))/sin^4⁡𝑥 =𝑐𝑜𝑠⁡𝛼+𝑐𝑜𝑡⁡𝑥. 𝑠𝑖𝑛⁡𝛼 〖𝑠𝑖𝑛 〗⁡(𝑥 + 𝛼)/sin⁡𝑥 =𝑐𝑜𝑠⁡𝛼+𝑐𝑜𝑡⁡𝑥. 𝑠𝑖𝑛⁡𝛼 Thus, Answer =(−𝟐)/𝒔𝒊𝒏⁡𝒙 √(𝒔𝒊𝒏⁡(𝒙 + 𝜶)/𝐬𝐢𝐧⁡𝒙 ) + 𝑪