Ex 6.3,29 (MCQ) - Chapter 6 Class 12 Application of Derivatives

Last updated at Dec. 16, 2024 by

  Ex 6.3, 29 (MCQ - The max value of [x(x - 1) + 1]^1/3 is (a) (1/3)^1/3 - Ex 6.3

part 2 - Ex 6.3,29 (MCQ) - Ex 6.3 - Serial order wise - Chapter 6 Class 12 Application of Derivatives
part 3 - Ex 6.3,29 (MCQ) - Ex 6.3 - Serial order wise - Chapter 6 Class 12 Application of Derivatives

Remove Ads

Transcript

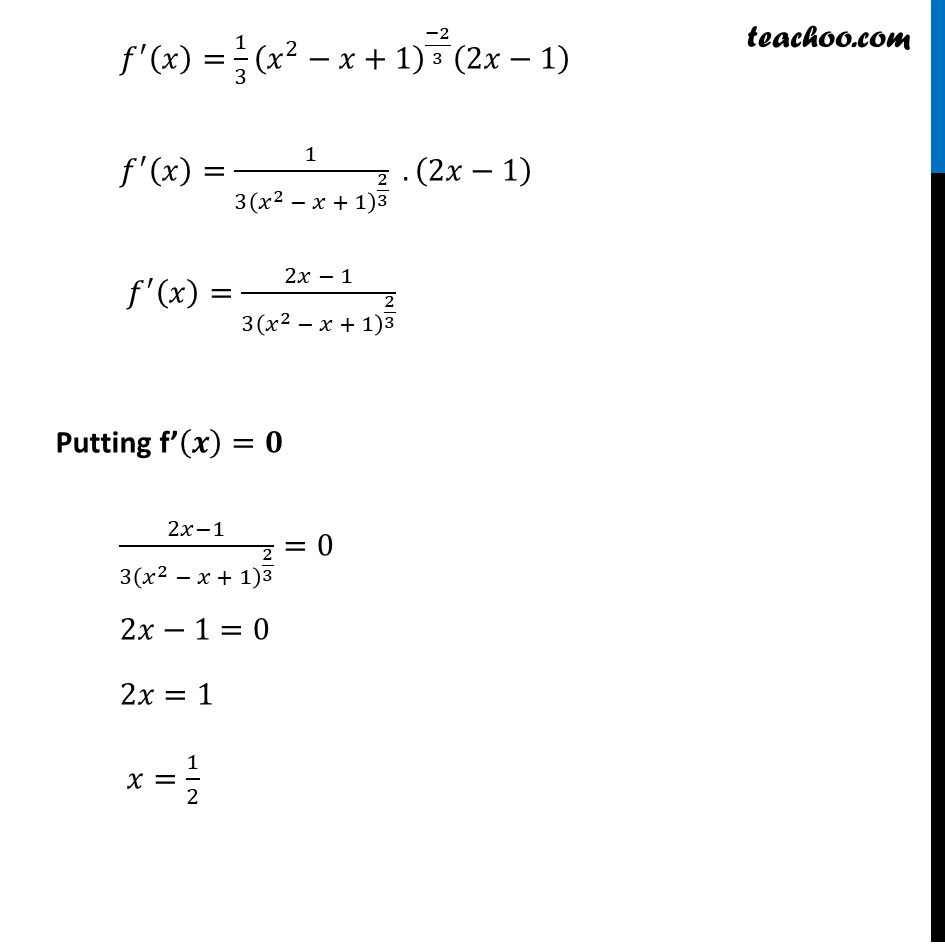
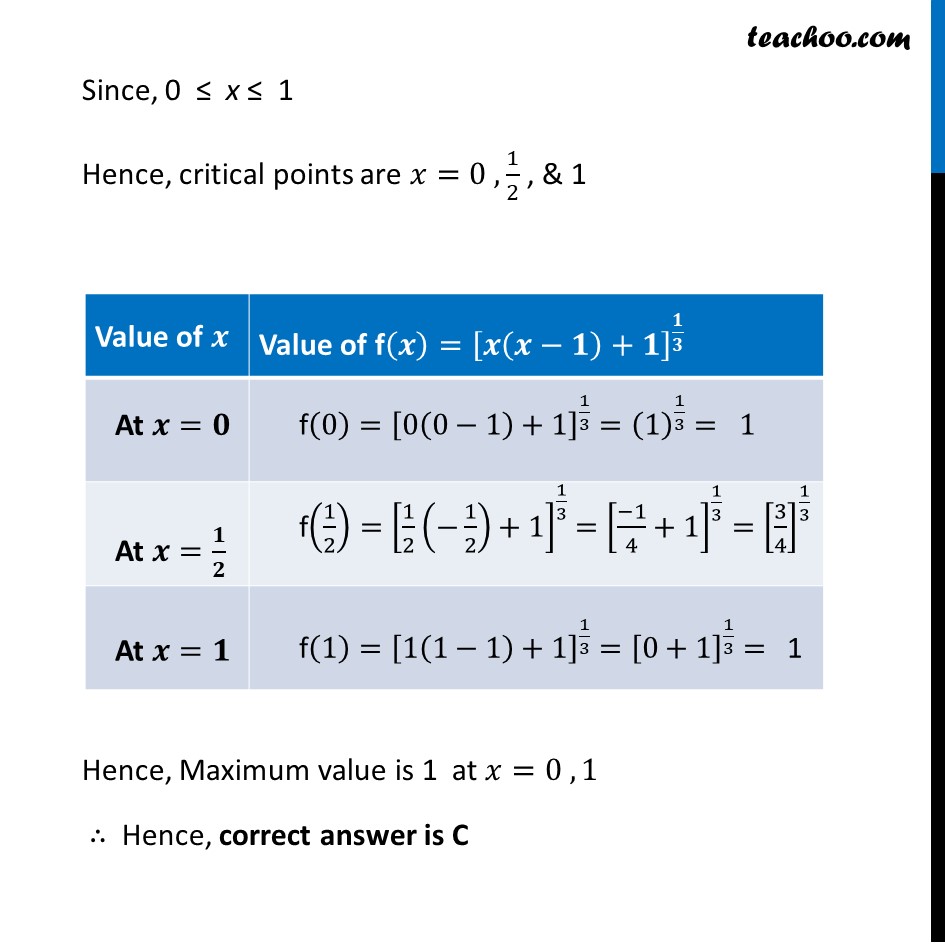
Ex 6.3, 29 The maximum value of 〖[𝑥(𝑥−1)+1]〗^(1/3) 0 ≤ x ≤ 1 is (A) (1/3)^(1/3) (B) 1/2 (C) 1 (D) 0 𝑓^′ (𝑥)=1/3 (𝑥^2−𝑥+1)^((−2)/3) (2𝑥−1) 𝑓^′ (𝑥)=1/(3(𝑥^2 − 𝑥 + 1)^(2/3) ) .(2𝑥−1) 𝑓^′ (𝑥)=(2𝑥 − 1)/(3(𝑥^2 − 𝑥 + 1)^(2/3) ) Putting f’(𝒙)=𝟎 (2𝑥−1)/(3(𝑥^2 − 𝑥 + 1)^(2/3) )=0 2𝑥−1=0 2𝑥=1 𝑥=1/2 Since, 0 ≤ x ≤ 1 Hence, critical points are 𝑥=0 ,1/2 , & 1 Hence, Maximum value is 1 at 𝑥=0 , 1 ∴ Hence, correct answer is C

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo