Ex 6.3,10 - Chapter 6 Class 12 Application of Derivatives

Last updated at Dec. 16, 2024 by

  Ex 6.3, 10 - Find max value of 2x3 - 24 x + 107 in [1, 3] - Ex 6.3

part 2 - Ex 6.3,10 - Ex 6.3 - Serial order wise - Chapter 6 Class 12 Application of Derivatives
part 3 - Ex 6.3,10 - Ex 6.3 - Serial order wise - Chapter 6 Class 12 Application of Derivatives
part 4 - Ex 6.3,10 - Ex 6.3 - Serial order wise - Chapter 6 Class 12 Application of Derivatives

Remove Ads

Transcript

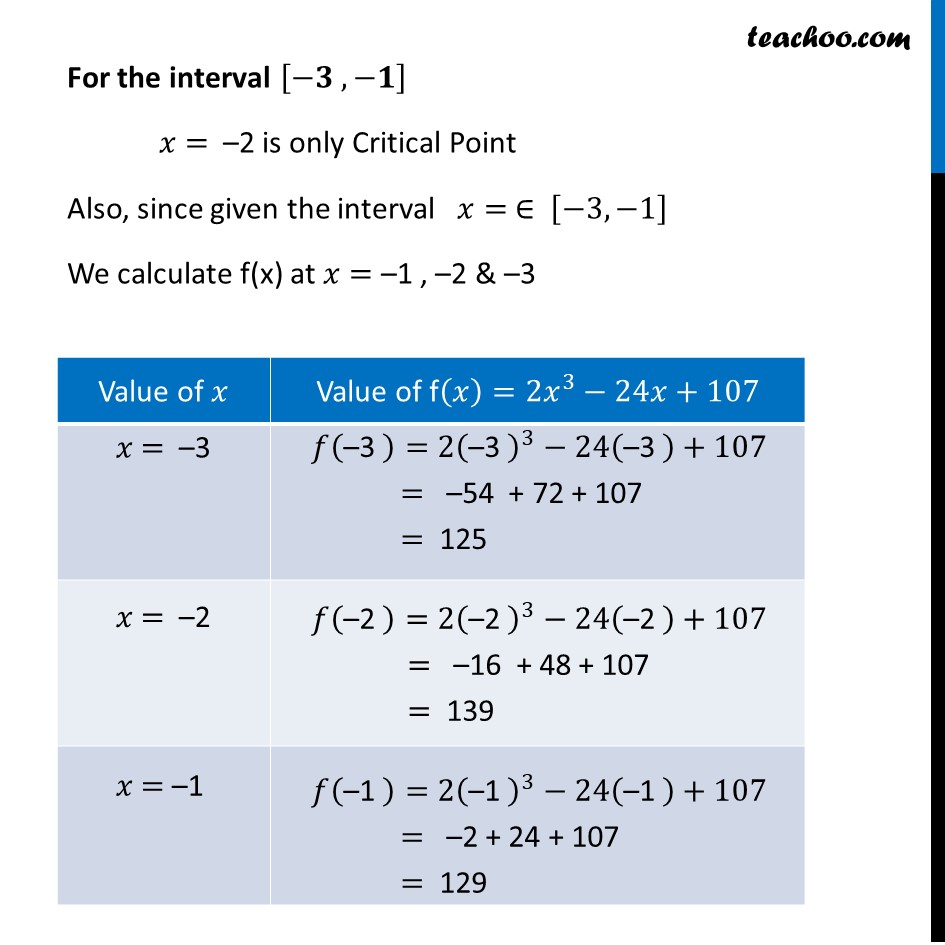
Ex 6.3, 10 Find the maximum value of 2𝑥3 – 24𝑥 + 107 in the interval [1, 3]. Find the maximum value of the same function in [–3, –1]. Let f(𝑥)=2𝑥^3−24𝑥+107 Finding f’(𝑥) f’(𝑥)=𝑑(2𝑥^3 − 24𝑥 + 107)/𝑑𝑥 = 2 × 3𝑥^2−24 = 6𝑥^2−24 = 6 (𝑥^2−4" " ) Putting f(𝑥)=0 6 (𝑥^2−4" " )=0 𝑥^2−4" = 0 " 𝑥^2=4 𝑥=±√4 𝑥=±2 Thus, 𝑥=2 , – 2 Since x is in interval [1 , 3] 𝑥 = 2 is only Critical point Also, since given the interval 𝑥= ∈ [1 , 3] We calculate f(x) at 𝑥= 1 , 2 & 3 Hence maximum value of f(𝒙)=𝟖𝟗 at 𝒙 = 3 in the interval [1 , 3] For the interval [−𝟑 , −𝟏] 𝑥 = –2 is only Critical Point Also, since given the interval 𝑥= ∈ [−3,−1] We calculate f(x) at 𝑥= –1 , –2 & –3 Hence maximum value of f(𝒙)=𝟏𝟑𝟗 at 𝒙 = –2 in the interval [−3,−1]

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo