Ex 6.3,7 - Chapter 6 Class 12 Application of Derivatives

Last updated at Dec. 16, 2024 by

  Ex 6.3, 7 - Find both max, min value of 3x4 - 8x3 + 12x2 - Ex 6.3

part 2 - Ex 6.3,7 - Ex 6.3 - Serial order wise - Chapter 6 Class 12 Application of Derivatives
part 3 - Ex 6.3,7 - Ex 6.3 - Serial order wise - Chapter 6 Class 12 Application of Derivatives
part 4 - Ex 6.3,7 - Ex 6.3 - Serial order wise - Chapter 6 Class 12 Application of Derivatives

Remove Ads

Transcript

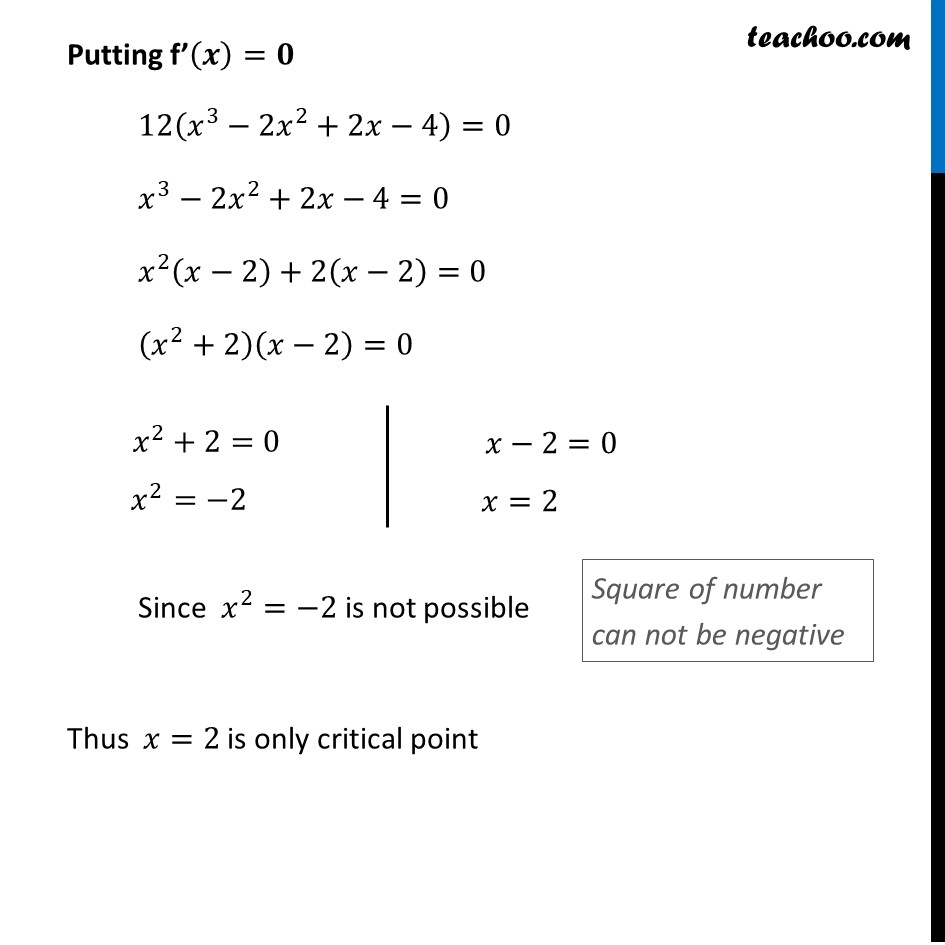
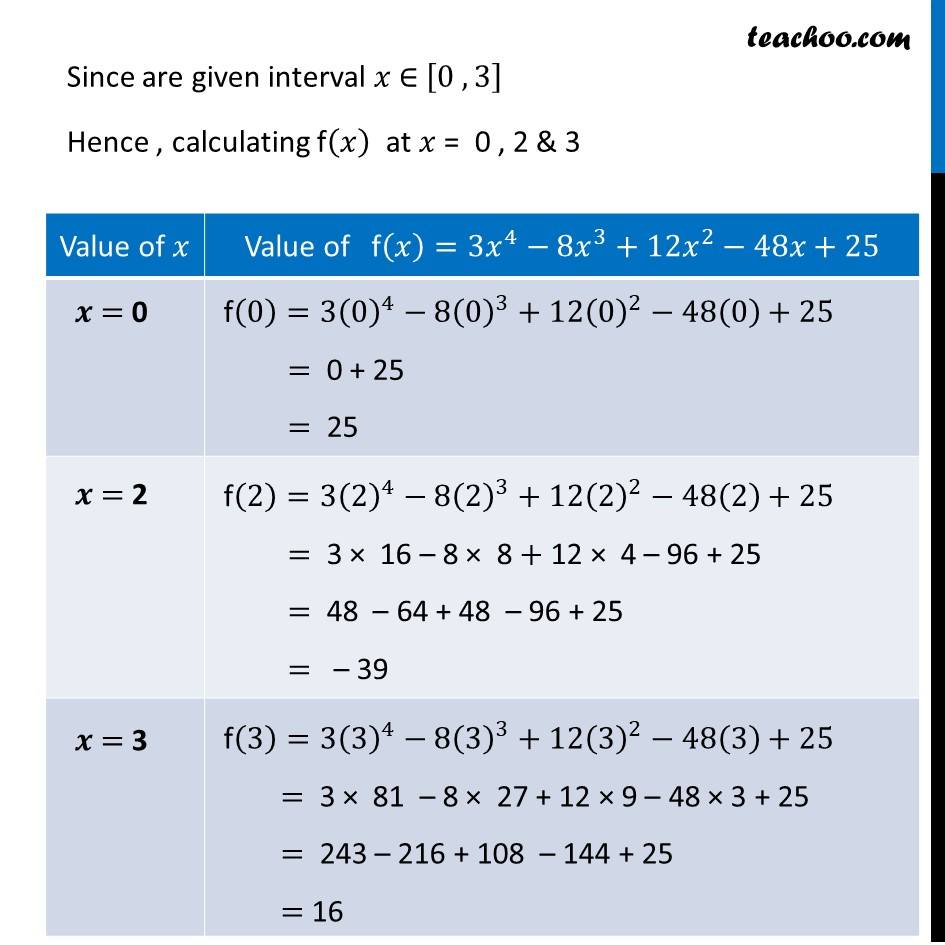
Ex 6.3, 7 Find both the maximum value and the minimum value of 3𝑥4 – 8𝑥3 + 12𝑥2 – 48𝑥 + 25 on the interval [0, 3].Let f(x) = 3𝑥4 – 8𝑥3 + 12𝑥2 – 48𝑥 + 25, where 𝑥 ∈ [0, 3] Finding f’(𝒙) f’(𝑥)=𝑑(3𝑥^4 − 8𝑥^3 + 12𝑥^2 − 48𝑥 + 25)/𝑑𝑥 f’(𝑥)=3 ×4𝑥^3−8 ×3𝑥^2+12 ×2𝑥−48+0 f’(𝑥)=12𝑥^3−24𝑥^2+24𝑥−48 f’(𝑥)=12(𝑥^3−2𝑥^2+2𝑥−4) Putting f’(𝒙)=𝟎 12(𝑥^3−2𝑥^2+2𝑥−4)=0 𝑥^3−2𝑥^2+2𝑥−4=0 𝑥^2 (𝑥−2)+2(𝑥−2)=0 (𝑥^2+2)(𝑥−2)=0 Since 𝑥^2=−2 is not possible Thus 𝑥=2 is only critical point Since are given interval 𝑥 ∈ [0 , 3] Hence , calculating f(𝑥) at 𝑥 = 0 , 2 & 3 Hence, Minimum value of f(𝑥) is –39 at 𝒙 = 2 Maximum value of f(𝑥) is 25 at 𝒙 = 0

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo