Ex 6.3, 2 (iv) - Chapter 6 Class 12 Application of Derivatives

Last updated at April 16, 2024 by Teachoo

Next: Ex 6.3, 2 (v) Important → Go Ad-free

Transcript

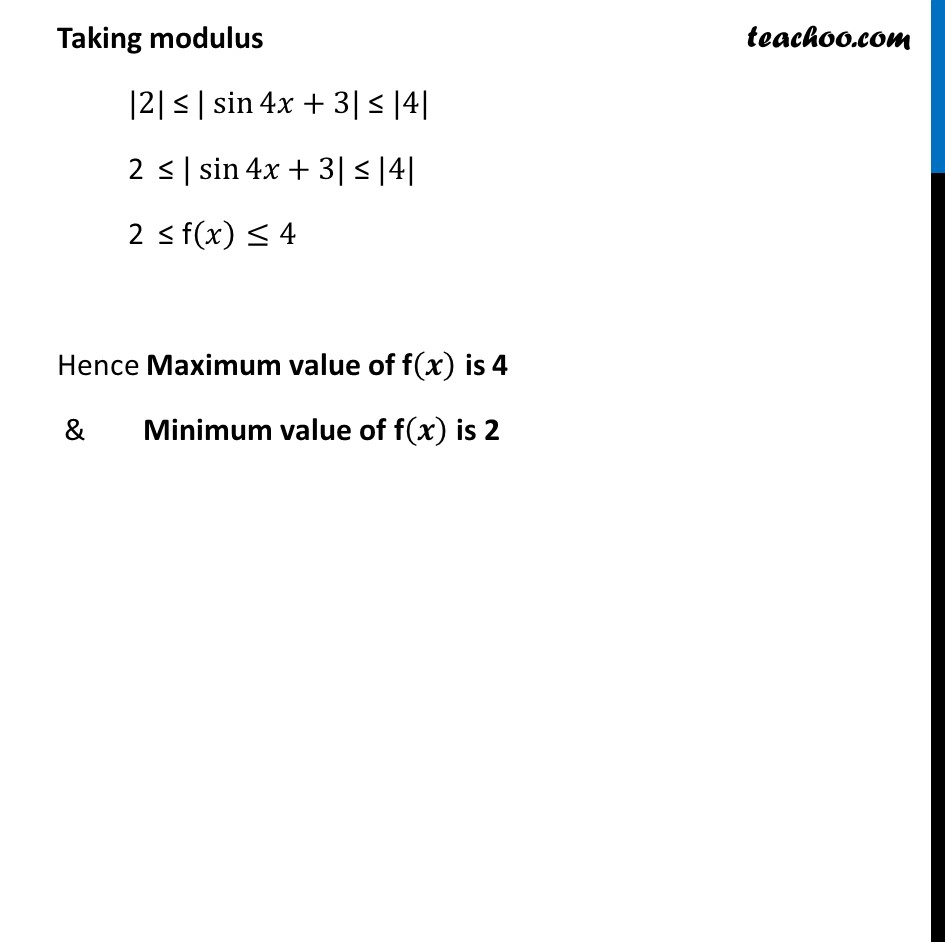
Ex 6.3, 2 Find the maximum and minimum values, if any, of the following functions given by (iv) 𝑓 (𝑥)=|sin⁡4𝑥+3|𝑓 (𝑥)=| sin⁡4𝑥+3| We know that –1 ≤ sin θ ≤ 1 So, –1 ≤ sin 4𝑥 ≤ 1 Adding 3 both sides –1 + 3 ≤ sin 4𝑥 + 3 ≤ 1 + 3 2 ≤ sin 4𝑥 +3 ≤ 4 Taking modulus |2| ≤ | sin⁡4𝑥+3| ≤ |4| 2 ≤ | sin⁡4𝑥+3| ≤ |4| 2 ≤ f(𝑥)≤4 Hence Maximum value of f(𝒙) is 4 & Minimum value of f(𝒙) is 2

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular