Ex 6.3,9 - Chapter 6 Class 12 Application of Derivatives

Last updated at August 17, 2026 by

  Ex 6.3, 9 - What is the maximum value of sin x + cos x? - Ex 6.3 - Ex 6.3

part 2 - Ex 6.3,9 - Ex 6.3 - Serial order wise - Chapter 6 Class 12 Application of Derivatives
part 3 - Ex 6.3,9 - Ex 6.3 - Serial order wise - Chapter 6 Class 12 Application of Derivatives

Remove Ads Take short quiz All Quiz and Worksheets
Teachoo · Class 12 Explore Class 12

Transcript

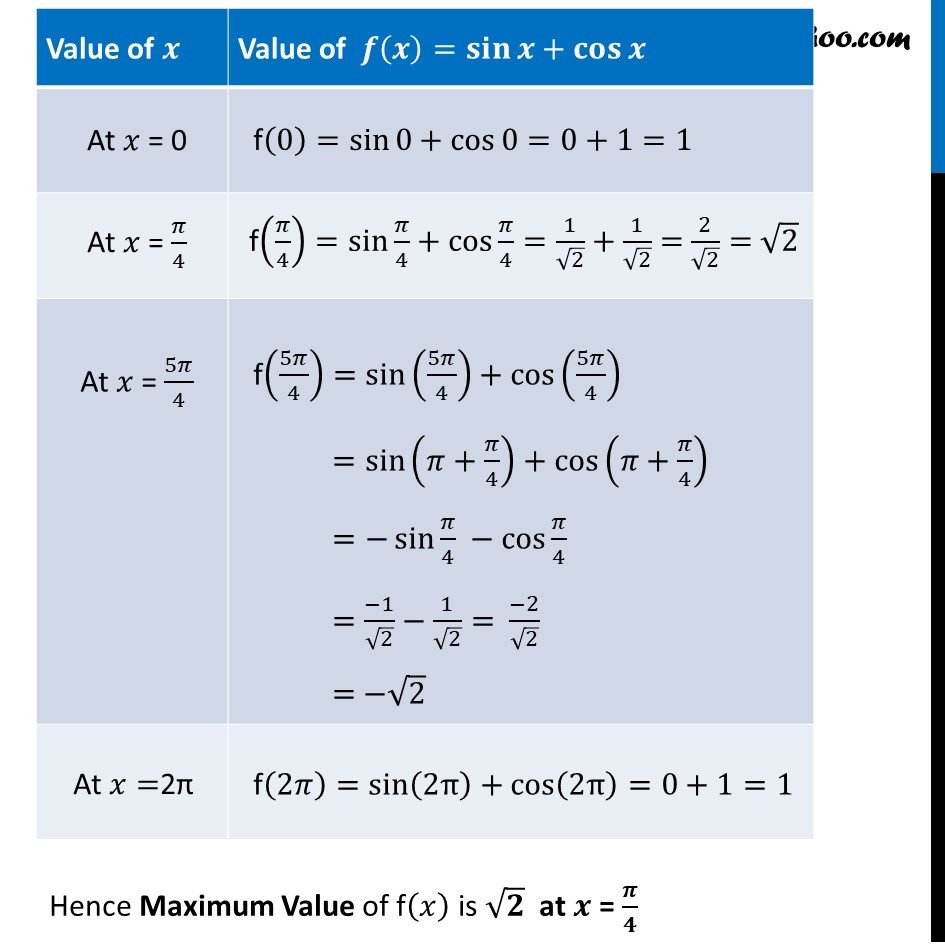
Ex 6.3, 9 What is the maximum value of the function sin⁡𝑥+cos⁡𝑥? Let f(𝑥)=sin⁡𝑥+cos⁡𝑥 Consider the interval 𝑥 ∈ [0 , 2𝜋] Finding f’(𝒙) f’(𝑥)=𝑑(sin⁡𝑥 + cos⁡𝑥 )/𝑑𝑥 f’(𝑥)=cos⁡𝑥−sin⁡𝑥 Putting f’(𝒙)=𝟎 cos⁡𝑥−sin⁡𝑥=0 cos⁡𝑥=sin⁡𝑥 1 =sin⁡𝑥/cos⁡𝑥 1= tan⁡𝑥 tan 𝑥=1 Since 𝑥 ∈ [0 , 2𝜋] tan 𝑥=1 at 𝑥=𝜋/4 , 𝑥=5𝜋/4 in the interval [0 , 2𝜋] We have given the interval 𝑥 ∈ [0 , 2𝜋] Hence Calculating f(𝑥) at 𝑥=0 ,𝜋/4 , 5𝜋/4 & 2𝜋 Hence Maximum Value of f(𝑥) is √𝟐 at 𝒙 = 𝝅/𝟒

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh is an IIT Kanpur graduate and has been teaching for 16+ years. At Teachoo, he breaks down Maths, Science and Computer Science into simple steps so students understand concepts deeply and score with confidence.

Many students prefer Teachoo Black for a smooth, ad-free learning experience.