Ex 6.3, 1 (iv) - Chapter 6 Class 12 Application of Derivatives

Last updated at Dec. 16, 2024 by

Slide22.JPG

Slide23.JPG
Slide24.JPG
Slide25.JPG

Share on WhatsApp

Transcript

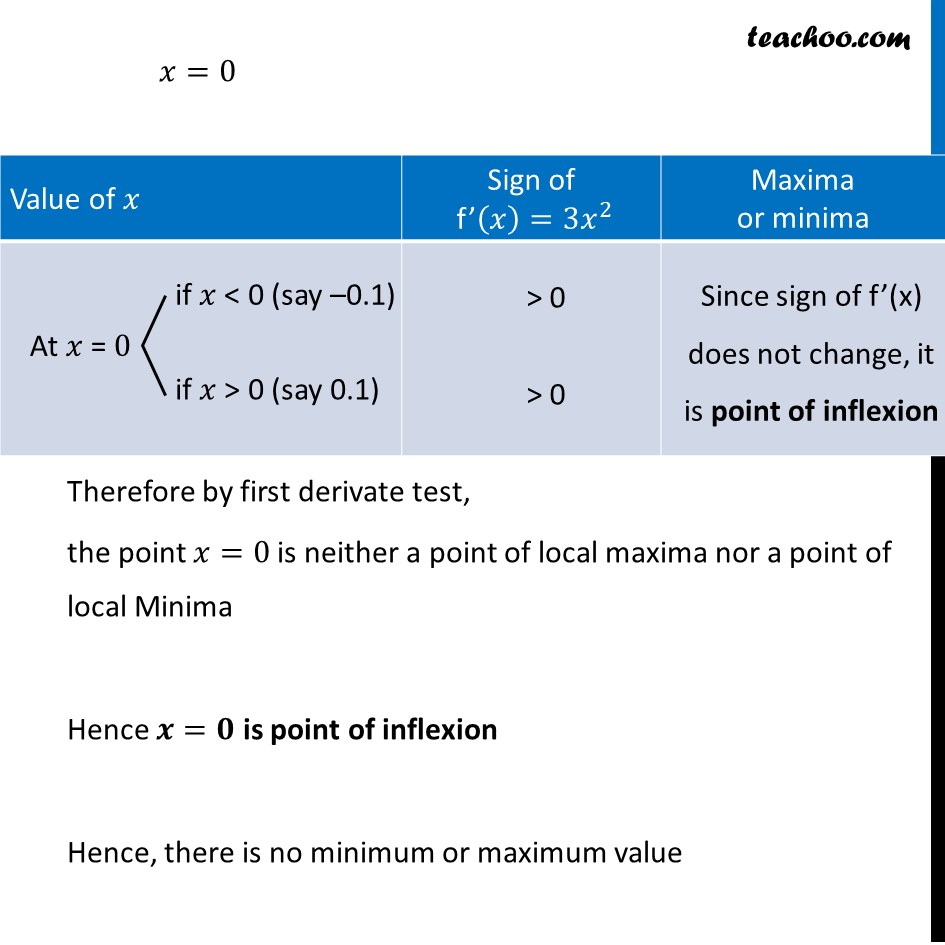
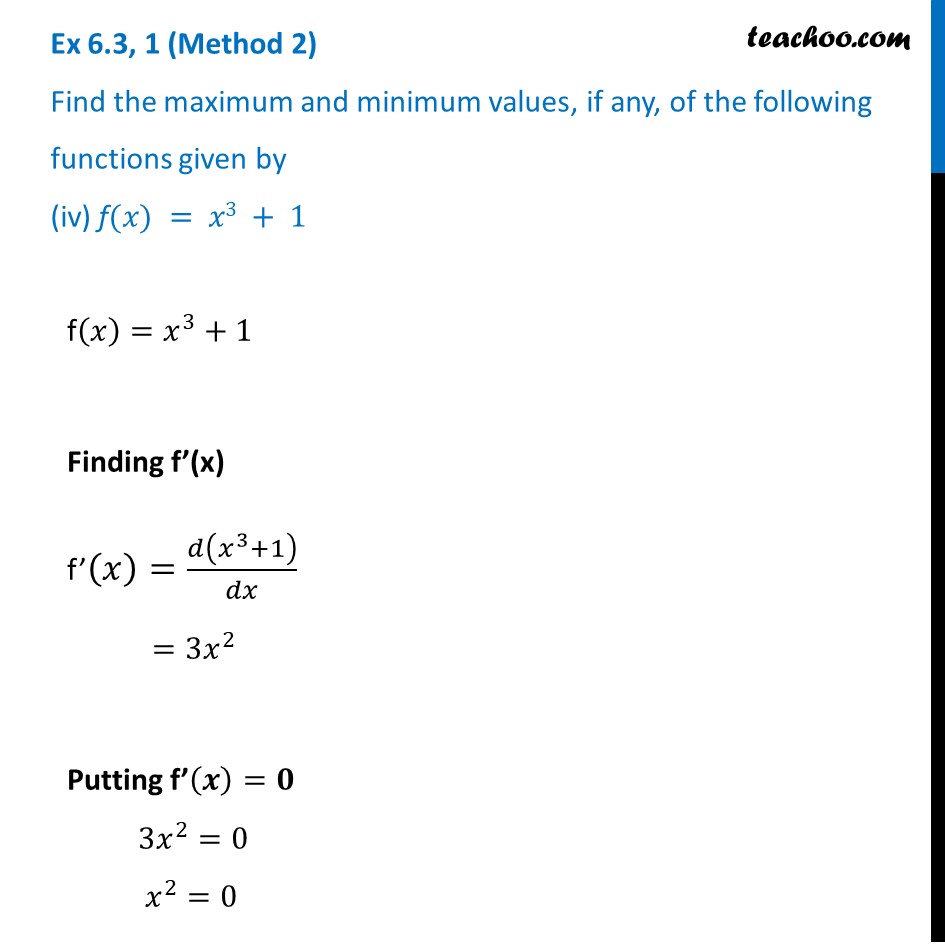
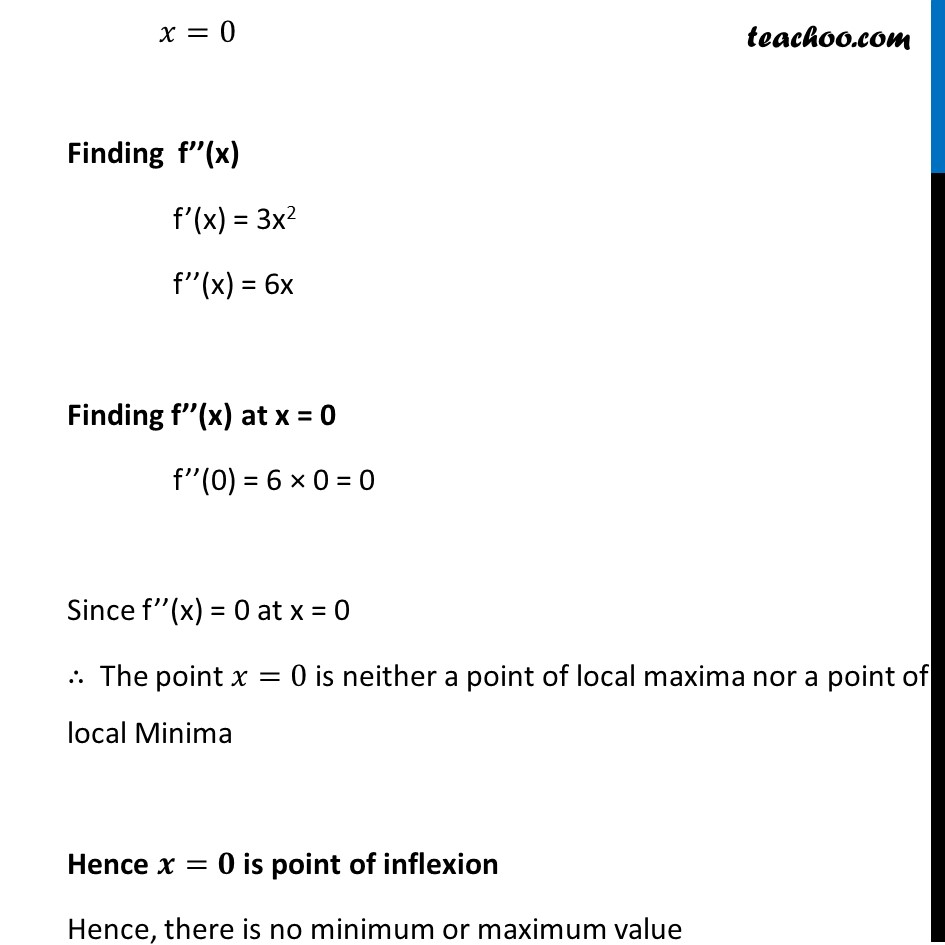
Ex 6.3,1 (Method 1) Find the maximum and minimum values, if any, of the following functions given by (iv) f(𝑥) = 𝑥3 + 1f(𝑥)=𝑥^3+1 Finding f’(x) f’(𝑥)=𝑑(𝑥^3 + 1)/𝑑𝑥 =3𝑥^2 Putting f’(𝒙)=𝟎 3𝑥^2=0 𝑥^2=0 𝑥=0 Therefore by first derivate test, the point 𝑥=0 is neither a point of local maxima nor a point of local Minima Hence 𝒙=𝟎 is point of inflexion Hence, there is no minimum or maximum value Ex 6.3, 1 (Method 2) Find the maximum and minimum values, if any, of the following functions given by (iv) f(𝑥) = 𝑥3 + 1f(𝑥)=𝑥^3+1 Finding f’(x) f’(𝑥)=𝑑(𝑥^3+1)/𝑑𝑥 =3𝑥^2 Putting f’(𝒙)=𝟎 3𝑥^2=0 𝑥^2=0 𝑥=0 Finding f’’(x) f’(x) = 3x2 f’’(x) = 6x Finding f’’(x) at x = 0 f’’(0) = 6 × 0 = 0 Since f’’(x) = 0 at x = 0 ∴ The point 𝑥=0 is neither a point of local maxima nor a point of local Minima Hence 𝒙=𝟎 is point of inflexion Hence, there is no minimum or maximum value

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo