Ex 6.5,23 - Chapter 6 Class 12 Application of Derivatives

Transcript

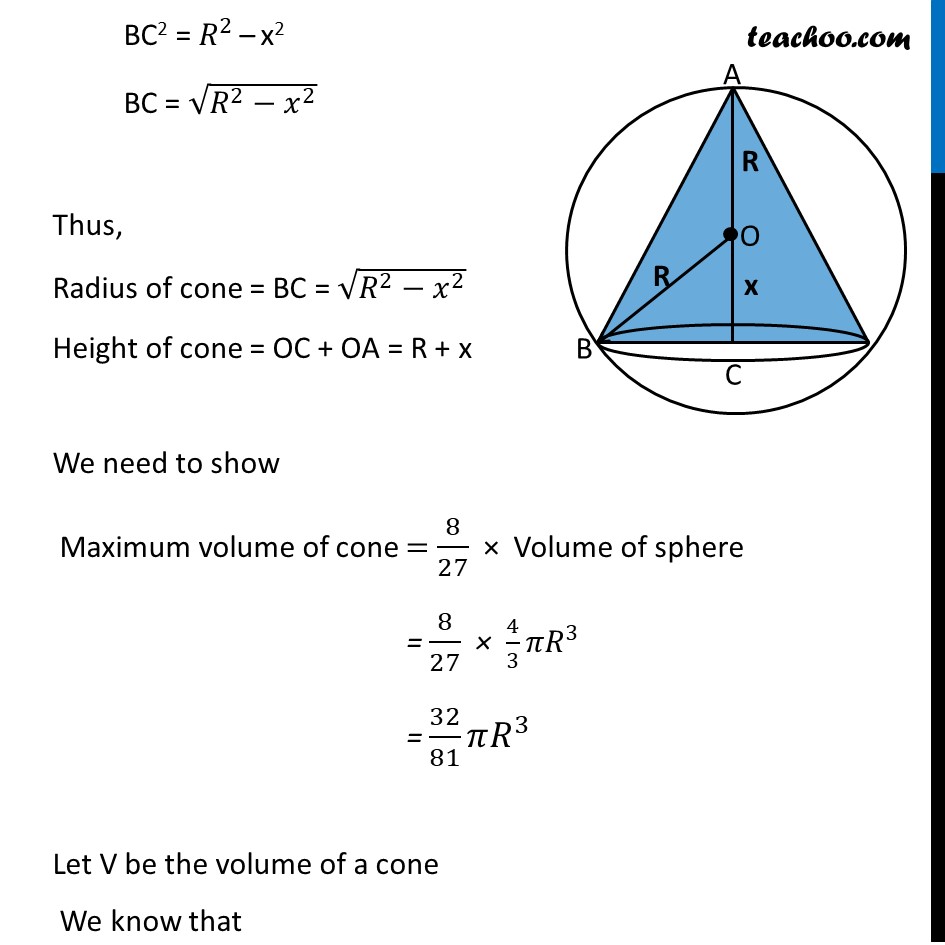
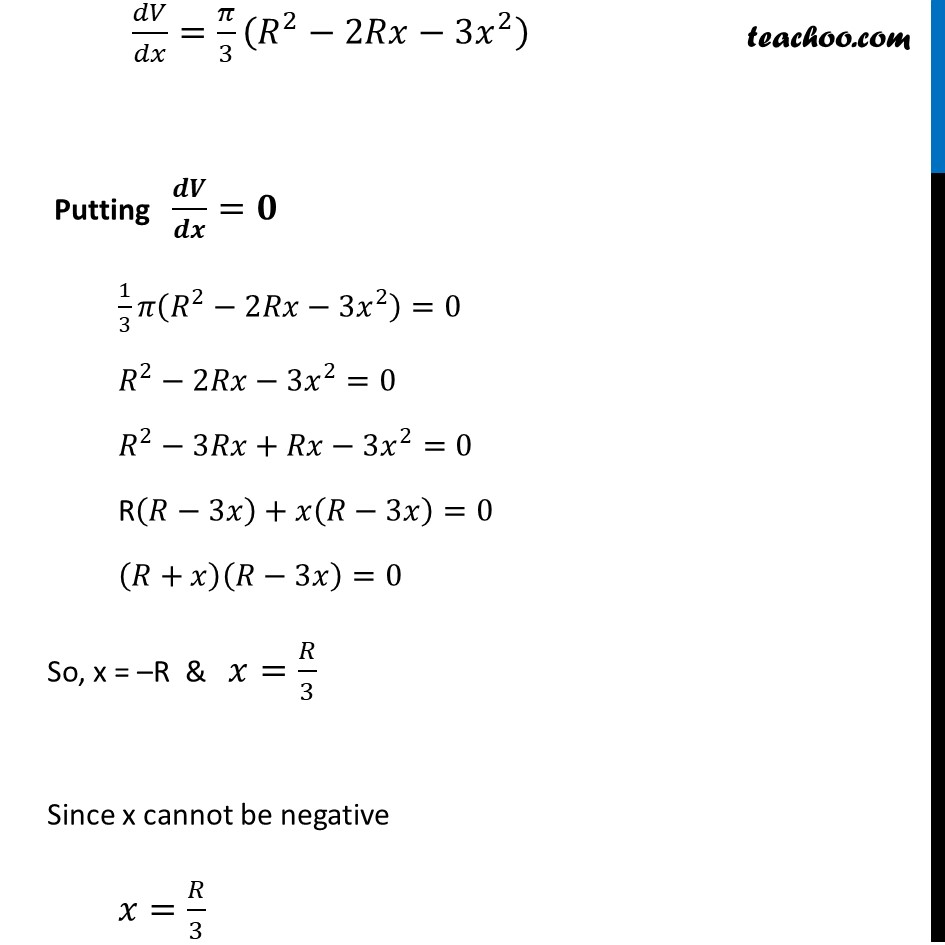
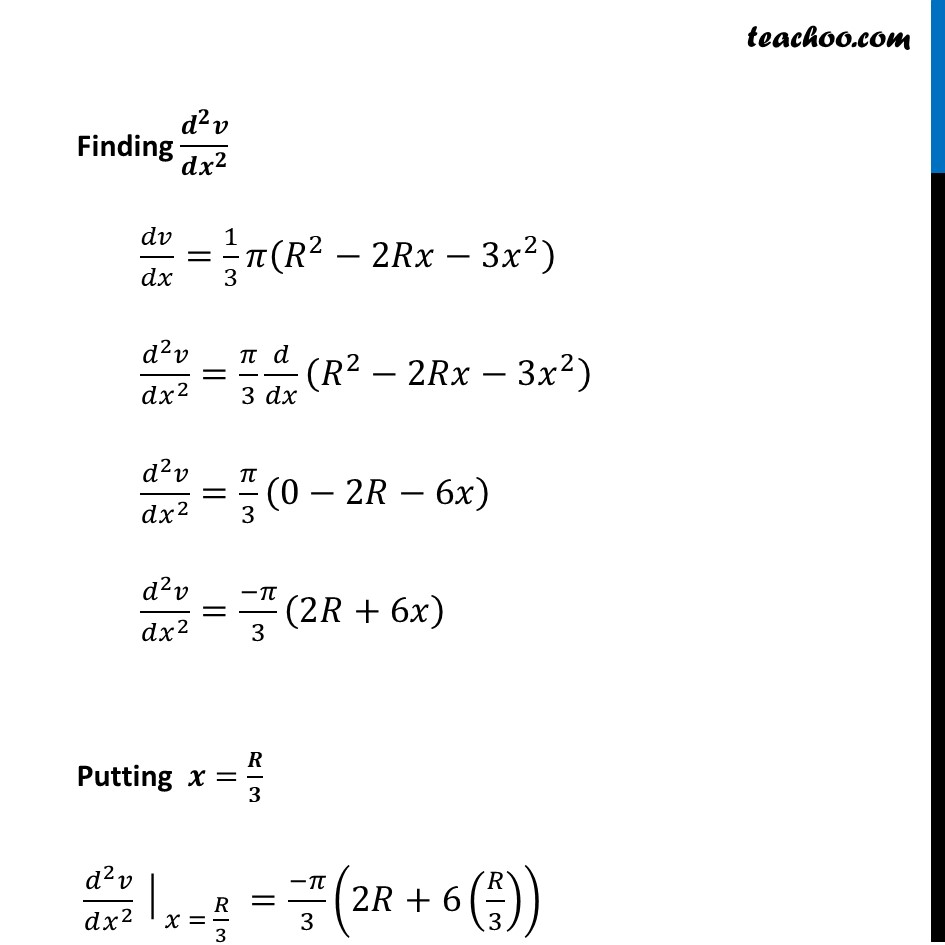
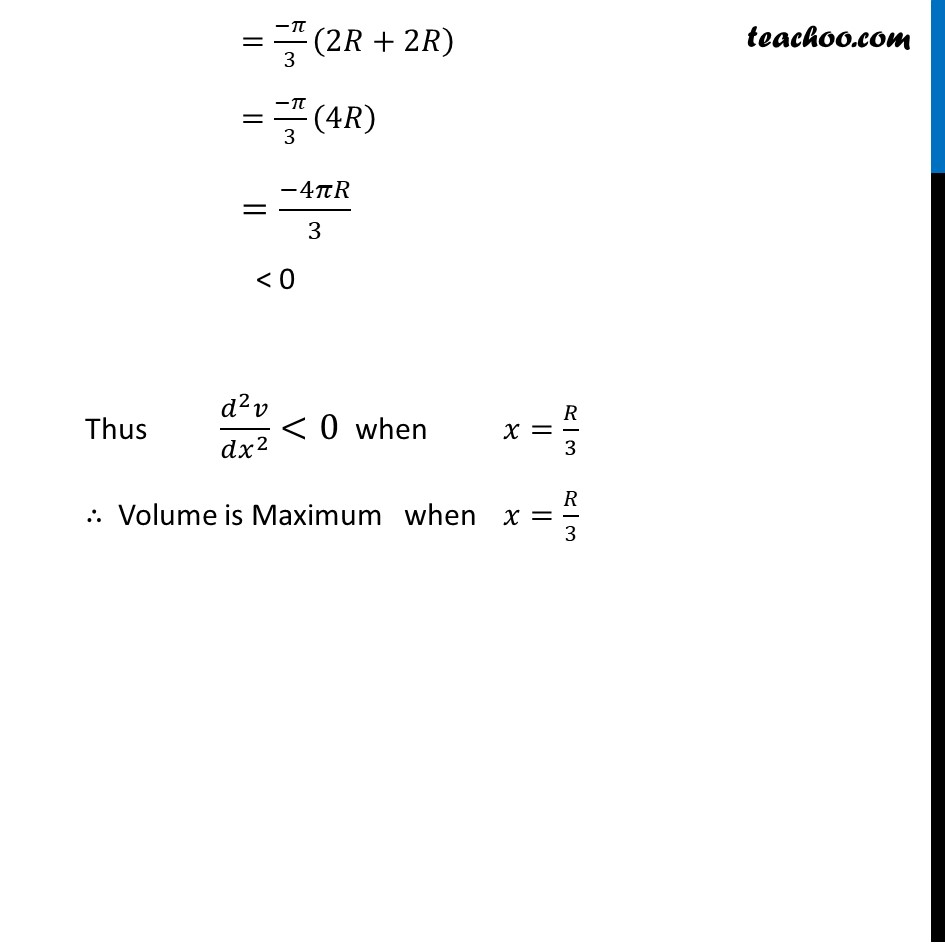
Ex 6.5,23 Prove that the volume of the largest cone that can be inscribed in a sphere of radius R is 8﷮27﷯ of the volume of the sphere. Cone of largest volume inscribed in the sphere of radius R Let OC = x Radius of cone = BC Height of cone = h = OC + OA Finding OC Δ BOC is a right angled triangle Using Pythagoras theorem in ∆BOC 𝑂𝐵﷮2﷯= 𝐵𝐶﷮2﷯+ 𝑂𝐶﷮2﷯ R2 = 𝐵𝐶﷮2﷯+ 𝑥﷮2﷯ BC2 = 𝑅﷮2﷯ – x2 BC = ﷮ 𝑅﷮2﷯− 𝑥﷮2﷯﷯ Thus, Radius of cone = BC = ﷮ 𝑅﷮2﷯− 𝑥﷮2﷯﷯ Height of cone = OC + OA = R + x We need to show Maximum volume of cone = 8﷮27﷯ × Volume of sphere = 8﷮27﷯ × 4﷮3﷯𝜋 𝑟﷮3﷯ = 32﷮81﷯𝜋 𝑟﷮3﷯ Let V be the volume of a cone We know that Volume of a cone = 1﷮3﷯𝜋 𝑟𝑎𝑑𝑖𝑢𝑠 ﷯﷮2﷯ ℎ𝑒𝑖𝑔ℎ𝑡 ﷯ V = 1﷮3﷯𝜋 ﷮ 𝑅﷮2﷯− 𝑥﷮2﷯﷯﷯﷮2﷯ 𝑅+𝑥﷯ V = 1﷮3﷯𝜋 𝑅﷮2﷯− 𝑥﷮2﷯﷯ 𝑅+𝑥﷯ V = 1﷮3﷯𝜋 𝑅﷮2﷯ 𝑅+𝑥﷯− 𝑥﷮2﷯ 𝑅+𝑥﷯﷯ V = 1﷮3﷯𝜋 𝑅﷮3 ﷯+ 𝑅﷮2﷯𝑥− 𝑥﷮2﷯𝑅− 𝑥﷮3﷯﷯ Diff w.r.t 𝑥 𝑑𝑉﷮𝑑𝑥﷯= 𝑑﷮𝑑𝑥﷯ 1﷮3﷯𝜋 𝑅﷮3 ﷯+ 𝑅﷮2﷯𝑥− 𝑥﷮2﷯𝑅− 𝑥﷮3﷯﷯﷯ 𝑑𝑉﷮𝑑𝑥﷯= 𝜋﷮3﷯ 𝑑﷮𝑑𝑥﷯ 𝑅﷮3 ﷯+ 𝑅﷮2﷯𝑥− 𝑥﷮2﷯𝑅− 𝑥﷮3﷯﷯﷯ 𝑑𝑉﷮𝑑𝑥﷯= 𝜋﷮3﷯ 0+ 𝑅﷮2﷯.1−𝑅 ×2𝑥−3 𝑥﷮2﷯﷯ 𝑑𝑉﷮𝑑𝑥﷯= 𝜋﷮3﷯ 𝑅﷮2﷯−2𝑅𝑥−3 𝑥﷮2﷯﷯ Putting 𝑑𝑉﷮𝑑𝑥﷯=0 1﷮3﷯𝜋 𝑅﷮2﷯−2𝑅𝑥−3 𝑥﷮2﷯﷯=0 𝑅﷮2﷯−2𝑅𝑥−3 𝑥﷮2﷯=0 𝑅﷮2﷯−3𝑅𝑥+𝑅𝑥−3 𝑥﷮2﷯=0 R 𝑅−3𝑥﷯+𝑥 𝑅−3𝑥﷯=0 𝑅+𝑥﷯ 𝑅−3𝑥﷯=0 So, x = –R & 𝑥= 𝑅﷮3﷯ Since x cannot be negative 𝑥= 𝑅﷮3﷯ Finding 𝑑﷮2﷯𝑣﷮𝑑 𝑥﷮2﷯﷯ 𝑑𝑣﷮𝑑𝑥﷯= 1﷮3﷯𝜋 𝑅﷮2﷯−2𝑅𝑥−3 𝑥﷮2﷯﷯ 𝑑﷮2﷯𝑣﷮𝑑 𝑥﷮2﷯﷯= 𝜋﷮3﷯ 𝑑﷮𝑑𝑥﷯ 𝑅﷮2﷯−2𝑅𝑥−3 𝑥﷮2﷯﷯ 𝑑﷮2﷯𝑣﷮𝑑 𝑥﷮2﷯﷯= 𝜋﷮3﷯ 0−2𝑅−6𝑥﷯ 𝑑﷮2﷯𝑣﷮𝑑 𝑥﷮2﷯﷯= −𝜋﷮3﷯ 2𝑅+6𝑥﷯ Putting 𝑥= 𝑅﷮3﷯ 𝑑﷮2﷯𝑣﷮𝑑 𝑥﷮2﷯﷯│﷮𝑥= 𝑅﷮3﷯﷯ = −𝜋﷮3﷯ 2𝑅+6 𝑅﷮3﷯﷯﷯ = −𝜋﷮3﷯ 2𝑅+2𝑅﷯ = −𝜋﷮3﷯ 4𝑅﷯ = −4𝜋𝑅﷮3﷯ < 0 Thus 𝑑﷮2﷯𝑣﷮𝑑 𝑥﷮2﷯﷯<0 when 𝑥= 𝑅﷮3﷯ ⇒ Volume is Maximum when 𝑥= 𝑅﷮3﷯ Finding maximum volume From (1) Volume of cone = 1﷮3﷯𝜋 𝑅﷮2﷯− 𝑥﷮2﷯﷯ 𝑅+𝑥﷯ Putting 𝑥 = 𝑅﷮3﷯ = 1﷮3﷯𝜋 𝑅﷮2﷯− 𝑅﷮3﷯﷯﷮2﷯﷯ 𝑅+ 𝑅﷮3﷯﷯ = 1﷮3﷯𝜋 𝑅﷮2﷯− 𝑅﷮2﷯﷮9﷯﷯ 3𝑅 + 𝑅﷮3﷯﷯ = 1﷮3﷯𝜋 9 𝑅﷮2﷯− 𝑅﷮2﷯﷮9﷯﷯ 4𝑅﷮3﷯﷯ = 𝟑𝟐﷮𝟖𝟏﷯𝝅 𝑹﷮𝟑﷯ Hence proved