Β Β
![Ex 6.3, 8 - At what points in interval [0, 2pi], does sin 2x - Ex 6.3](https://cdn.teachoo.com/5938c769-42e0-41ad-88b4-e29a83d59dca/slide22.jpg)
Ex 6.3,8 - Chapter 6 Class 12 Application of Derivatives
Last updated at Dec. 16, 2024 by Teachoo
Ex 6.3
Ex 6.3, 1 (i)
Important
You are here
You are here
Transcript
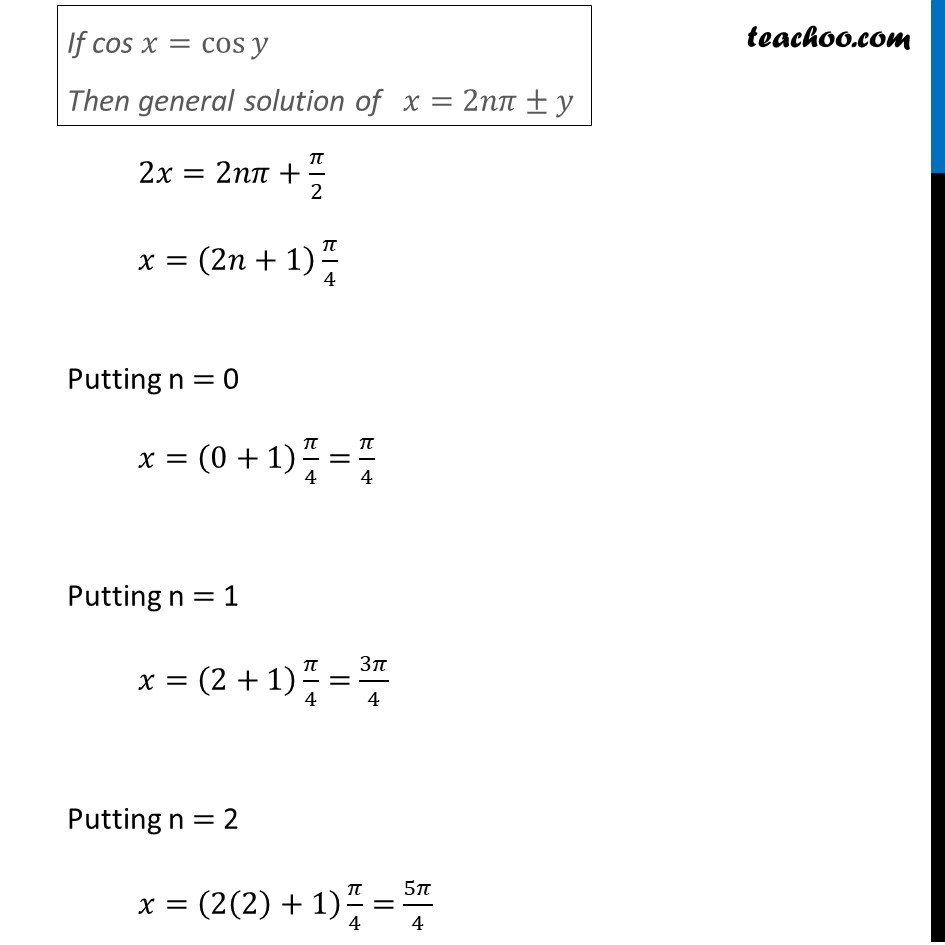
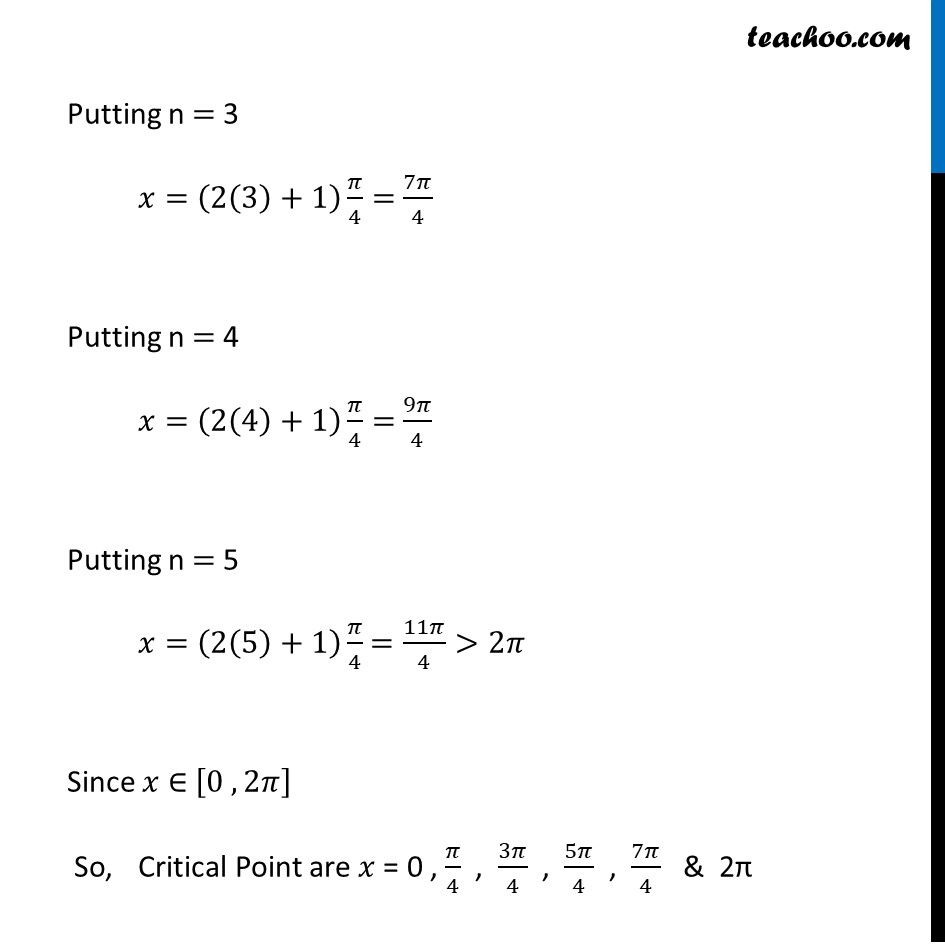
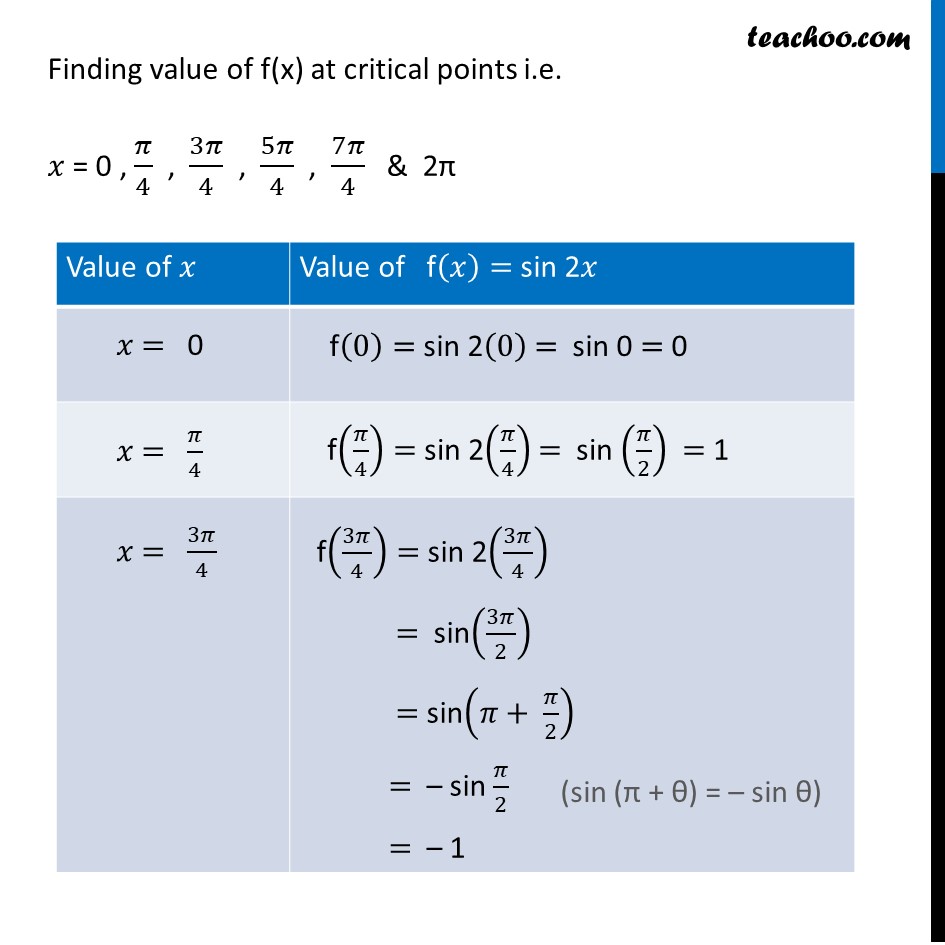
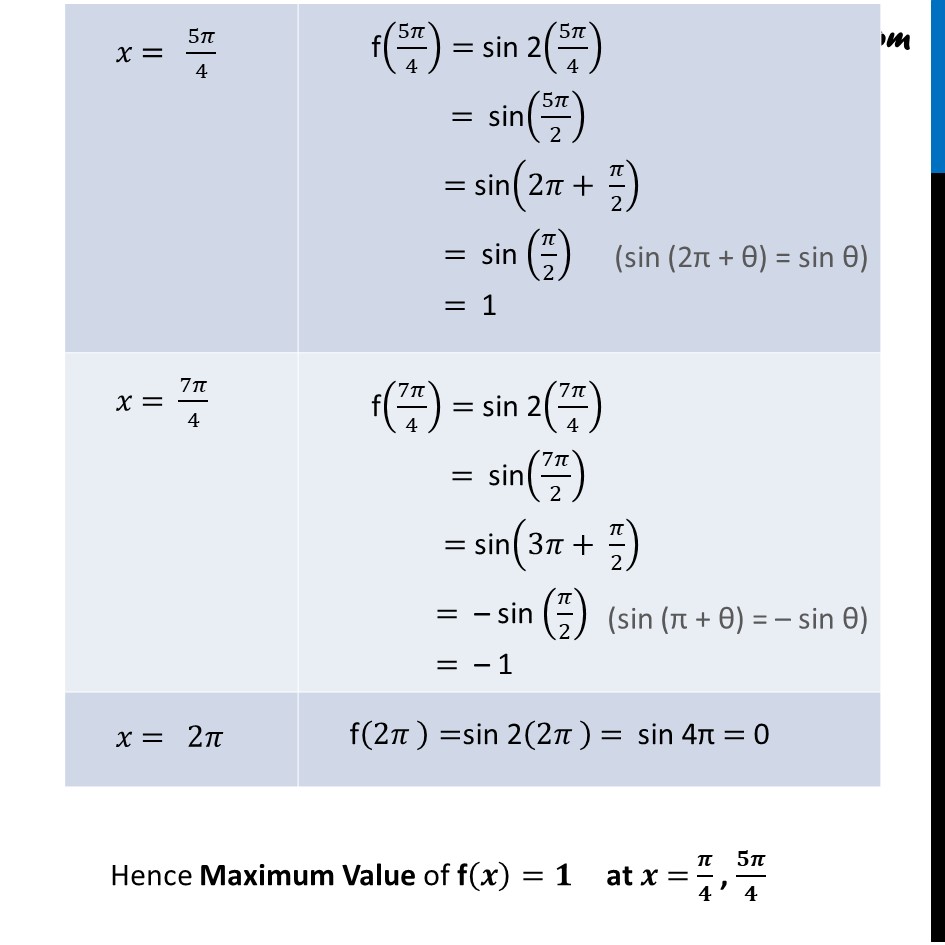
Ex 6.3, 8 At what points in the interval [0, 2Ο ], does the function sin 2π₯ attain its maximum value? Let f(π₯)=sinβ‘2π₯, π₯ β [0 , 2π] Finding fβ(π) fβ(π₯)=π(sinβ‘2π₯ )/ππ₯ fβ(π₯)=2 cosβ‘2π₯ Putting fβ(π)=π 2cos 2π₯=0 cos 2π₯=0 cos 2π₯=cosβ‘γπ/2γ 2π₯=2ππ+π/2 π₯=(2π+1) π/4 Putting n = 0 π₯=(0+1) π/4= π/4 Putting n = 1 π₯=(2+1) π/4= 3π/4 Putting n = 2 π₯=(2(2)+1) π/4= 5π/4 Putting n = 3 π₯=(2(3)+1) π/4= 7π/4 Putting n = 4 π₯=(2(4)+1) π/4= 9π/4 Putting n = 5 π₯=(2(5)+1) π/4 =11π/4>2π Since π₯ β [0 , 2π] So, Critical Point are π₯ = 0 , π/4 , 3π/4 , 5π/4 , 7π/4 & 2Ο Finding value of f(x) at critical points i.e. π₯ = 0 , π/4 , 3π/4 , 5π/4 , 7π/4 & 2Ο Finding value of f(x) at critical points i.e. π₯ = 0 , π/4 , 3π/4 , 5π/4 , 7π/4 & 2Ο
