Ex 6.3,16 - Chapter 6 Class 12 Application of Derivatives
Last updated at August 25, 2026 by Teachoo
Ex 6.3
Ex 6.3, 1 (i)
Important
You are here
You are here
Chapter 6 Class 12 Application of Derivatives
Serial order wise
Transcript
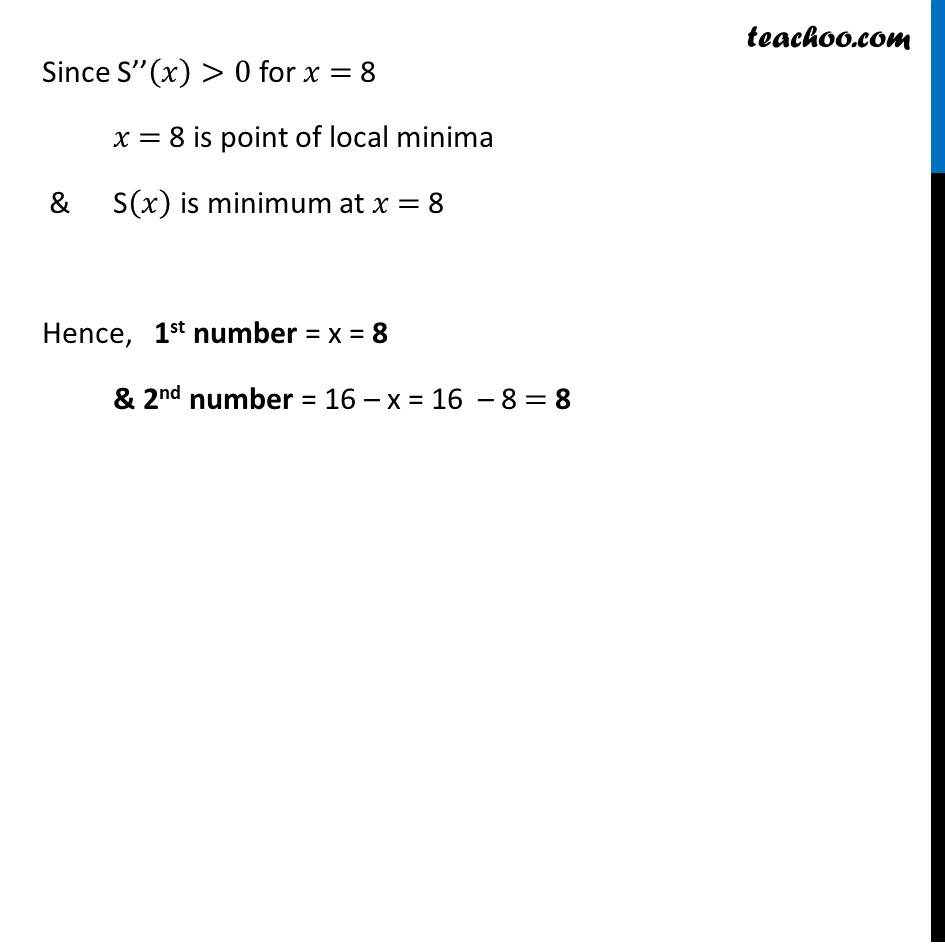
Ex 6.3, 16 Find two positive numbers whose sum is 16 and the sum of whose cubes is minimum.Let first number be 𝑥 Now, First number + second number =16 𝑥 + second number = 16 second number = 16 – 𝑥 Now, Sum of Cubes = (𝑓𝑖𝑟𝑠𝑡 𝑛𝑢𝑚𝑏𝑒𝑟 )^3+(𝑠𝑒𝑐𝑜𝑛𝑑 𝑛𝑢𝑚𝑏𝑒𝑟 )^3 Let S(𝑥) = 𝑥3 + (16−𝑥)^3 We Need to Find Minimum Value of s(𝑥) Finding S’(𝑥) S’(𝑥)= 𝑑(𝑥^3+ (16 − 𝑥)^3 )/𝑑𝑥 = 3𝑥2 + 3(16−𝑥)^2. (0−1) = 3𝑥2 + 3(16−𝑥)^2 (−1) = 3𝑥2 – 3((16)^2+(𝑥)^2−2(16)(𝑥)) = 3𝑥2 – 3(256+𝑥^2−32𝑥) = 3𝑥2 – 3(256)−3𝑥^2+3(32)𝑥 = –3(256−32𝑥) Putting S’(𝑥)=0 –3(256−32𝑥)=0 256 – 32𝑥 = 0 32𝑥 = 256 𝑥 = 256/32 𝑥 = 8 Finding S’’(𝑥) S’(𝑥)=−3(256−32𝑥) S’’(𝑥)=𝑑(−3(256 − 32𝑥))/𝑑𝑥 = –3 𝑑(256 − 32𝑥)/𝑑𝑥 = –3 [0−32] = 96 > 0 Since S’’(𝑥)>0 for 𝑥 = 8 𝑥 = 8 is point of local minima & S(𝑥) is minimum at 𝑥 = 8 Hence, 1st number = x = 8 & 2nd number = 16 – x = 16 – 8 = 8
