Ex 7.3, 16 - Chapter 7 Class 12 Integrals
Last updated at Dec. 6, 2019 by Teachoo
Transcript
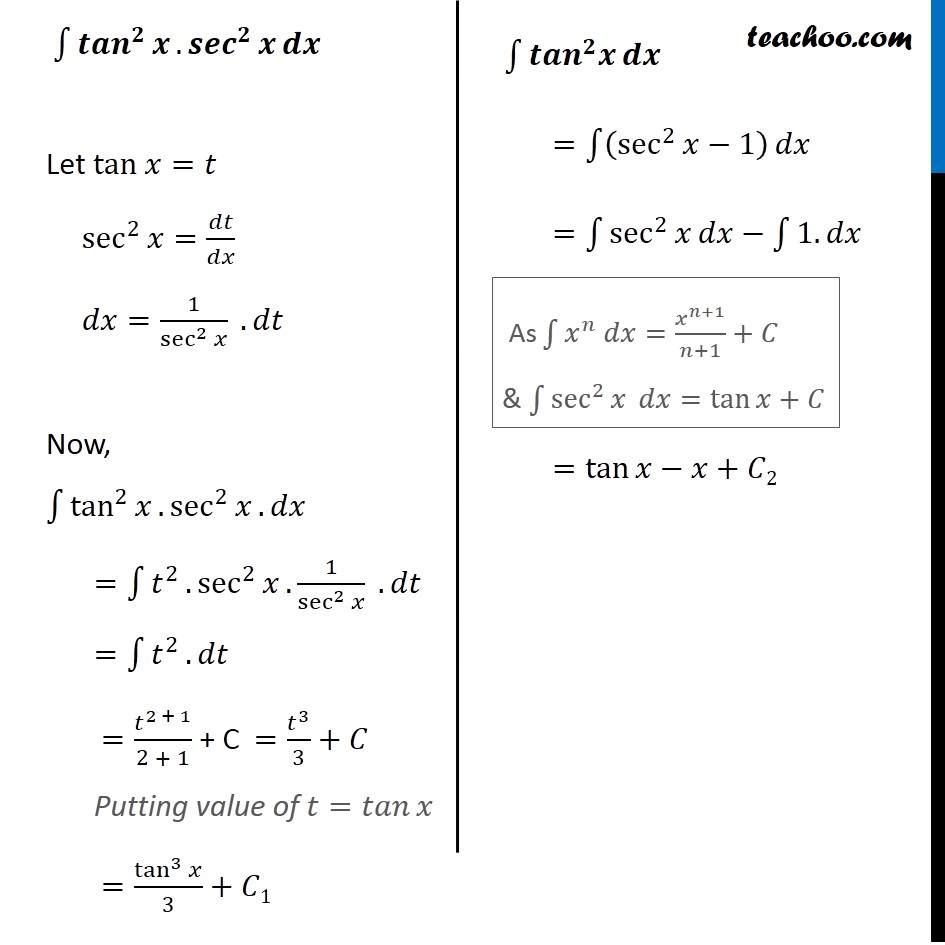
Ex 7.3, 16 tan4 𝑥𝑑𝑥 tan4 𝑥𝑑𝑥= tan2 𝑥 . tan2𝑥𝑑𝑥 = sec2𝑥−1 tan2𝑥𝑑𝑥 = sec2𝑥. tan2𝑥− tan2𝑥𝑑𝑥 = tan2𝑥. sec2𝑥𝑑𝑥− tan2𝑥𝑑𝑥 Now, tan4 𝑥𝑑𝑥= tan2 𝑥 . sec2𝑥𝑑𝑥− tan2 𝑥 𝑑𝑥 = tan3𝑥3+𝐶1− tan𝑥−𝑥+𝐶2 = tan3𝑥3 − tan𝑥+𝑥+𝐶1−𝐶2 = 𝒕𝒂𝒏𝟑𝒙𝟑 − 𝒕𝒂𝒏𝒙+𝒙+𝑪
Ex 7.3
Ex 7.3, 1
Ex 7.3, 2
Ex 7.3, 3 Important
Ex 7.3, 4 Important
Ex 7.3, 5
Ex 7.3, 6 Important
Ex 7.3, 7
Ex 7.3, 8
Ex 7.3, 9 Important
Ex 7.3, 10 Important
Ex 7.3, 11
Ex 7.3, 12
Ex 7.3, 13
Ex 7.3, 14
Ex 7.3, 15
Ex 7.3, 16 Important You are here
Ex 7.3, 17
Ex 7.3, 18 Important
Ex 7.3, 19 Important
Ex 7.3, 20 Important
Ex 7.3, 21
Ex 7.3, 22 Important
Ex 7.3, 23
Ex 7.3, 24 Important
About the Author
Davneet Singh
Davneet Singh is a graduate from Indian Institute of Technology, Kanpur. He has been teaching from the past 9 years. He provides courses for Maths and Science at Teachoo.