Ex 7.3, 3 - Chapter 7 Class 12 Integrals

Transcript

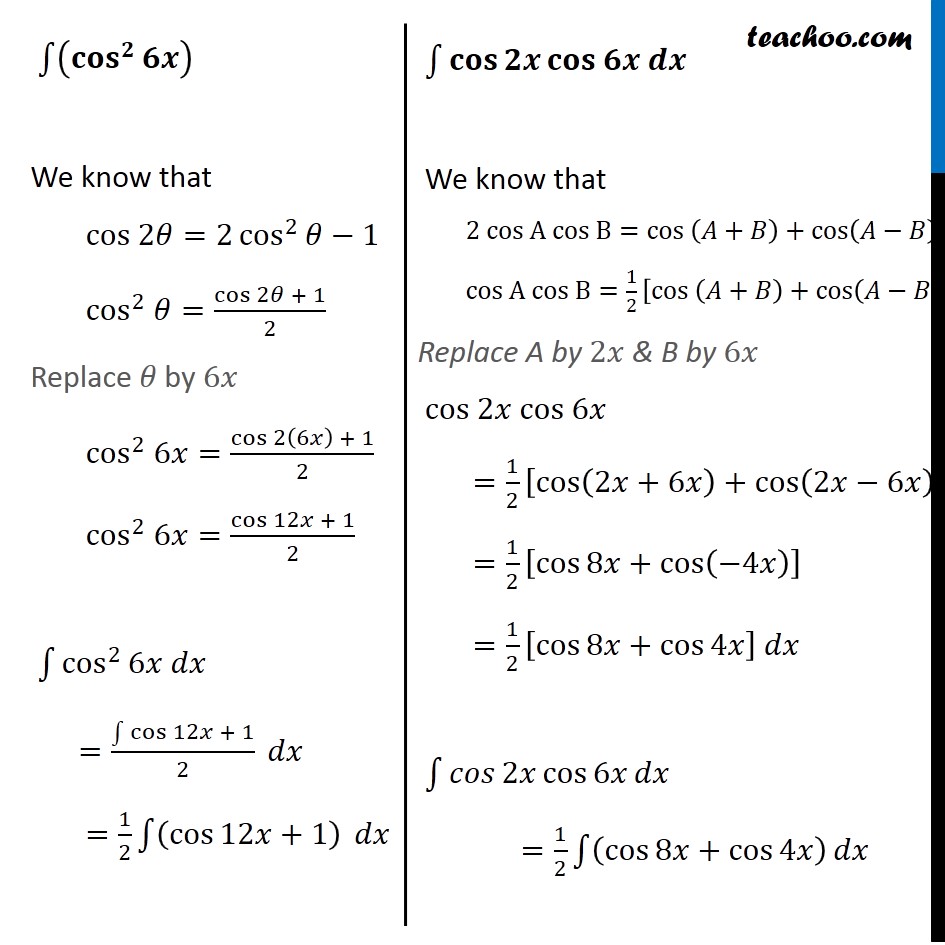
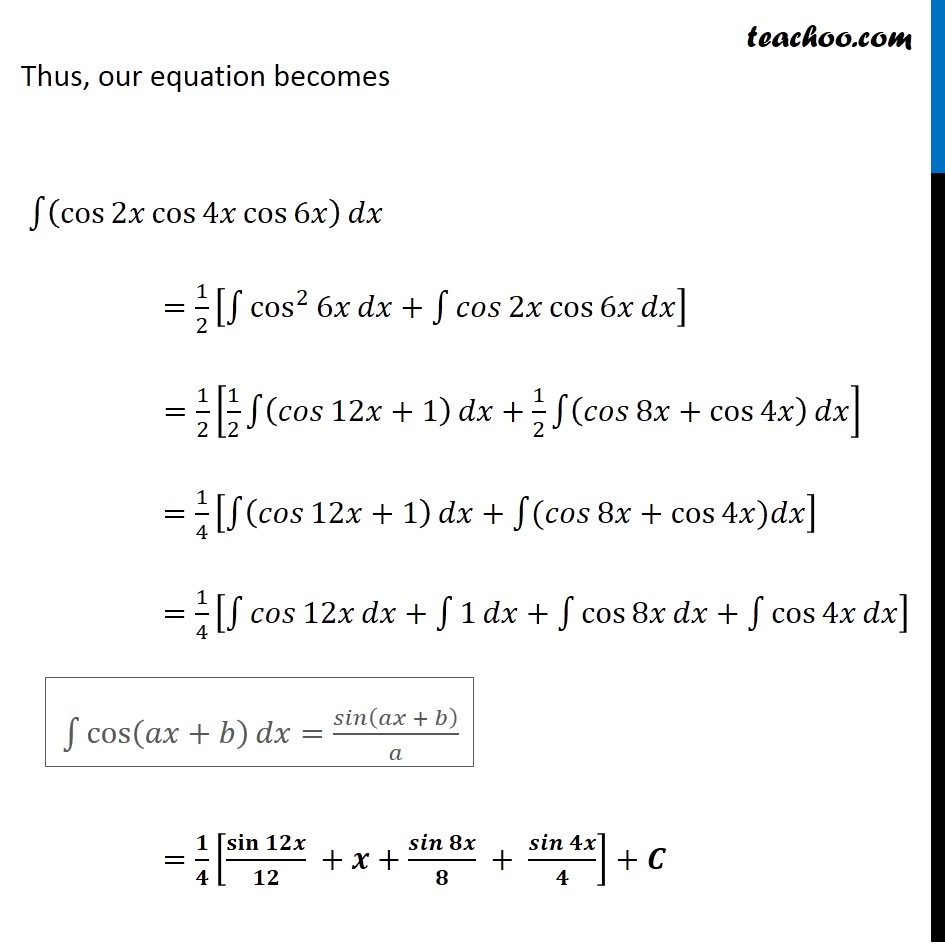
Ex 7.3, 3 Integrate the function - cos 2x cos 4x cos 6x We know that 2 cos A cos B=[cos⁡(𝐴+𝐵)+cos⁡(𝐴−𝐵) ] Replace A by 2𝑥 & B by 4𝑥 2 cos 2𝑥 cos 4𝑥=cos⁡(2𝑥+4𝑥)+cos⁡(2𝑥−4𝑥) 2(cos 2𝑥 cos 4𝑥)=cos⁡〖 (6𝑥)〗+cos⁡(−2𝑥) 2 cos 2𝑥 cos 4𝑥=cos⁡6𝑥+cos⁡2𝑥 cos 2𝑥 cos 4𝑥=1/2 (cos⁡6𝑥+cos⁡2𝑥 ) (∵𝑐𝑜𝑠⁡(−𝑥)=𝑐𝑜𝑠⁡𝑥) Now, ∫1▒(cos⁡2𝑥 cos⁡4𝑥 cos⁡6𝑥 ) 𝑑𝑥 =∫1▒(1/2 (cos⁡6𝑥+cos⁡2𝑥 ) cos⁡6𝑥 ) 𝑑𝑥 =1/2 ∫1▒〖(cos⁡6𝑥+cos⁡2𝑥 ) cos⁡6𝑥 〗 𝑑𝑥 =1/2 ∫1▒〖(cos⁡6𝑥 )^2+(cos⁡2𝑥.cos⁡6𝑥 ) 〗 𝑑𝑥 =1/2 [∫1▒〖(cos⁡6𝑥 )^2 𝑑𝑥+〗 ∫1▒〖cos⁡2𝑥.cos⁡6𝑥 〗 𝑑𝑥] Solving both these integrals separately ∫1▒(〖𝐜𝐨𝐬〗^𝟐⁡𝟔𝒙 ) We know that cos 2𝜃=2 cos^2⁡𝜃−1 cos^2 𝜃=(cos⁡2𝜃 + 1)/2 Replace 𝜃 by 6𝑥 cos^2 6𝑥=cos⁡〖2(6𝑥) + 1〗/2 cos^2 6𝑥=cos⁡〖12𝑥 + 1〗/2 ∫1▒cos^2⁡〖6𝑥 𝑑𝑥〗 =(∫1▒cos⁡〖12𝑥 + 1〗 )/2 𝑑𝑥 =1/2 ∫1▒(cos⁡〖12𝑥+1〗 ) 𝑑𝑥 ∫1▒𝐜𝐨𝐬⁡𝟐𝒙 𝐜𝐨𝐬 𝟔𝒙 𝒅𝒙 We know that 2 cos A cos B=cos (𝐴+𝐵)+cos⁡(𝐴−𝐵) cos A cos B=1/2 [cos (𝐴+𝐵)+cos⁡(𝐴−𝐵) ] Replace A by 2𝑥 & B by 6𝑥 cos 2𝑥 cos 6𝑥 =1/2 [cos⁡(2𝑥+6𝑥)+cos⁡(2𝑥−6𝑥) ] =1/2 [cos⁡8𝑥+cos⁡(−4𝑥) ] =1/2 [cos⁡8𝑥+cos⁡4𝑥 ] 𝑑𝑥 ∫1▒〖𝑐𝑜𝑠⁡2𝑥 cos⁡6𝑥 〗 𝑑𝑥 =1/2 ∫1▒(cos⁡8𝑥+cos⁡4𝑥 ) 𝑑𝑥 Thus, our equation becomes ∫1▒(cos⁡2𝑥 cos⁡4𝑥 cos⁡6𝑥 ) 𝑑𝑥 =1/2 [∫1▒cos^2⁡6𝑥 𝑑𝑥+∫1▒𝑐𝑜𝑠⁡2𝑥 cos⁡6𝑥 𝑑𝑥] =1/2 [1/2 ∫1▒(𝑐𝑜𝑠⁡〖12𝑥+1〗 ) 𝑑𝑥+1/2 ∫1▒(𝑐𝑜𝑠⁡8𝑥+cos⁡4𝑥 ) 𝑑𝑥] =1/4 [∫1▒(𝑐𝑜𝑠⁡〖12𝑥+1〗 ) 𝑑𝑥+∫1▒〖(𝑐𝑜𝑠⁡8𝑥 〗+cos⁡4𝑥)𝑑𝑥] =1/4 [∫1▒𝑐𝑜𝑠⁡12𝑥 𝑑𝑥+∫1▒1 𝑑𝑥+∫1▒cos⁡8𝑥 𝑑𝑥+∫1▒cos⁡4𝑥 𝑑𝑥] =𝟏/𝟒 [𝐬𝐢𝐧⁡𝟏𝟐𝒙/𝟏𝟐 +𝒙+𝒔𝒊𝒏⁡𝟖𝒙/𝟖 + 𝒔𝒊𝒏⁡𝟒𝒙/𝟒]+𝑪 ∫1▒cos⁡(𝑎𝑥+𝑏) 𝑑𝑥=𝑠𝑖𝑛⁡(𝑎𝑥 + 𝑏)/𝑎 =1/4 [∫1▒(𝑐𝑜𝑠⁡〖12𝑥+1〗 ) 𝑑𝑥+∫1▒〖(𝑐𝑜𝑠⁡8𝑥 〗+cos⁡4𝑥)𝑑𝑥] =1/4 [∫1▒𝑐𝑜𝑠⁡12𝑥 𝑑𝑥+∫1▒1 𝑑𝑥+∫1▒cos⁡8𝑥 𝑑𝑥+∫1▒cos⁡4𝑥 𝑑𝑥] =𝟏/𝟒 [𝐬𝐢𝐧⁡𝟏𝟐𝒙/𝟏𝟐 +𝒙+𝒔𝒊𝒏⁡𝟖𝒙/𝟖 + 𝒔𝒊𝒏⁡𝟒𝒙/𝟒]+𝑪