Ex 7.10, 11 - Chapter 7 Class 12 Integrals

Last updated at April 16, 2024 by Teachoo

Transcript

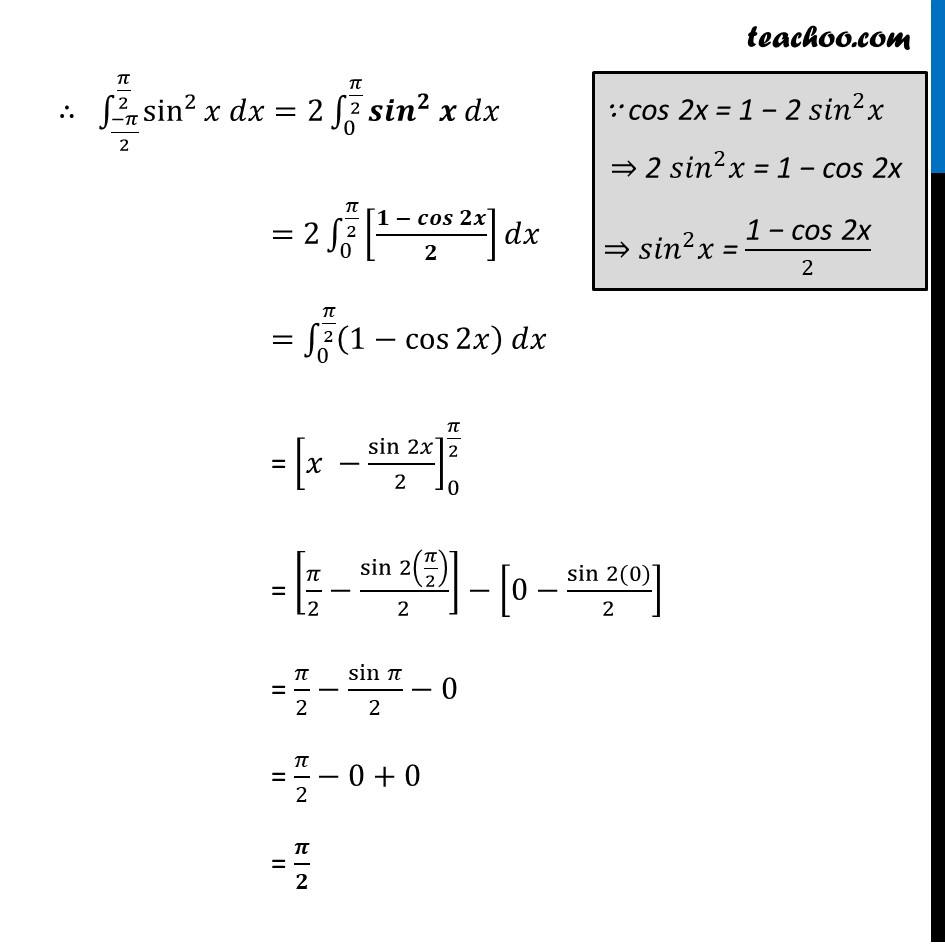
Ex 7.10, 11 By using the properties of definite integrals, evaluate the integrals : ∫_((− 𝜋)/2)^(𝜋/2)▒〖 sin^2〗⁡𝑥 𝑑𝑥 This is of form ∫_(−𝑎)^𝑎▒𝑓(𝑥)𝑑𝑥 where 𝑓(𝑥)=sin^2⁡𝑥 𝑓(−𝑥)=sin^2⁡(−𝑥)=(−𝑠𝑖𝑛𝑥)^2=sin^2⁡𝑥 ∴ 𝑓(𝑥)=𝑓(−𝑥) ∴ ∫_((−𝜋)/2)^(𝜋/2)▒〖sin^2⁡〖𝑥 𝑑𝑥〗=2∫_0^(𝜋/2)▒〖〖𝒔𝒊𝒏〗^𝟐 𝒙 𝑑𝑥〗〗 =2∫_0^(𝜋/2)▒[(𝟏 − 𝒄𝒐𝒔⁡𝟐𝒙)/𝟐]𝑑𝑥 =∫_0^(𝜋/2)▒〖(1−cos⁡2𝑥 ) 𝑑𝑥〗 = [𝑥 −sin⁡2𝑥/2]_0^(𝜋/2) = [𝜋/2−sin⁡2(𝜋/2)/2]− [0−sin⁡〖2(0)〗/2] = 𝜋/2−sin⁡𝜋/2−0 = 𝜋/2−0+0 = 𝝅/𝟐

Next: Ex 7.10, 12 Important → Ask a doubt

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular