Example 2 (i) - Chapter 7 Class 12 Integrals

Last updated at August 18, 2026 by

Example 2 - Find integrals (i) x^3 - 1 / x^2 dx (ii) x^2/3 + 1 dx

Example 2 - Chapter 7 Class 12 Integrals - Part 2

 

Remove Ads Take short quiz All Quiz and Worksheets
Teachoo · Class 12 Explore Class 12

Transcript

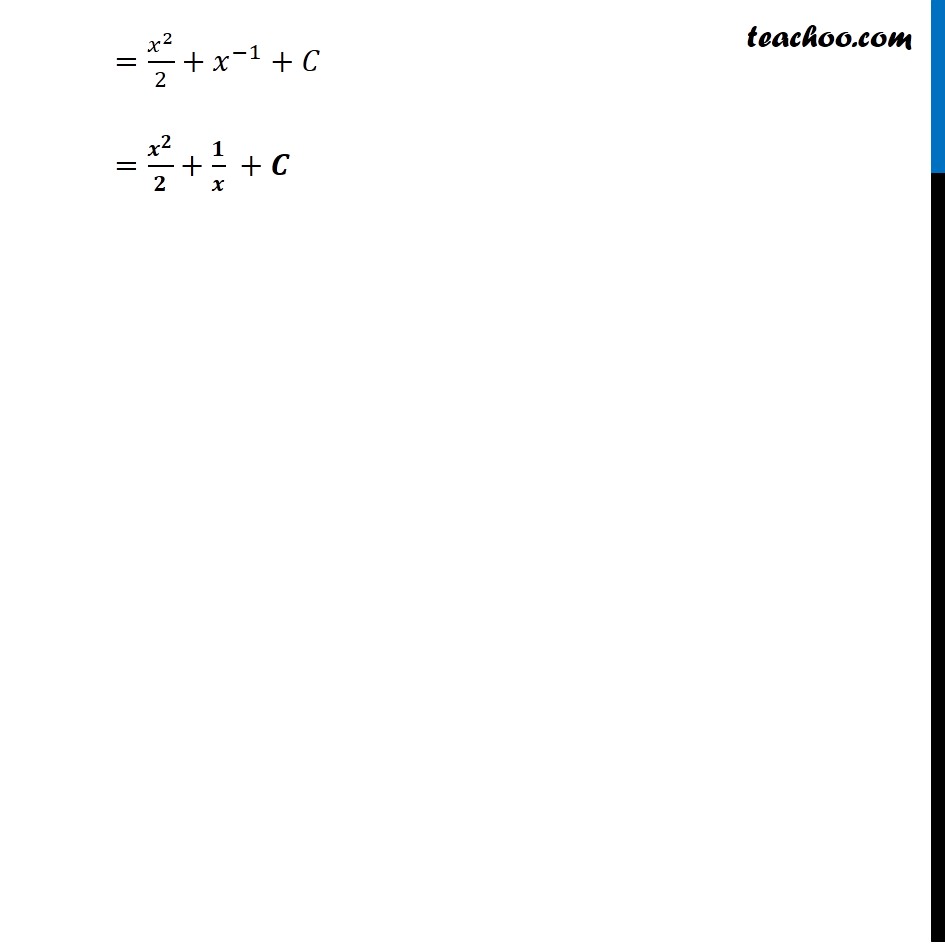
Example 2 Find the following integrals: (i) ∫▒(𝑥^3 − 1)/𝑥^2 𝑑𝑥 ∫1▒〖((𝑥^3 − 1))/𝑥^2 .𝑑𝑥〗 =∫1▒(𝑥^3/𝑥^2 −1/𝑥^2 )𝑑𝑥 =∫1▒〖(𝑥−1/𝑥^2 ).𝑑𝑥〗 =∫1▒〖𝑥 𝑑𝑥−∫1▒〖1/𝑥^2 𝑑𝑥〗〗 =∫1▒〖𝑥^1 𝑑𝑥−∫1▒〖𝑥^(−2). 𝑑𝑥〗〗 =[(𝑥^(1 + 1)/(1 + 1))]−[(𝑥^(−2 + 1)/(−2 + 1))]+𝐶 =𝑥^2/2−𝑥^(−1)/(−1)+𝐶 (█(∫1▒〖𝑥^𝑛 𝑑𝑥=𝑥^(𝑛+1)/(𝑛+1)+𝐶〗)) =𝑥^2/2+𝑥^(−1)+𝐶 =𝒙^𝟐/𝟐+𝟏/𝒙 +𝑪

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh is an IIT Kanpur graduate and has been teaching for 16+ years. At Teachoo, he breaks down Maths, Science and Computer Science into simple steps so students understand concepts deeply and score with confidence.

Many students prefer Teachoo Black for a smooth, ad-free learning experience.