Example 18 - Chapter 5 Class 12 Continuity and Differentiability
Last updated at April 16, 2024 by Teachoo
Examples
Example 1
You are here
You are here
Transcript
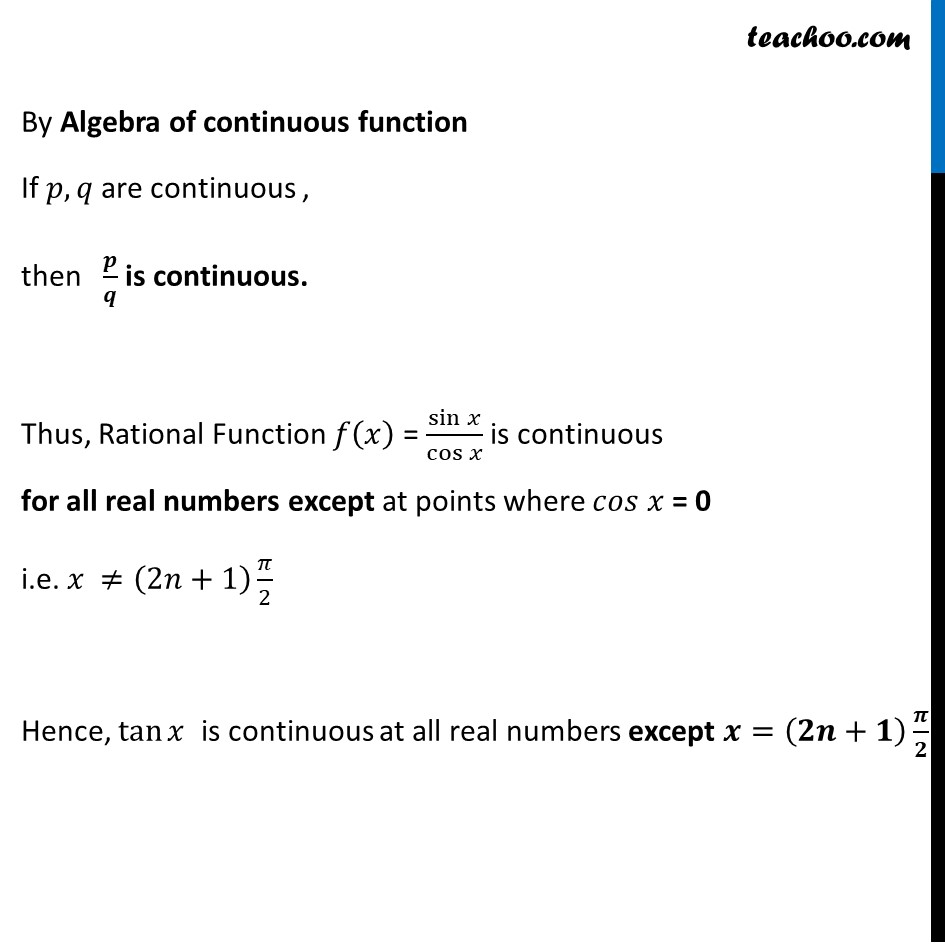
Example 18 Prove that the function defined by f (x) = tan x is a continuous function.Let π(π₯) = tanβ‘π₯ π(π) = π¬π’π§β‘π/ππ¨π¬β‘π Here, π(π₯) is defined for all real number except πππβ‘π = 0 i.e. for all x except x = (ππ+π) π /π Let π(π₯)=sinβ‘π₯ & π(π₯)=cosβ‘π₯ We know that sin x & cosβ‘π₯ is continuous for all real numbers. Therefore, π(π₯) & π(π₯) is continuous. By Algebra of continuous function If π, π are continuous , then π/π is continuous. Thus, Rational Function π(π₯) = sinβ‘π₯/cosβ‘π₯ is continuous for all real numbers except at points where πππ π₯ = 0 i.e. π₯ β (2π+1) π/2 Hence, tanβ‘π₯ is continuous at all real numbers except π=(ππ+π) π /π
