Example 17 - Chapter 5 Class 12 Continuity and Differentiability

Last updated at Dec. 16, 2024 by

  Slide44.JPG

Slide45.JPG
Slide46.JPG

Go Ad-free

Transcript

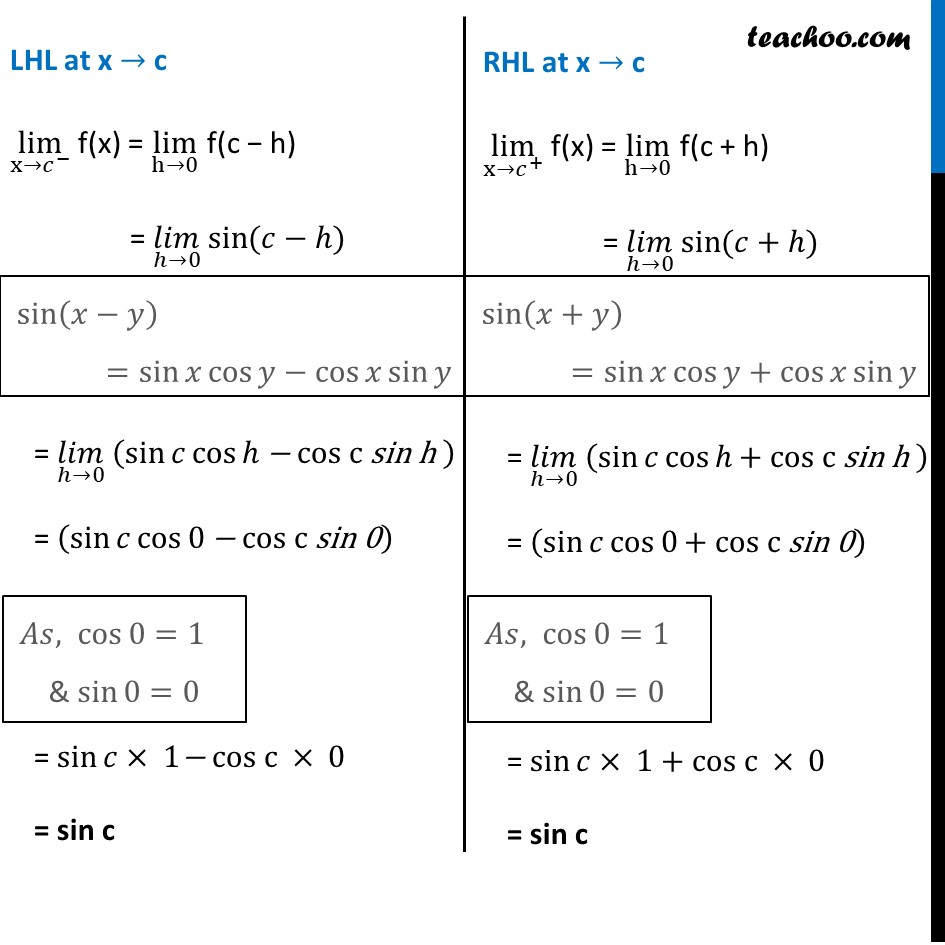
Example 17 Discuss the continuity of sine function.Let 𝑓(π‘₯)=sin⁑π‘₯ Let’s check continuity of f(x) at any real number Let c be any real number. We know that A function is continuous at π‘₯ = 𝑐 if L.H.L = R.H.L = 𝒇(𝒄) i.e. lim┬(x→𝑐^βˆ’ ) 𝑓(π‘₯)= lim┬(x→𝑐^+ ) " " 𝑓(π‘₯)= 𝑓(𝑐) LHL at x β†’ c lim┬(x→𝑐^βˆ’ ) f(x) = lim┬(hβ†’0) f(c βˆ’ h) = (π‘™π‘–π‘š)┬(β„Žβ†’0) sin⁑〖(π‘γ€—βˆ’β„Ž) = (π‘™π‘–π‘š)┬(β„Žβ†’0) (sin⁑𝑐 cosβ‘β„Ž "βˆ’ cos c sin h " ) = (sin⁑𝑐 cos⁑0 "βˆ’ cos c sin 0" ) = sin⁑𝑐× 1"βˆ’ cos c" Γ— 0 = sin c RHL at x β†’ c lim┬(x→𝑐^+ ) f(x) = lim┬(hβ†’0) f(c + h) = (π‘™π‘–π‘š)┬(β„Žβ†’0) sin⁑〖(𝑐〗+β„Ž) = (π‘™π‘–π‘š)┬(β„Žβ†’0) (sin⁑𝑐 cosβ‘β„Ž "+ cos c sin h " ) = (sin⁑𝑐 cos⁑0 "+ cos c sin 0" ) = sin⁑𝑐× 1" + cos c" Γ— 0 = sin c sin⁑(π‘₯βˆ’π‘¦) =sin⁑π‘₯ cosβ‘π‘¦βˆ’cos⁑π‘₯ sin⁑𝑦 sin⁑(π‘₯+𝑦) =sin⁑π‘₯ cos⁑𝑦+cos⁑π‘₯ sin⁑𝑦 𝐴𝑠, cos⁑0=1 & sin⁑0=0 𝐴𝑠, cos⁑0=1 & sin⁑0=0 And, 𝒇(𝒄) = π’”π’Šπ’β‘π’„ Since L.H.L = R.H.L = 𝑓(𝑐) Therefore, 𝑓(π‘₯) is continuous for all real number So, π’”π’Šπ’β‘π’™ is continuous.

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo