Ex 7.8, 16 - Chapter 7 Class 12 Integrals

Transcript

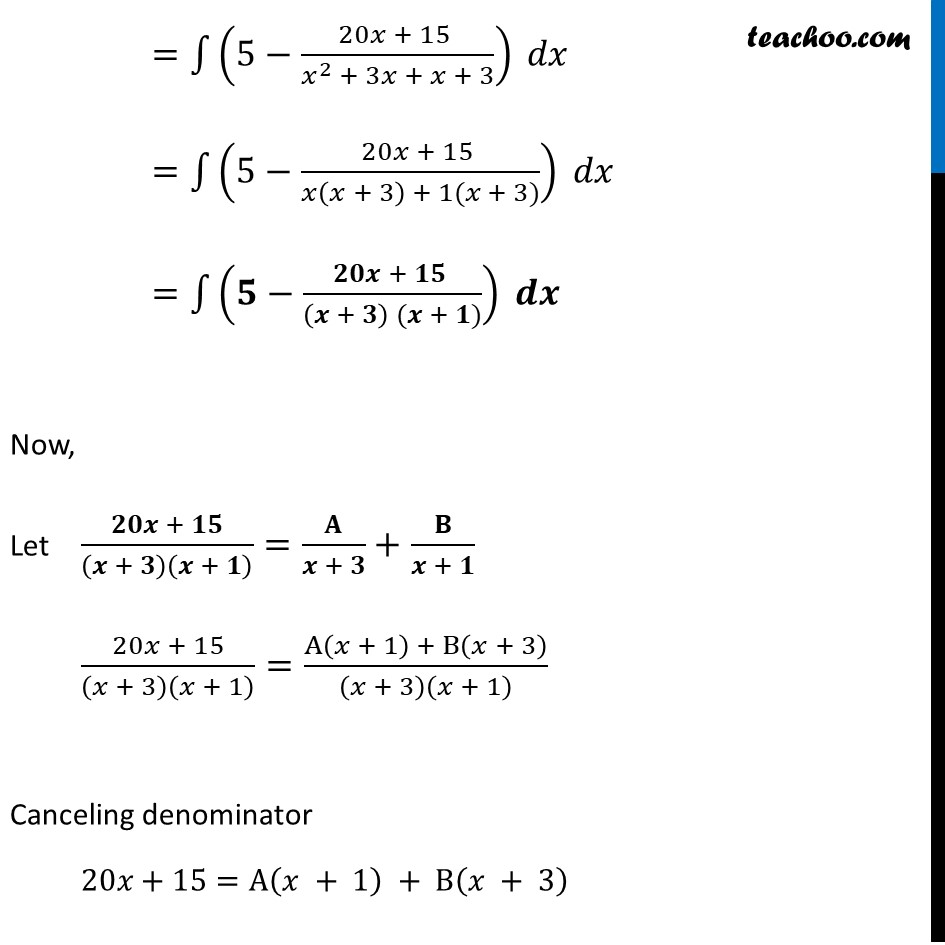
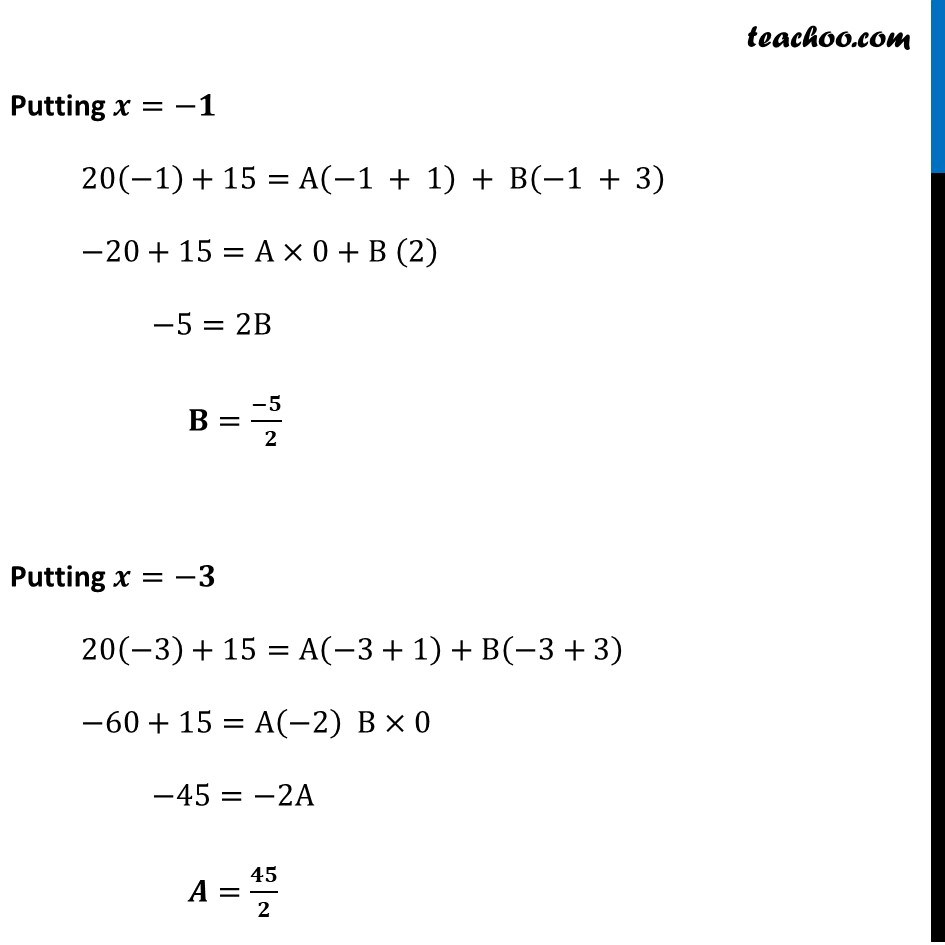
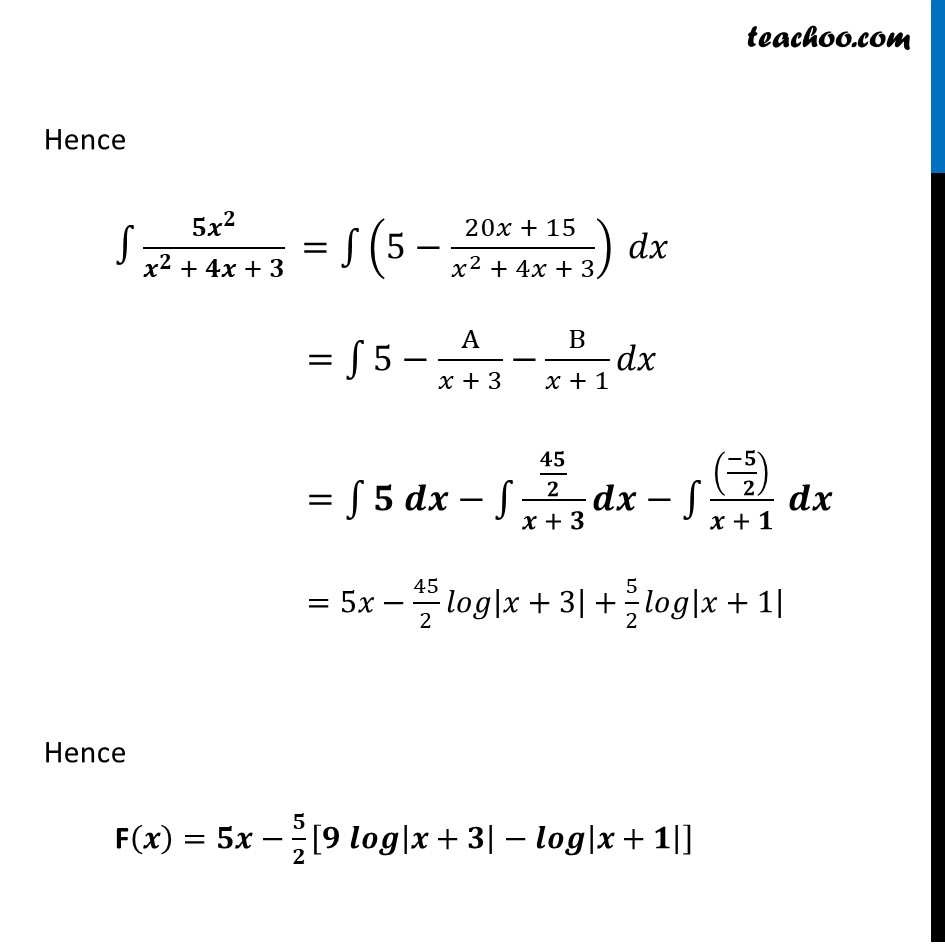
Ex 7.8, 16 ∫_1^2▒(5𝑥^2)/(𝑥^2 + 4𝑥 + 3) 𝑑𝑥 Let F(𝑥)=∫1▒〖(5𝑥^2)/(𝑥^2 + 4𝑥 + 3) 𝑑𝑥〗 =5∫1▒〖𝒙^𝟐/(𝑥^2 + 4𝑥 + 3) 𝑑𝑥〗 =5∫1▒〖(𝒙^𝟐 + 𝟒𝒙 + 𝟑 − 𝟒𝒙 − 𝟑)/(𝒙^𝟐 + 𝟒𝒙 + 𝟑) 𝑑𝑥〗 =5∫1▒〖(𝑥^2 + 4𝑥 + 3)/(𝑥^2 + 4𝑥 + 3) 𝑑𝑥〗−5∫1▒〖( (4𝑥 + 3))/(𝑥^2 + 4𝑥 + 3) 𝑑𝑥〗 =∫1▒〖(𝟓−(𝟐𝟎𝒙 + 𝟏𝟓 )/(𝒙^𝟐 + 𝟒𝒙 + 𝟑)) 𝒅𝒙〗 =∫1▒〖(5−(20𝑥 + 15 )/(𝑥^2 + 3𝑥 + 𝑥 + 3)) 𝑑𝑥〗 =∫1▒〖(5−(20𝑥 + 15 )/(𝑥(𝑥 + 3) + 1(𝑥 + 3))) 𝑑𝑥〗 =∫1▒〖(𝟓−(𝟐𝟎𝒙 + 𝟏𝟓 )/((𝒙 + 𝟑) (𝒙 + 𝟏))) 𝒅𝒙〗 Now, Let (𝟐𝟎𝒙 + 𝟏𝟓)/(𝒙 + 𝟑)(𝒙 + 𝟏) =𝐀/(𝒙 + 𝟑)+𝐁/(𝒙 + 𝟏) (20𝑥 + 15)/(𝑥 + 3)(𝑥 + 1) =(A(𝑥 + 1) + B(𝑥 + 3))/(𝑥 + 3)(𝑥 + 1) Canceling denominator 20𝑥+15=A(𝑥 + 1) + B(𝑥 + 3) Putting 𝒙=−𝟏 20(−1)+15=A(−1 + 1) + B(−1 + 3) −20+15=A×0+B (2) −5=2B 𝐁=(−𝟓)/( 𝟐) Putting 𝒙=−𝟑 20(−3)+15=A(−3+1)+B(−3+3) −60+15=A(−2) B×0 −45=−2A 𝑨=𝟒𝟓/𝟐 Hence ∫1▒█((𝟓𝒙^𝟐)/(𝒙^𝟐 + 𝟒𝒙 + 𝟑) " " =∫1▒〖(5−(20𝑥 + 15 )/(𝑥^2 + 4𝑥 + 3)) 𝑑𝑥〗) =∫1▒〖5−A/(𝑥 + 3)−〗 B/(𝑥 + 1) 𝑑𝑥 =∫1▒〖𝟓 𝒅𝒙〗−∫1▒〖(𝟒𝟓/𝟐)/(𝒙 + 𝟑) 𝒅𝒙−∫1▒〖(((−𝟓)/( 𝟐)))/(𝒙 + 𝟏) 𝒅𝒙〗〗 =5𝑥−45/2 𝑙𝑜𝑔|𝑥+3|+5/2 𝑙𝑜𝑔|𝑥+1| Hence F(𝒙)=𝟓𝒙−𝟓/𝟐 [𝟗 𝒍𝒐𝒈|𝒙+𝟑|−𝒍𝒐𝒈|𝒙+𝟏|] Now, ∫_1^2▒〖(𝟓𝒙^𝟐)/(𝒙^𝟐 + 𝟒𝒙 + 𝟑) 𝒅𝒙=𝐹(2)−𝐹(1) 〗 =[5 × 2−5/2 (9 𝑙𝑜𝑔|2+3|−𝑙𝑜𝑔|2+1|)] − [5 × 1−5/2 (9 𝑙𝑜𝑔|1+3|−𝑙𝑜𝑔|1+1|)] =10−5/2 [9 𝑙𝑜𝑔 5−𝑙𝑜𝑔 3]−5+5/2 [9 𝑙𝑜𝑔 4−𝑙𝑜𝑔 2] =10−5−5/2 [9𝑙𝑜𝑔 5−𝑙𝑜𝑔 3−9𝑙𝑜𝑔 4+𝑙𝑜𝑔 2)] =10−5−5/2 [9𝑙𝑜𝑔 5−9𝑙𝑜𝑔 4−(𝑙𝑜𝑔 3−𝑙𝑜𝑔 2)] =𝟓−𝟓/𝟐 (𝟗 𝐥𝐨𝐠 𝟓/𝟒−𝐥𝐨𝐠 𝟑/𝟐)