Ex 7.4, 22 - Chapter 7 Class 12 Integrals (Term 2)

Transcript

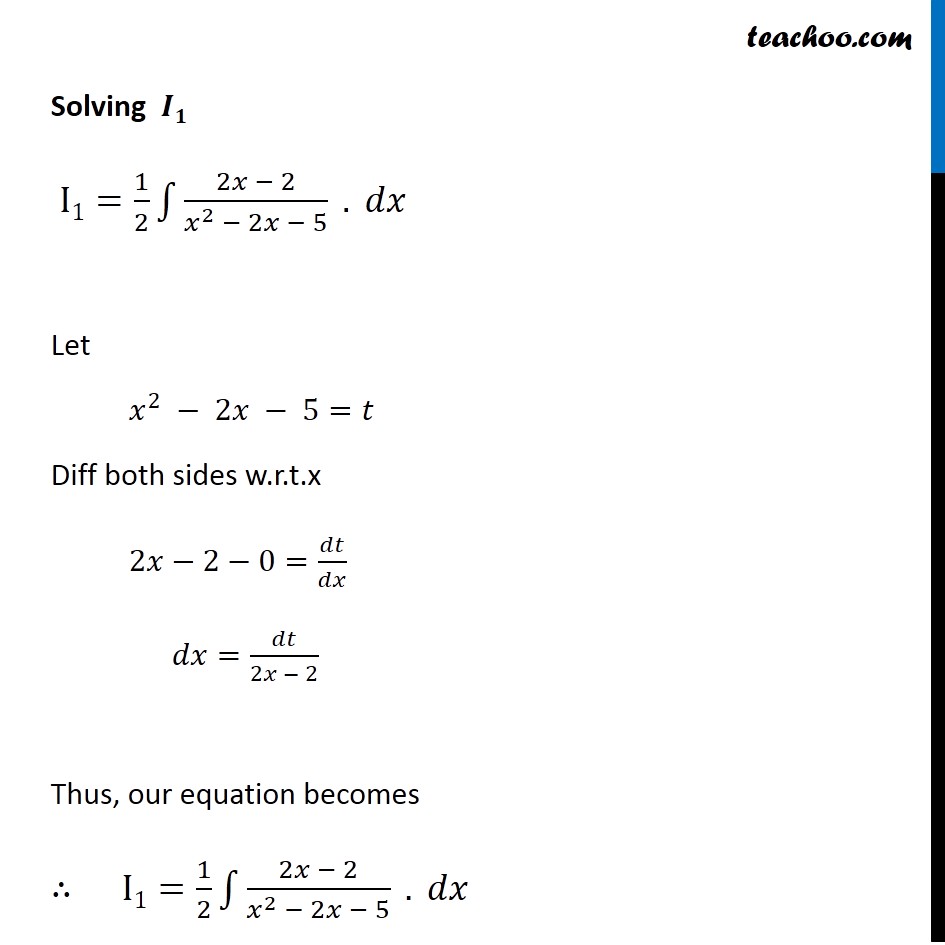
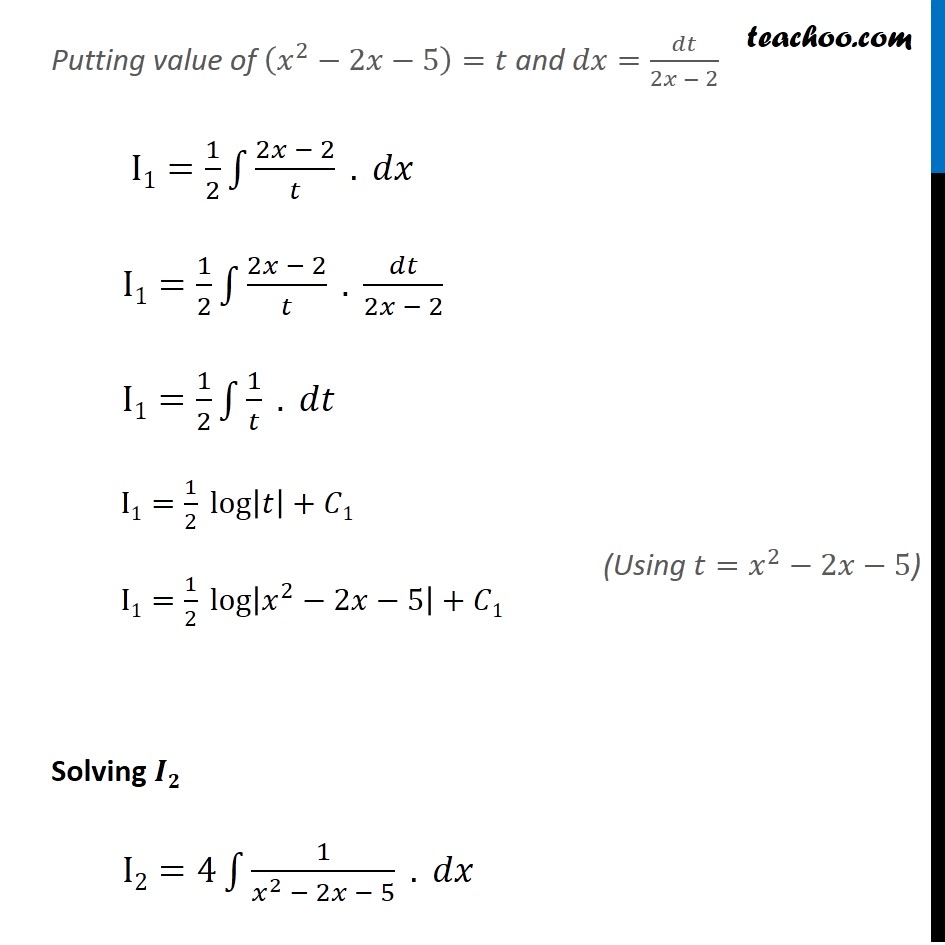
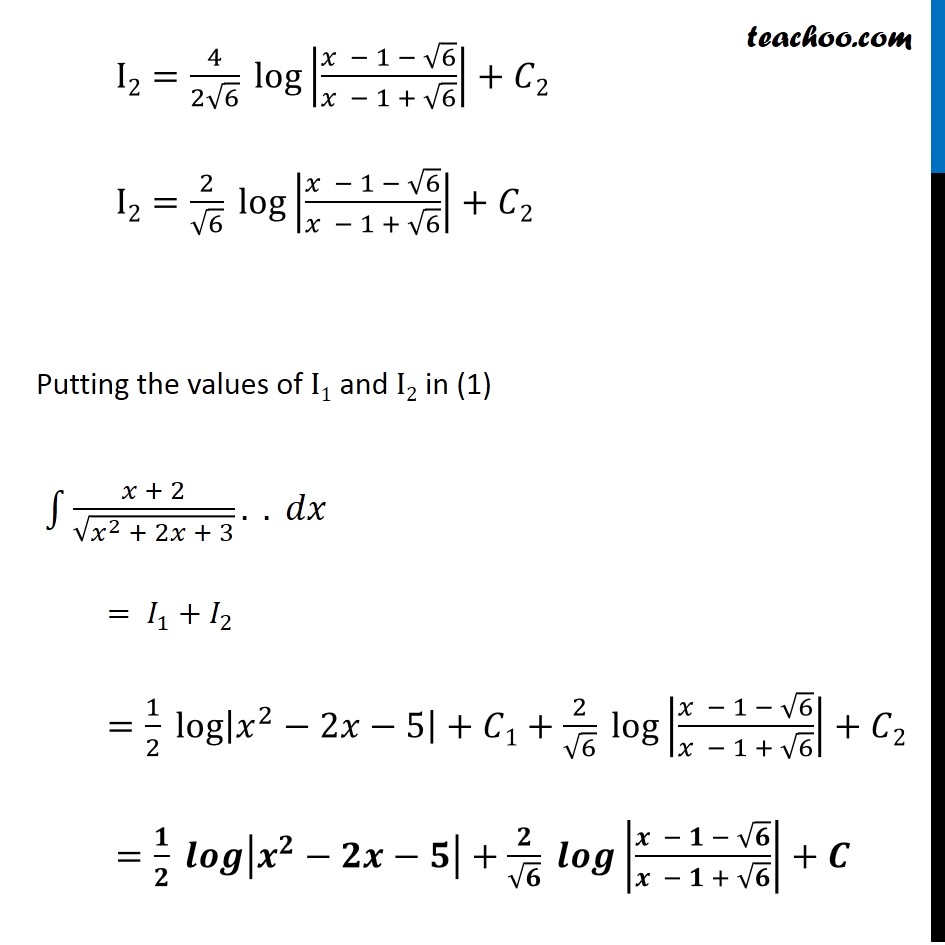
Ex 7.4, 22 Integrate the function (𝑥 + 3)/(𝑥^2 − 2𝑥 − 5) ∫1▒(𝑥 + 3)/(𝑥^2 − 2𝑥 − 5) 𝑑𝑥 =1/2 ∫1▒(2𝑥 + 6)/(𝑥^2 − 2𝑥 − 5) 𝑑𝑥 =1/2 ∫1▒(2𝑥 − 2 + 2 + 6 )/(𝑥^2 − 2𝑥 − 5) 𝑑𝑥 =1/2 ∫1▒(2𝑥 − 2)/(𝑥^2 − 2𝑥 − 5) 𝑑𝑥+8/2 ∫1▒𝑑𝑥/(𝑥^2 − 2𝑥 − 5) =1/2 ∫1▒(2𝑥 − 2)/(𝑥^2 − 2𝑥 − 5) 𝑑𝑥+4∫1▒𝑑𝑥/(𝑥^2 − 2𝑥 − 5) Rough (𝑥^2−2𝑥−5)^′=2𝑥−2 Solving 𝑰𝟏 I1=1/2 ∫1▒(2𝑥 − 2)/(𝑥^2 − 2𝑥 − 5) . 𝑑𝑥 Let 𝑥^2 − 2𝑥 − 5=𝑡 Diff both sides w.r.t.x 2𝑥−2−0=𝑑𝑡/𝑑𝑥 𝑑𝑥=𝑑𝑡/(2𝑥 − 2) Thus, our equation becomes ∴ I1=1/2 ∫1▒(2𝑥 − 2)/(𝑥^2 − 2𝑥 − 5) . 𝑑𝑥 Putting value of (𝑥^2−2𝑥−5)=𝑡 and 𝑑𝑥=𝑑𝑡/(2𝑥 − 2) I1=1/2 ∫1▒(2𝑥 − 2)/𝑡 . 𝑑𝑥 I1=1/2 ∫1▒(2𝑥 − 2)/𝑡 . 𝑑𝑡/(2𝑥 − 2) I1=1/2 ∫1▒1/𝑡 . 𝑑𝑡 I1=1/2 log⁡|𝑡|+𝐶1 I1=1/2 log⁡|𝑥^2−2𝑥−5|+𝐶1 Solving 𝑰𝟐 I2=4∫1▒1/(𝑥^2 − 2𝑥 − 5) . 𝑑𝑥 (Using 𝑡=𝑥^2−2𝑥−5) I2=4∫1▒1/(𝑥^2 − 2(𝑥)(1) − 5) . 𝑑𝑥 I2=4∫1▒1/(𝑥^2 − 2(𝑥)(1) + (1)^2 − (1)^2 − 5) . 𝑑𝑥 I2=4∫1▒1/((𝑥 − 1)^2 − (1)^2 − 5) . I2=4∫1▒1/((𝑥 − 1)^2 − 1 − 5) . 𝑑𝑥 I2=4∫1▒1/((𝑥 − 1)^2 − 6) . 𝑑𝑥 I2=4∫1▒1/((𝑥 − 1)^2 −(√6 )^2 ) . 𝑑𝑥 It is of form ∫1▒𝑑𝑥/(𝑥^2 − 𝑎^2 ) =1/2𝑎 log⁡|(𝑥 − 𝑎)/(𝑥 + 𝑎)|+𝐶 ∴ Replacing 𝑥 by (𝑥−1) and a by √6 , we get I2=4/(2√6) log⁡|(𝑥 − 1 − √6)/(𝑥 − 1 + √6)|+𝐶2 I2=2/√6 log⁡|(𝑥 − 1 − √6)/(𝑥 − 1 + √6)|+𝐶2 Putting the values of I1 and I2 in (1) ∫1▒〖(𝑥 + 2)/√(𝑥^2 + 2𝑥 + 3).〗 . 𝑑𝑥 = 𝐼_1+𝐼_2 =1/2 log⁡|𝑥^2−2𝑥−5|+𝐶1+2/√6 log⁡|(𝑥 − 1 − √6)/(𝑥 − 1 + √6)|+𝐶"2 " =𝟏/𝟐 𝒍𝒐𝒈⁡|𝒙^𝟐−𝟐𝒙−𝟓|+𝟐/√𝟔 𝒍𝒐𝒈⁡|(𝒙 − 𝟏 − √𝟔)/(𝒙 − 𝟏 + √𝟔)|+𝑪