![How to check continuity of secant function? [Video] - Teachoo](https://d1avenlh0i1xmr.cloudfront.net/f589c1c1-d16b-4690-b153-fcf3b6db702e/slide6.jpg)
Ex 5.1, 22 (iii) - Chapter 5 Class 12 Continuity and Differentiability
Last updated at April 16, 2024 by Teachoo
Ex 5.1
Ex 5.1 ,1
Ex 5.1 ,2
Ex 5.1, 3 (a)
Ex 5.1, 3 (b)
Ex 5.1, 3 (c) Important
Ex 5.1, 3 (d) Important
Ex 5.1 ,4
Ex 5.1 ,5 Important
Ex 5.1 ,6
Ex 5.1 ,7 Important
Ex 5.1 ,8
Ex 5.1, 9 Important
Ex 5.1, 10
Ex 5.1, 11
Ex 5.1, 12 Important
Ex 5.1, 13
Ex 5.1, 14
Ex 5.1, 15 Important
Ex 5.1, 16
Ex 5.1, 17 Important
Ex 5.1, 18 Important
Ex 5.1, 19 Important
Ex 5.1, 20
Ex 5.1, 21
Ex 5.1, 22 (i) Important
Ex 5.1, 22 (ii)
Ex 5.1, 22 (iii) You are here
Ex 5.1, 22 (iv) Important
Ex 5.1, 23
Ex 5.1, 24 Important
Ex 5.1, 25
Ex 5.1, 26 Important
Ex 5.1, 27
Ex 5.1, 28 Important
Ex 5.1, 29
Ex 5.1, 30 Important
Ex 5.1, 31
Ex 5.1, 32
Ex 5.1, 33
Ex 5.1, 34 Important
Transcript
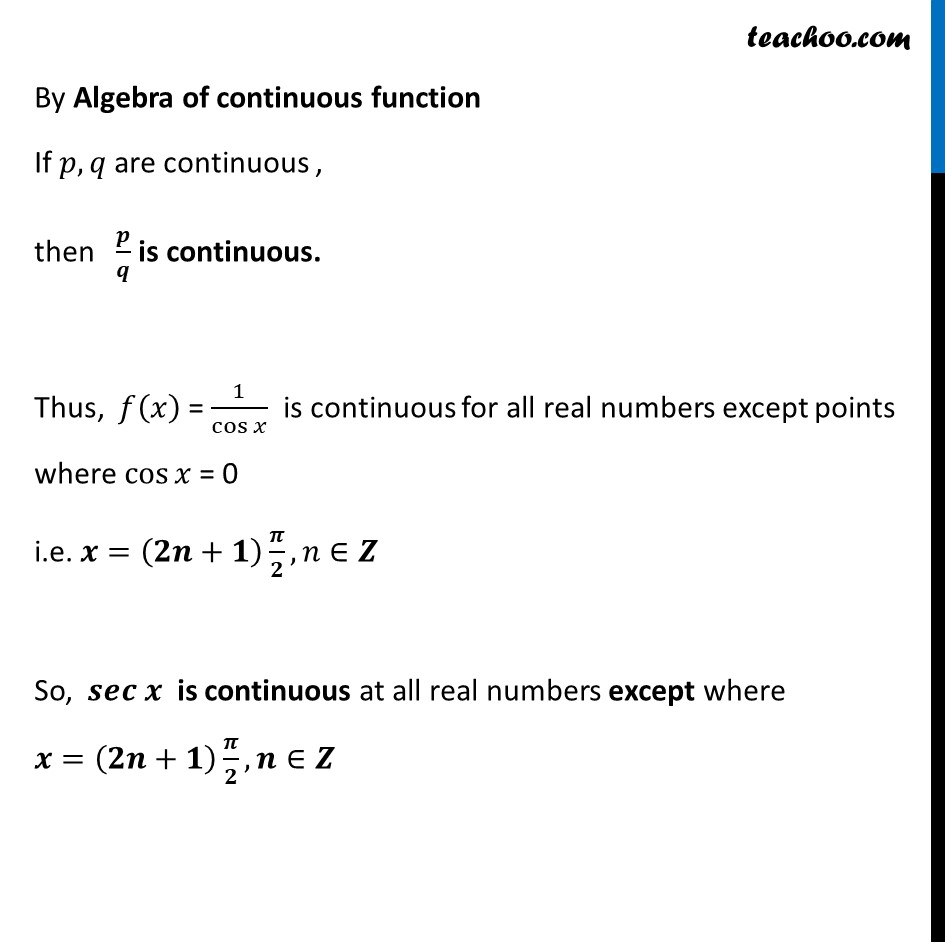
Ex 5.1, 22 (iii) Discuss the continuity of the cosine, cosecant, secant and cotangent functions. Let 𝒇(𝒙)="sec x" 𝑓(𝑥) = 1/c𝑜𝑠𝑥 Let 𝒑(𝒙)=1 & 𝒒(𝒙)=𝑐𝑜𝑠𝑥 Since, p(x) is a constant, ∴ 𝑝(𝑥) is continuous We know that cos𝑥 is continuous for all real number ∴ 𝑞(𝑥) is continuous By Algebra of continuous function If 𝑝, 𝑞 are continuous , then 𝒑/𝒒 is continuous. Thus, 𝑓(𝑥) = 1/cos𝑥 is continuous for all real numbers except points where cos𝑥 = 0 i.e. 𝒙=(𝟐𝒏+𝟏) 𝝅/𝟐, 𝑛∈𝒁 So, 𝒔𝒆𝒄 𝒙 is continuous at all real numbers except where 𝒙=(𝟐𝒏+𝟏) 𝝅/𝟐, 𝒏∈𝒁
