Ex 9.3, 20 - Chapter 9 Class 12 Differential Equations

Transcript

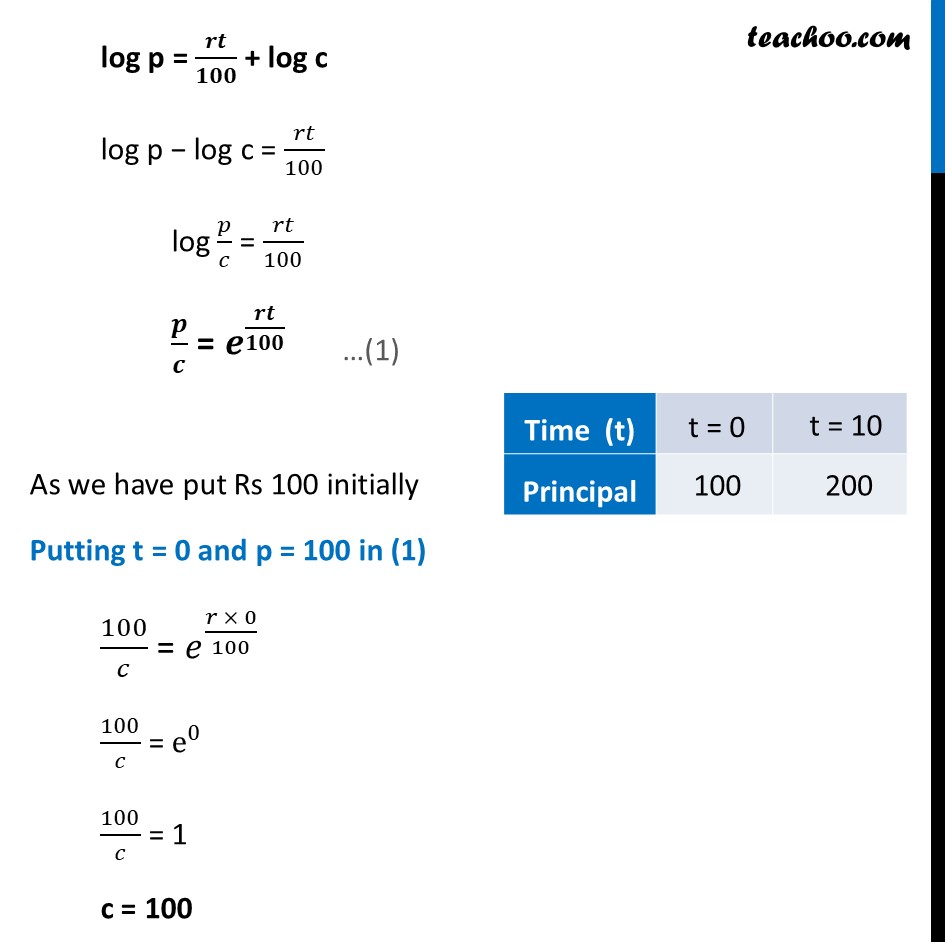
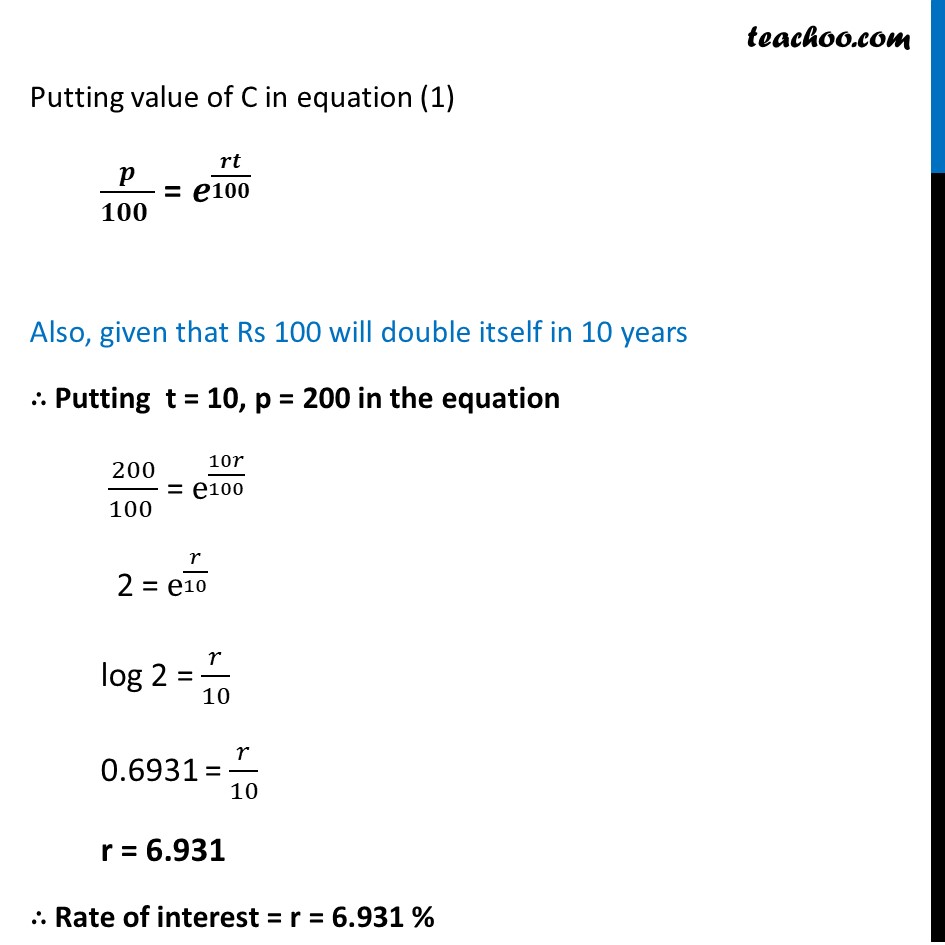
Ex 9.3, 20 In a bank, principal increases continuously at the rate of 𝑟% per year. Find the value of r if Rs 100 double itself in 10 years (log⁡〖2=0.6931〗 )Let Principal = p Given, principal increases ar rate r % per year ∴ 𝒅𝒑/𝒅𝒕 = 𝒓 % × P ∴ 𝑑𝑝/𝑑𝑡 = 𝑟/100 × p 𝒅𝒑/𝒑 = 𝒓/𝟏𝟎𝟎 dt Integrating both sides ∫1▒𝑑𝑝/𝑝 = 𝑟/100 ∫1▒𝑑𝑡 log p = 𝒓𝒕/𝟏𝟎𝟎 + log c log p − log c = 𝑟𝑡/100 log 𝑝/𝑐 = 𝑟𝑡/100 𝒑/𝒄 = 𝒆^(𝒓𝒕/𝟏𝟎𝟎) As we have put Rs 100 initially Putting t = 0 and p = 100 in (1) 100/𝑐 = 𝑒^((𝑟 × 0)/100) 100/𝑐 = e^0 100/𝑐 = 1 c = 100 Putting value of C in equation (1) 𝒑/(𝟏𝟎𝟎 ) = 𝒆^(𝒓𝒕/𝟏𝟎𝟎) Also, given that Rs 100 will double itself in 10 years ∴ Putting t = 10, p = 200 in the equation 200/(100 ) = e^(10𝑟/100) 2 = e^(𝑟/10) log 2 = 𝑟/10 0.6931 = 𝑟/10 r = 6.931 ∴ Rate of interest = r = 6.931 %