Question 11 - Tangents and Normals (using Differentiation) - Chapter 6 Class 12 Application of Derivatives
Last updated at April 16, 2024 by Teachoo
Tangents and Normals (using Differentiation)
Question 1
Deleted for CBSE Board 2025 Exams
You are here
You are here
Chapter 6 Class 12 Application of Derivatives
Serial order wise
- Ex 6.1
- Ex 6.2
- Ex 6.3
- Examples
- Miscellaneous
- Case Based Questions (MCQ)
- NCERT Exemplar - MCQs
-
Tangents and Normals (using Differentiation)
- Approximations (using Differentiation)
Transcript
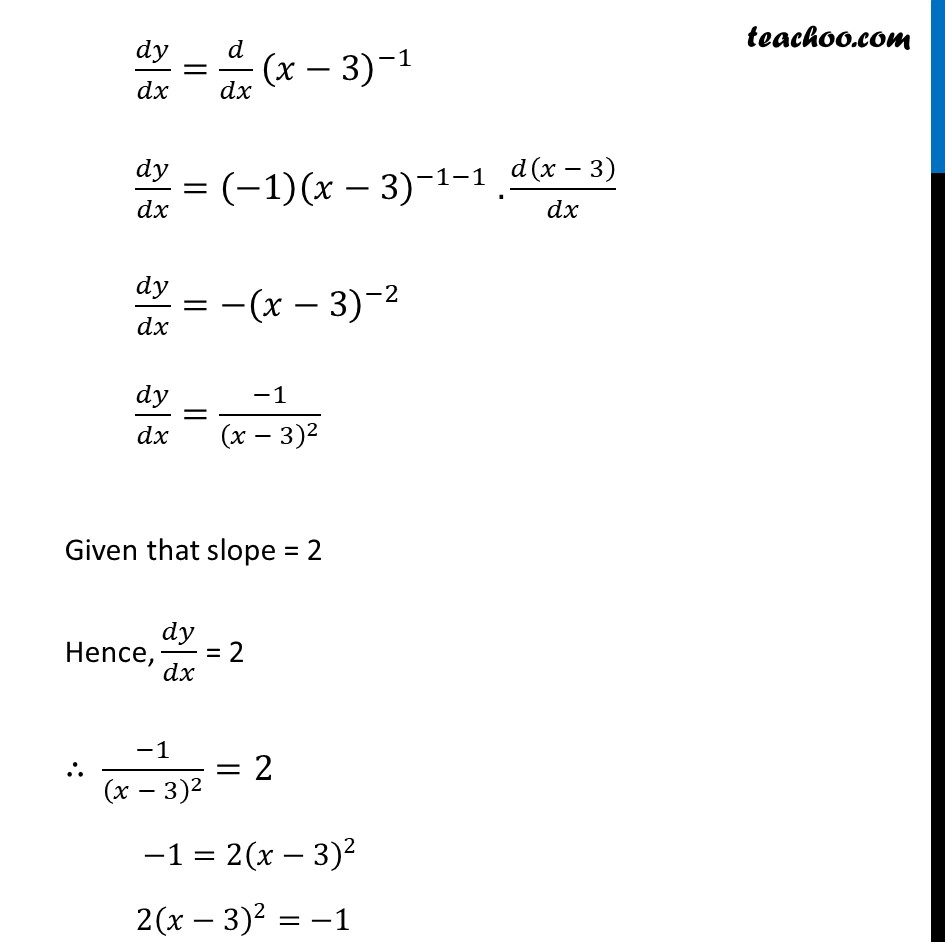
Question 11 Find the equation of all lines having slope 2 which are tangents to the curve 𝑦=1/(𝑥 − 3) , 𝑥≠3.The Equation of Given Curve is : 𝑦=1/(𝑥 − 3) We know that Slope of tangent is 𝑑𝑦/𝑑𝑥 𝑑𝑦/𝑑𝑥=𝑑(1/(𝑥 − 3))/𝑑𝑥 𝑑𝑦/𝑑𝑥=𝑑/𝑑𝑥 (𝑥−3)^(−1) 𝑑𝑦/𝑑𝑥=(−1) (𝑥−3)^(−1−1) . 𝑑(𝑥 − 3)/𝑑𝑥 𝑑𝑦/𝑑𝑥=−(𝑥−3)^(−2) 𝑑𝑦/𝑑𝑥=(−1)/(𝑥 − 3)^2 Given that slope = 2 Hence, 𝑑𝑦/𝑑𝑥 = 2 ∴ (−1)/(𝑥 − 3)^2 =2 −1=2(𝑥−3)^2 〖2(𝑥−3)〗^2=−1 (𝑥−3)^2=(−1)/( 2) We know that Square of any number is always positive So, (𝑥−3)^2>0 ∴ (𝑥−3)^2=(−1)/( 2) not possible Thus, No tangent to the Curve has Slope 2
