Question 9 - Tangents and Normals (using Differentiation) - Chapter 6 Class 12 Application of Derivatives

Last updated at Dec. 16, 2024 by

Ex 6.3, 9 - Find point on y = x3 - 11x + 5 at which tangent

Ex 6.3,9 - Chapter 6 Class 12 Application of Derivatives - Part 2
Ex 6.3,9 - Chapter 6 Class 12 Application of Derivatives - Part 3

Remove Ads

Transcript

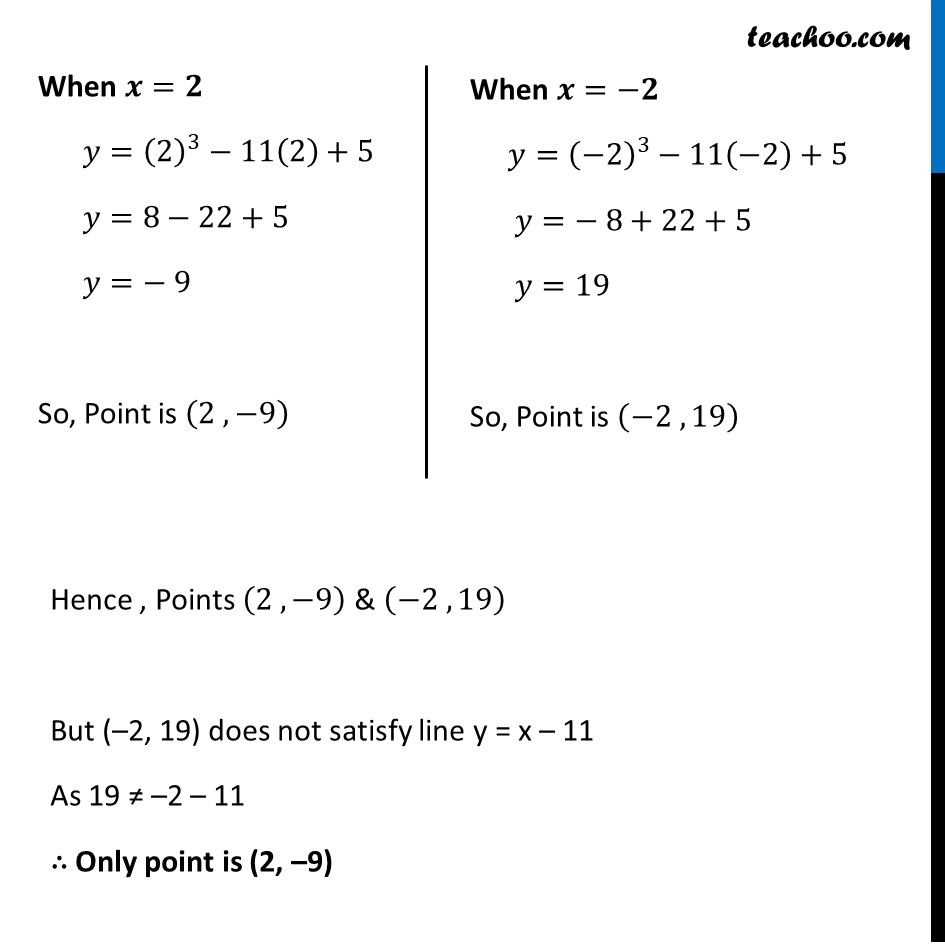
Question 9 Find the point on the curve 𝑦=𝑥^3−11𝑥+5 at which the tangent is 𝑦=𝑥 −11.Equation of Curve is 𝑦=𝑥^3−11𝑥+5 We know that Slope of tangent is 𝑑𝑦/𝑑𝑥 𝑑𝑦/𝑑𝑥=𝑑(𝑥^3 − 11𝑥 + 5)/𝑑𝑥 𝑑𝑦/𝑑𝑥=〖3𝑥〗^2−11 Also, Given tangent is 𝑦=𝑥−12 Comparing with 𝑦=𝑚𝑥+𝑐 , when m is the Slope Slope of tangent =1 From (1) and (2) 𝑑𝑦/𝑑𝑥=1 3𝑥^2−11=1 3𝑥^2=1+11 3𝑥^2=12 𝑥^2=12/3 𝑥^2=4 𝑥=±2 When 𝒙=𝟐 𝑦=(2)^3−11(2)+5 𝑦=8−22+5 𝑦=− 9 So, Point is (2 , −9) When 𝒙=−𝟐 𝑦=(−2)^3−11(−2)+5 𝑦=− 8+22+5 𝑦=19 So, Point is (−2 , 19) Hence , Points (2 , −9) & (−2 , 19) But (–2, 19) does not satisfy line y = x – 11 As 19 ≠ –2 – 11 ∴ Only point is (2, –9)

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo