Ex 3.3, 6 - Chapter 3 Class 11 Trigonometric Functions

Last updated at Feb. 13, 2020 by Teachoo

Learn All Concepts of Chapter 2 Class 11 Relations and Function - FREE. Check - Trigonometry Class 11 - All Concepts

Transcript

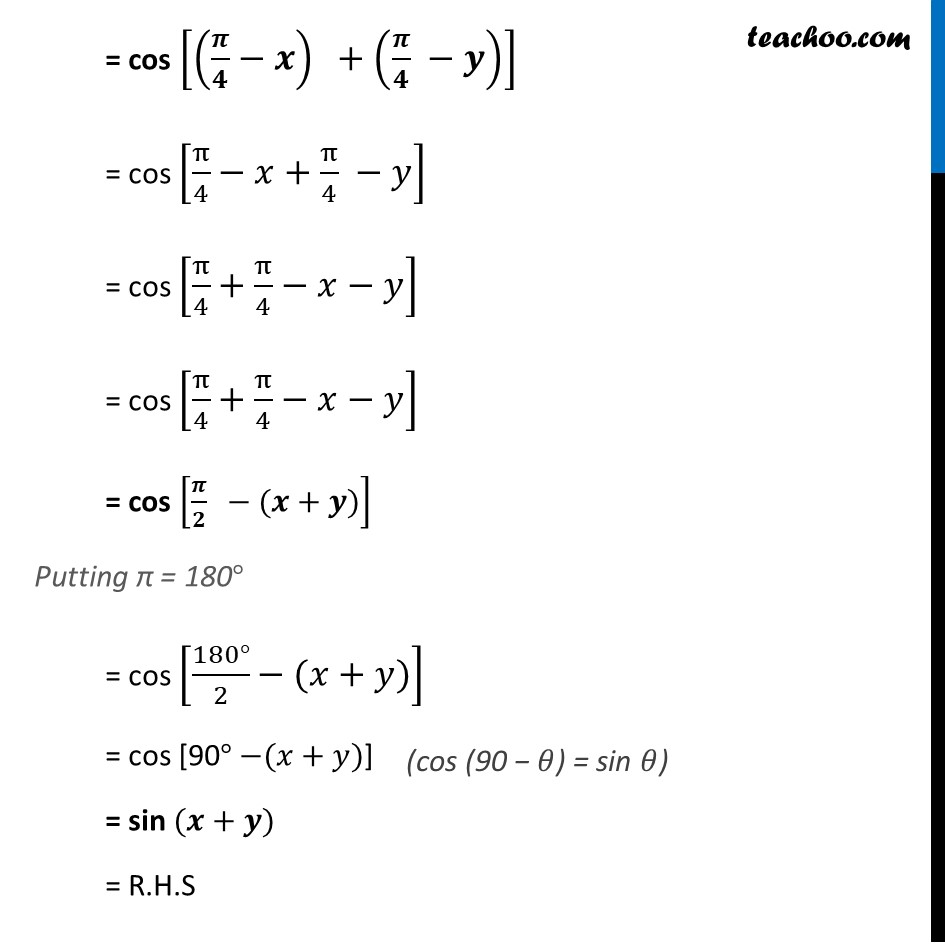
Ex 3.3, 6 Prove that: cos (π/4−𝑥) cos (π/4−𝑦) – sin (π/4−𝑥) sin (π/4−𝑦) = sin⁡(𝑥 + 𝑦) Taking L.H.S We know that cos (A + B) = cos A cos B – sin A sin B The equation given in Question is of this form Where A = (𝜋/4 −𝑥) B = (𝜋/4 −𝑦) Hence cos (π/4−𝑥) cos (π/4−𝑦) – sin (π/4−𝑥) sin (π/4−𝑦) = cos [(π/4−𝑥)" " +(π/4 −𝑦)] = cos [π/4−𝑥+π/4 −𝑦] = cos [π/4+π/4−𝑥−𝑦] = cos [π/4+π/4−𝑥−𝑦] = cos [π/2 " " −(𝑥+𝑦)] Putting π = 180° = cos [(180°)/2−(𝑥+𝑦)] = cos [90° −(𝑥+𝑦)] = sin (𝑥+𝑦) = R.H.S Hence proved

Ex 3.3

Ex 3.3, 6 Important You are here

Ex 3.4 →

Chapter 3 Class 11 Trigonometric Functions

Serial order wise

About the Author

Davneet Singh

Davneet Singh is a graduate from Indian Institute of Technology, Kanpur. He has been teaching from the past 9 years. He provides courses for Maths and Science at Teachoo.