Ex 12.1, 24 - Chapter 12 Class 11 Limits and Derivatives

Transcript

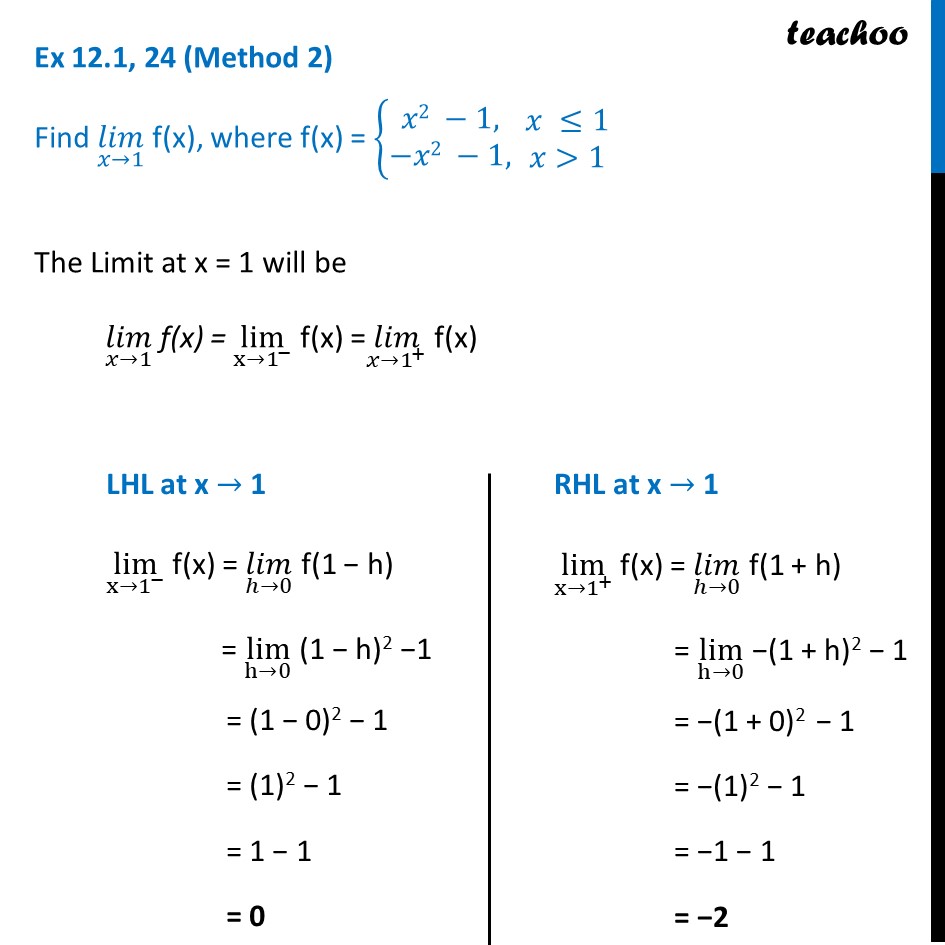
Ex 12.1, 24 (Method 1) Find (𝑙𝑖𝑚)┬(𝑥→1) f(x), where f(x) = {█(𝑥2 −1,@−𝑥2 −1,)┤ ■8(𝑥 ≤1@𝑥>1) The Limit at x = 1 will be (𝑙𝑖𝑚)┬(𝑥→1) f(x) = lim┬(〖x→1〗^− ) f(x) =(𝑙𝑖𝑚)┬(〖𝑥→1〗^+ ) f(x) (𝒍𝒊𝒎)┬(〖𝒙→𝟏〗^− ) f(x) = (𝑙𝑖𝑚)┬(𝑥→1) x2 – 1 = (1)2 – 1 = 1 – 1 = 0 (𝒍𝒊𝒎)┬(〖𝒙→𝟏〗^+ ) f(x) = (𝑙𝑖𝑚)┬(𝑥→1) (–x2 – 1) = –(1)2 – 1 = –1 – 1 = –2 Thus, (𝒍𝒊𝒎)┬(〖𝒙→𝟏〗^+ )f(x) ≠ (𝒍𝒊𝒎)┬(〖𝒙→𝟏〗^− )f(x) Since, Left Hand Limit & Right Hand Limit are not equal Hence (𝐥𝒊𝒎)┬(𝒙→𝟏) f(x) does not exit Ex 12.1, 24 (Method 2) Find (𝑙𝑖𝑚)┬(𝑥→1) f(x), where f(x) = {█(𝑥2 −1,@−𝑥2 −1,)┤ ■8(𝑥 ≤1@𝑥>1) The Limit at x = 1 will be (𝑙𝑖𝑚)┬(𝑥→1) f(x) = lim┬(〖x→1〗^− ) f(x) =(𝑙𝑖𝑚)┬(〖𝑥→1〗^+ ) f(x) LHL at x → 1 lim┬(x→1^− ) f(x) = lim┬(h→0) f(1 − h) = lim┬(h→0) (1 − h)2 −1 = (1 − 0)2 − 1 = (1)2 − 1 = 1 − 1 = 0 RHL at x → 1 lim┬(x→1^+ ) f(x) = lim┬(h→0) f(1 + h) = lim┬(h→0) −(1 + h)2 − 1 = −(1 + 0)2 − 1 = −(1)2 − 1 = −1 − 1 = −2 Since LHL ≠ RHL ∴ (𝒍𝒊𝒎)┬(𝒙→𝟏) f(x) doesn’t exist