Get live Maths 1-on-1 Classs - Class 6 to 12
Ex 13.1, 14 - Chapter 13 Class 11 Limits and Derivatives (Term 1 and Term 2)
Last updated at March 22, 2023 by Teachoo
Ex 13.1
Ex 13.1, 1
Ex 13.1, 2
Ex 13.1, 3
Ex 13.1, 4 Important
Ex 13.1, 5
Ex 13.1, 6 Important
Ex 13.1, 7
Ex 13.1, 8 Important
Ex 13.1, 9
Ex 13.1,10 Important
Ex 13.1, 11
Ex 13.1, 12
Ex 13.1, 13
Ex 13.1, 14 Important You are here
Ex 13.1, 15 Important
Ex 13.1, 16
Ex 13.1, 17 Important
Ex 13.1, 18
Ex 13.1, 19 Important
Ex 13.1, 20
Ex 13.1, 21 Important
Ex 13.1, 22 Important
Ex 13.1, 23
Ex 13.1, 24
Ex 13.1, 25 Important
Ex 13.1, 26
Ex 13.1, 27
Ex 13.1, 28 Important
Ex 13.1, 29
Ex 13.1, 30 Important
Ex 13.1, 31
Ex 13.1, 32 Important
Transcript
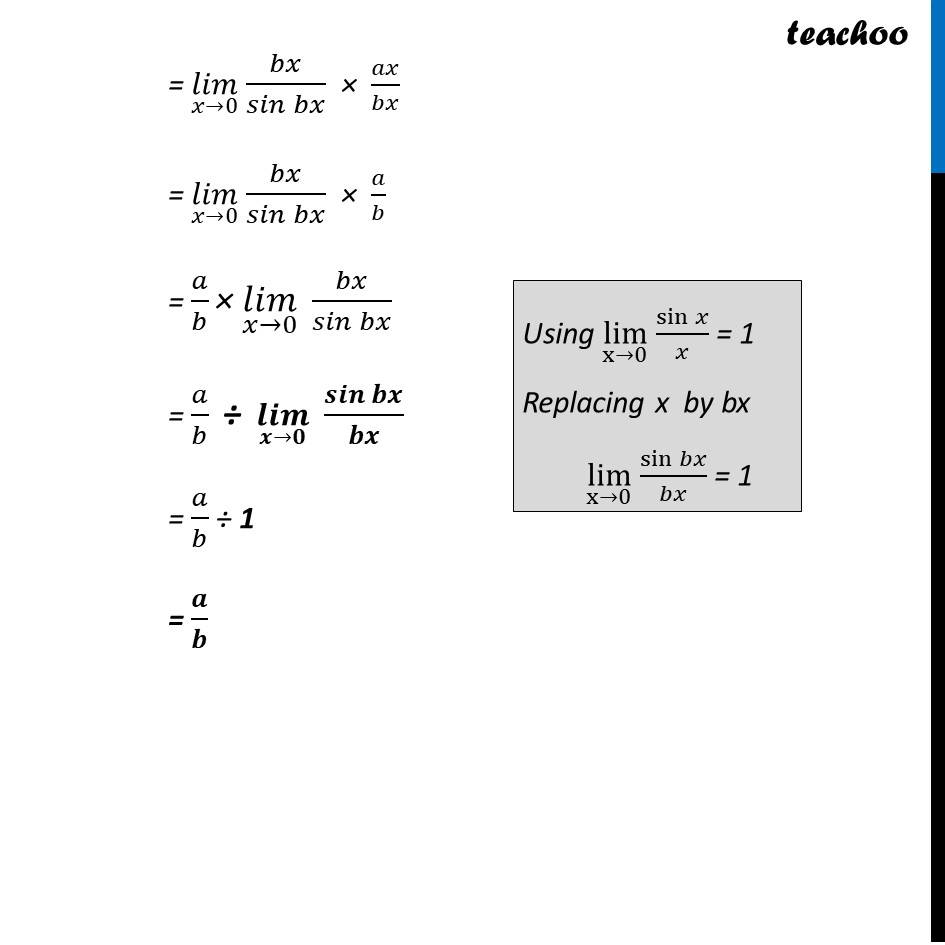
Ex 13.1, 14 Evaluate the Given limit: lim┬(x→0) sin〖ax 〗/(sin bx), a, b ≠ 0 (𝑙𝑖𝑚)┬(𝑥→0) 𝑠𝑖𝑛〖𝑎𝑥 〗/(𝑠𝑖𝑛 𝑏𝑥) = (𝑙𝑖𝑚)┬(𝑥→0) sin ax × (𝑙𝑖𝑚)┬(𝑥→0) 1/𝑠𝑖𝑛𝑏𝑥 Multiplying & dividing by ax = (𝒍𝒊𝒎)┬(𝒙→𝟎) 𝒔𝒊𝒏𝒂𝒙/𝒂𝒙 × (𝑙𝑖𝑚)┬(𝑥→0) 𝑎𝑥/𝑠𝑖𝑛𝑏𝑥 = 1 × (𝑙𝑖𝑚)┬(𝑥→0) 𝑎𝑥/𝑠𝑖𝑛𝑏𝑥 = (𝑙𝑖𝑚)┬(𝑥→0) 𝑎𝑥/𝑠𝑖𝑛𝑏𝑥 Multiplying & dividing by bx = (𝑙𝑖𝑚)┬(𝑥→0) 𝑎𝑥/𝑠𝑖𝑛𝑏𝑥 × 𝑏𝑥/𝑏𝑥 Using lim┬(x→0) sin𝑥/𝑥 = 1 Replacing x by ax lim┬(x→0) sin𝑎𝑥/𝑎𝑥 = 1 = (𝑙𝑖𝑚)┬(𝑥→0) 𝑏𝑥/𝑠𝑖𝑛𝑏𝑥 × 𝑎𝑥/𝑏𝑥 = (𝑙𝑖𝑚)┬(𝑥→0) 𝑏𝑥/𝑠𝑖𝑛𝑏𝑥 × 𝑎/𝑏 = 𝑎/𝑏 "×" (𝑙𝑖𝑚)┬(𝑥→0) 𝑏𝑥/𝑠𝑖𝑛𝑏𝑥 = 𝑎/𝑏 ÷ (𝒍𝒊𝒎)┬(𝒙→𝟎) 𝒔𝒊𝒏𝒃𝒙/𝒃𝒙 = 𝑎/𝑏 ÷ 1 = 𝒂/𝒃 Using lim┬(x→0) sin𝑥/𝑥 = 1 Replacing x by bx lim┬(x→0) sin𝑏𝑥/𝑏𝑥 = 1
