

Examples
Last updated at Dec. 16, 2024 by Teachoo



Transcript
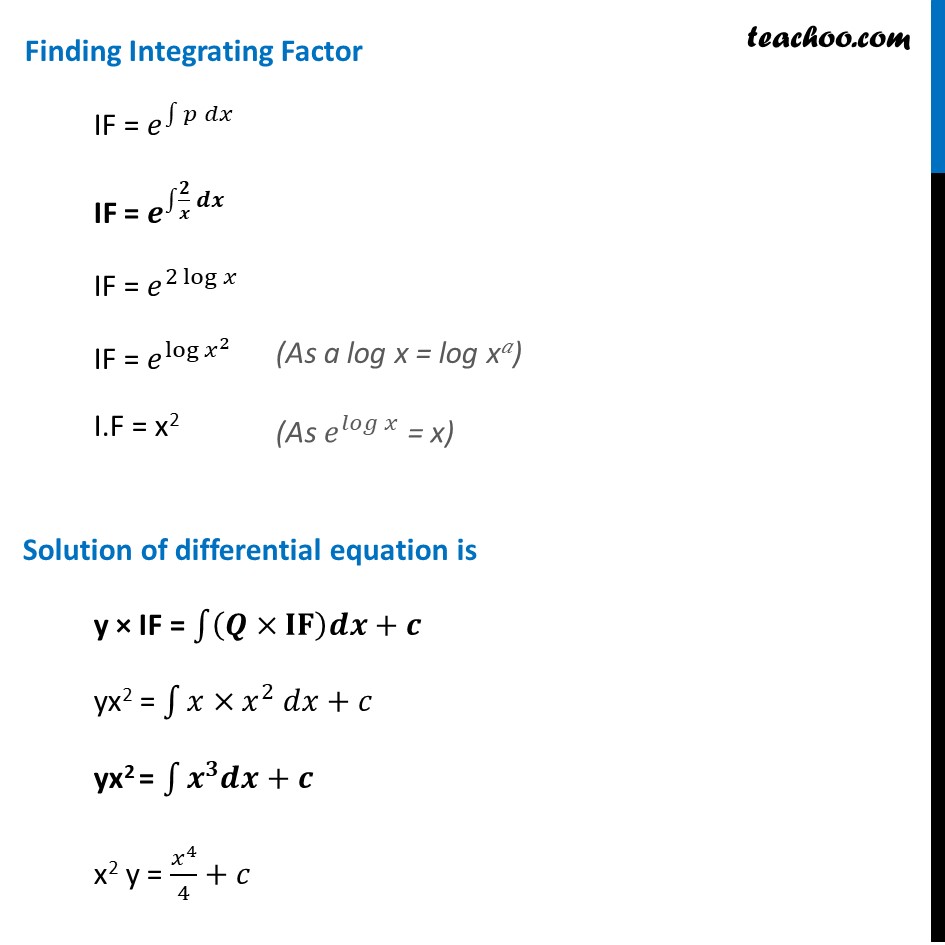
Example 15 Find the general solution of the differential equation 𝑥 𝑑𝑦/𝑑𝑥+2𝑦=𝑥^2 (𝑥≠0) 𝑥 𝑑𝑦/𝑑𝑥+2𝑦=𝑥^2 (𝑥 𝑑𝑦)/(𝑥 𝑑𝑥) + 2𝑦/𝑥 = 𝑥^2/𝑥 Dividing both sides by x 𝒅𝒚/𝒅𝒙 + 𝟐𝒚/𝒙 = x Differential equation is of the form 𝑑𝑦/𝑑𝑥+𝑃𝑦=𝑄 where P = 2/𝑥 & Q = x Finding Integrating Factor IF = 𝑒^∫1▒〖𝑝 𝑑𝑥〗 IF = 𝒆^∫1▒〖𝟐/𝒙 𝒅𝒙〗 IF = 𝑒^(2 log𝑥 ) IF = 𝑒^(〖log𝑥〗^2 ) I.F = x2 Solution of differential equation is y × IF = ∫1▒〖(𝑸×𝐈𝐅)𝒅𝒙+𝒄〗 yx2 = ∫1▒〖𝑥×𝑥^2 𝑑𝑥+𝑐〗 yx2 = ∫1▒〖𝒙^𝟑 𝒅𝒙+𝒄〗 x2 y = 𝑥^4/4+𝑐 y = 𝒙^𝟐/𝟒+𝒄𝒙^(−𝟐) is the required general solution