Misc 19 - Chapter 5 Class 12 Continuity and Differentiability

Transcript

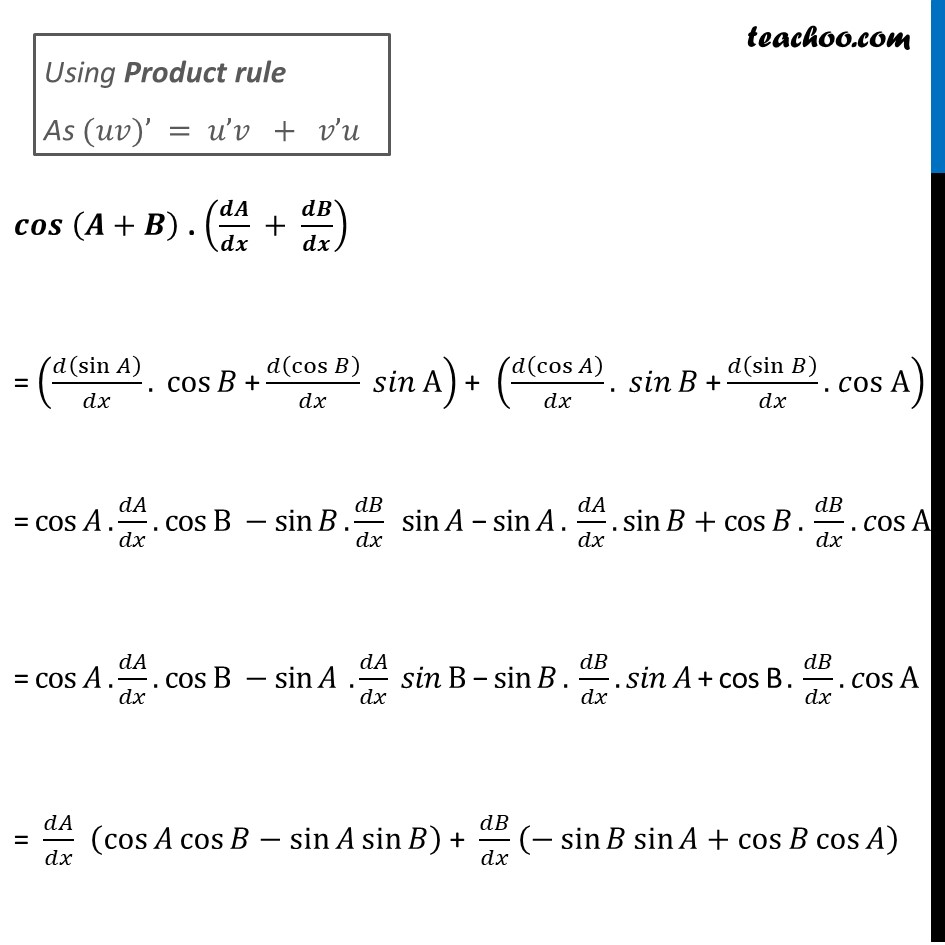
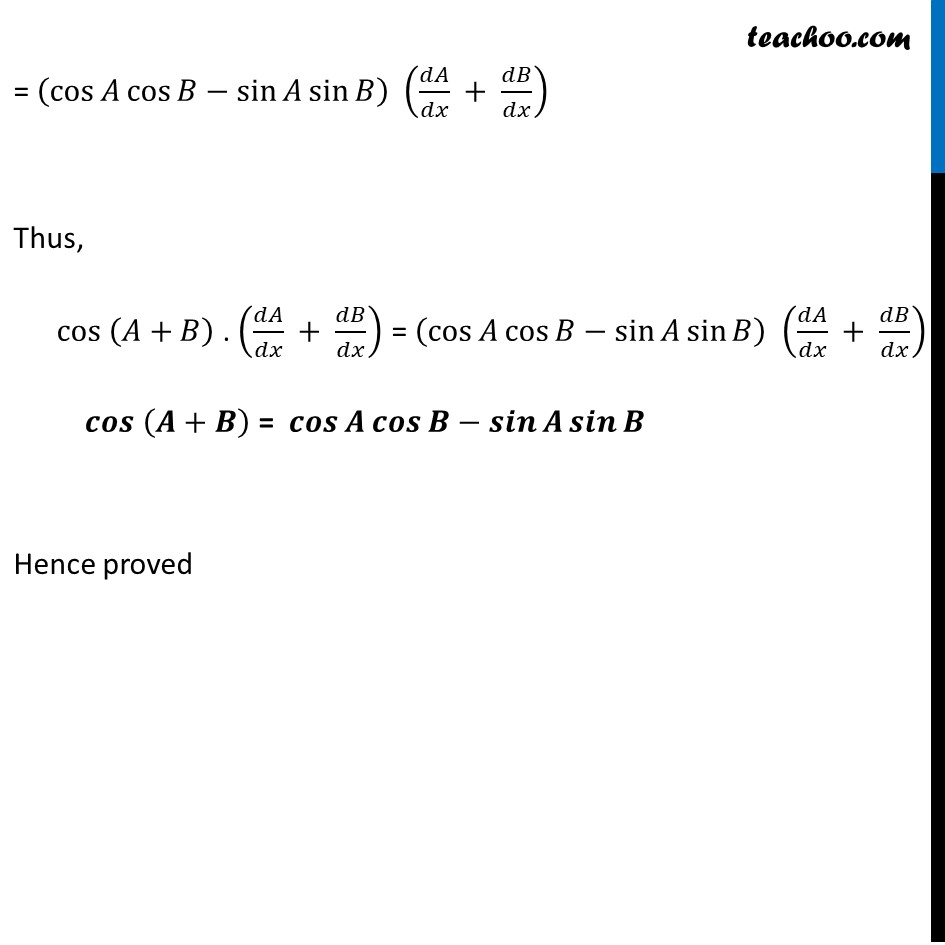
Misc 19 Using the fact that sin⁡(𝐴 + 𝐵)=sin⁡𝐴 cos⁡𝐵+cos⁡𝐴 sin⁡𝐵 and the differentiation, obtain the sum formula for cosines.Given sin⁡(𝐴 + 𝐵)=sin⁡𝐴 cos⁡𝐵+cos⁡𝐴 sin⁡𝐵 Consider A & B are function of 𝑥 Differentiating both side 𝑤.𝑟.𝑡.𝑥. 𝑑(sin⁡(𝐴 + 𝐵) )/𝑑𝑥 = 𝑑(sin⁡𝐴 cos⁡𝐵 + cos⁡𝐴 sin⁡𝐵)/𝑑𝑥 𝑑(sin⁡(𝐴 + 𝐵) )/𝑑𝑥 = 𝑑(sin⁡𝐴 . cos⁡𝐵)/𝑑𝑥 + 𝑑(cos⁡〖𝐴 〗. sin⁡𝐵)/𝑑𝑥 cos (𝐴+𝐵) . 𝑑(𝐴 + 𝐵)/𝑑𝑥 = 𝑑(sin⁡𝐴 . cos⁡𝐵)/𝑑𝑥 + 𝑑(cos⁡〖𝐴 〗. sin⁡𝐵)/𝑑𝑥 𝒄𝒐𝒔 (𝑨+𝑩) . (𝒅𝑨/𝒅𝒙 + 𝒅𝑩/𝒅𝒙) = (𝑑(sin⁡𝐴 )/𝑑𝑥. cos⁡𝐵" +" 𝑑(cos⁡𝐵 )/𝑑𝑥 " " 𝑠𝑖𝑛⁡"A" ) + (𝑑(cos⁡𝐴 )/𝑑𝑥. 𝑠𝑖𝑛⁡𝐵" +" 𝑑(sin⁡𝐵 )/𝑑𝑥 ". " 𝑐"os A" ) = cos⁡𝐴.𝑑𝐴/𝑑𝑥 ". cos B "−sin⁡𝐵.𝑑𝐵/𝑑𝑥 " " sin⁡𝐴 − sin⁡𝐴. 𝑑𝐴/𝑑𝑥.sin⁡𝐵+cos⁡𝐵. 𝑑𝐵/𝑑𝑥 ". " 𝑐"os A" = cos⁡𝐴.𝑑𝐴/𝑑𝑥 ". cos B "−sin⁡𝐴 .𝑑𝐴/𝑑𝑥 " " 𝑠𝑖𝑛⁡"B" − sin⁡𝐵. 𝑑𝐵/𝑑𝑥. 𝑠𝑖𝑛 𝐴⁡"+ cos B" . 𝑑𝐵/𝑑𝑥 ". " 𝑐"os A" = 𝑑𝐴/𝑑𝑥 (cos⁡𝐴 cos⁡𝐵−sin⁡𝐴 sin⁡𝐵 ) + 𝑑𝐵/𝑑𝑥 (−sin⁡𝐵 sin⁡𝐴+cos⁡𝐵 cos⁡𝐴 ) = (cos⁡𝐴 cos⁡𝐵−sin⁡𝐴 sin⁡𝐵 ) (𝑑𝐴/𝑑𝑥 + 𝑑𝐵/𝑑𝑥) Thus, cos (𝐴+𝐵) . (𝑑𝐴/𝑑𝑥 + 𝑑𝐵/𝑑𝑥) = (cos⁡𝐴 cos⁡𝐵−sin⁡𝐴 sin⁡𝐵 ) (𝑑𝐴/𝑑𝑥 + 𝑑𝐵/𝑑𝑥) 𝒄𝒐𝒔" " (𝑨+𝑩) = 𝒄𝒐𝒔⁡𝑨 𝒄𝒐𝒔⁡𝑩−𝒔𝒊𝒏⁡𝑨 𝒔𝒊𝒏⁡𝑩 Hence proved