Ex 7.10, 4 - Chapter 7 Class 12 Integrals (Term 2)

Transcript

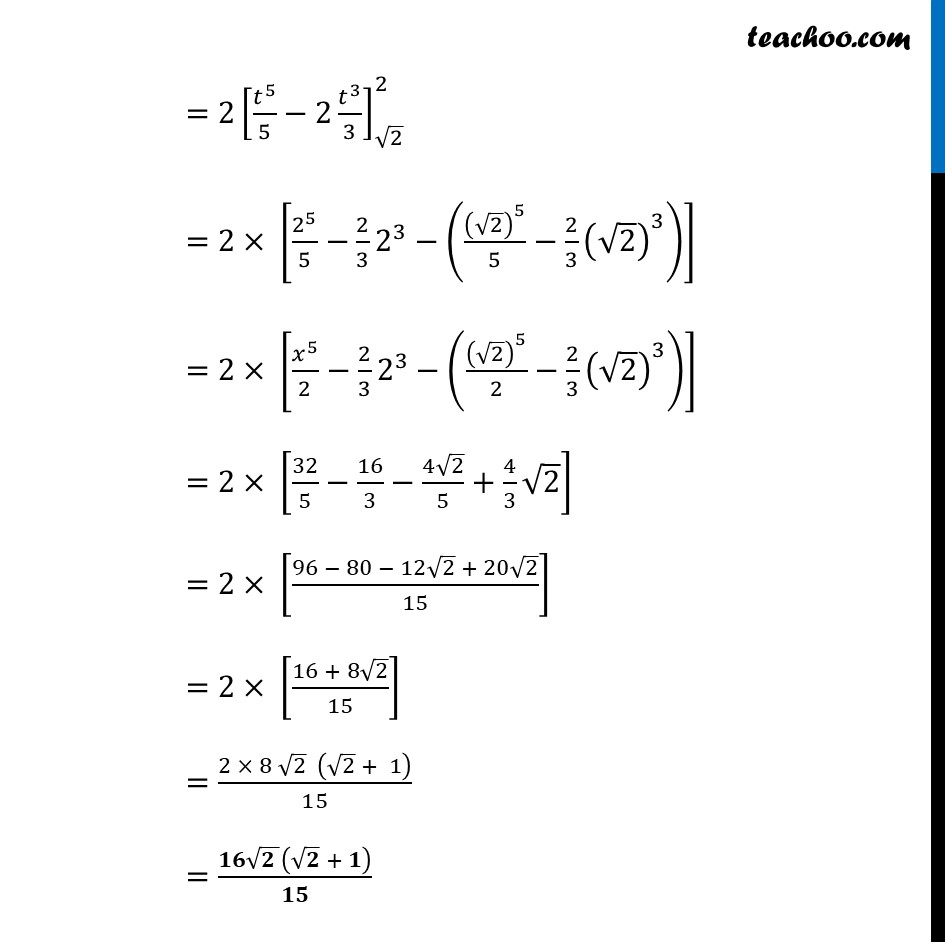
Ex 7.10, 4 Evaluate the integrals using substitution 0﷮2﷮ 𝑥 ﷮𝑥+2﷯﷮ 𝑝𝑢𝑡 𝑥+2= 𝑡﷮2﷯﷯﷯﷯ 0﷮2﷮ 𝑥 ﷮𝑥+2﷯﷮ 𝑑𝑥﷯﷯ Put 𝑥+2= 𝑡﷮2﷯ Differentiating w.r.t. 𝑥 𝑑 𝑥 + 2﷯﷮𝑑𝑥﷯= 𝑑 𝑡﷮2﷯﷯﷮𝑑𝑡﷯ × 𝑑𝑡﷮𝑑𝑥﷯ 1=2𝑡 × 𝑑𝑡﷮𝑑𝑥﷯ 𝑑𝑥=2𝑡 𝑑𝑡 Hence, when 𝑥 varies from 0 to 2, then t varies from ﷮2﷯ to 2 Therefore we can write 0﷮2﷮𝑥 ﷮𝑥+2﷯ 𝑑𝑥 = ﷮2﷯﷮2﷮ 𝑡﷮2﷯−2﷯ ﷮ 𝑡﷮2﷯﷯ 2𝑡 𝑑𝑡﷯﷯ = ﷮2﷯﷮2﷮ 𝑡﷮2﷯−2﷯𝑡 ×2𝑡 𝑑𝑡﷯ =2 ﷮2﷯﷮2﷮ 𝑡﷮2﷯−2﷯ 𝑡﷮2﷯ 𝑑𝑡﷯ =2 ﷮2﷯﷮2﷮ 𝑡﷮4﷯−2 𝑡﷮2﷯﷯ 𝑑𝑡﷯ =2 𝑡﷮4+1﷯﷮4+1﷯−2 𝑡﷮2+1﷯﷮2+1﷯﷯﷮ ﷮2﷯﷮2﷯ =2 𝑡﷮5﷯﷮5﷯−2 𝑡﷮3﷯﷮3﷯﷯﷮ ﷮2﷯﷮2﷯ =2× 2﷮5﷯﷮5﷯− 2﷮3﷯ 2﷮3﷯− ﷮2﷯﷯﷮5﷯﷮5﷯− 2﷮3﷯ ﷮2﷯﷯﷮3﷯﷯﷯ =2× 𝑥﷮5﷯﷮2﷯− 2﷮3﷯ 2﷮3﷯− ﷮2﷯﷯﷮5﷯﷮2﷯− 2﷮3﷯ ﷮2﷯﷯﷮3﷯﷯﷯ =2× 32﷮5﷯− 16﷮3﷯− 4 ﷮2﷯﷮5﷯+ 4﷮3﷯ ﷮2﷯﷯ =2× 96 − 80 − 12 ﷮2﷯ + 20 ﷮2﷯﷮15﷯﷯ =2× 16 + 8 ﷮2﷯﷮15﷯﷯ = 2 × 8 ﷮2﷯ ﷮2﷯ + 1﷯﷮15﷯ = 𝟏𝟔 ﷮𝟐 ﷯ ﷮𝟐﷯ + 𝟏﷯﷮𝟏𝟓﷯