Get live Maths 1-on-1 Classs - Class 6 to 12
Example 7 - Chapter 3 Class 11 Trigonometric Functions (Term 2)
Last updated at March 22, 2023 by Teachoo
Examples
Example 1
Example 2 Important
Example 3
Example 4
Example 5 Important
Example 6 Important
Example 7 Important You are here
Example 8
Example 9 Important
Example 10
Example 11 Important
Example 12
Example 13
Example 14
Example 15
Example 16 Important
Example 17 Important
Example 18
Example 19
Example 20 Deleted for CBSE Board 2023 Exams
Example 21 Deleted for CBSE Board 2023 Exams
Example 22 Important Deleted for CBSE Board 2023 Exams
Example 23 Deleted for CBSE Board 2023 Exams
Example 24 Important Deleted for CBSE Board 2023 Exams
Example 25 Important
Example 26
Example 27 Important
Example 28 Important
Example 29 Important
Chapter 3 Class 11 Trigonometric Functions
Serial order wise
- Ex 3.1
- Ex 3.2
- Ex 3.3
- Ex 3.4
-
Examples
- Miscellaneous
Transcript
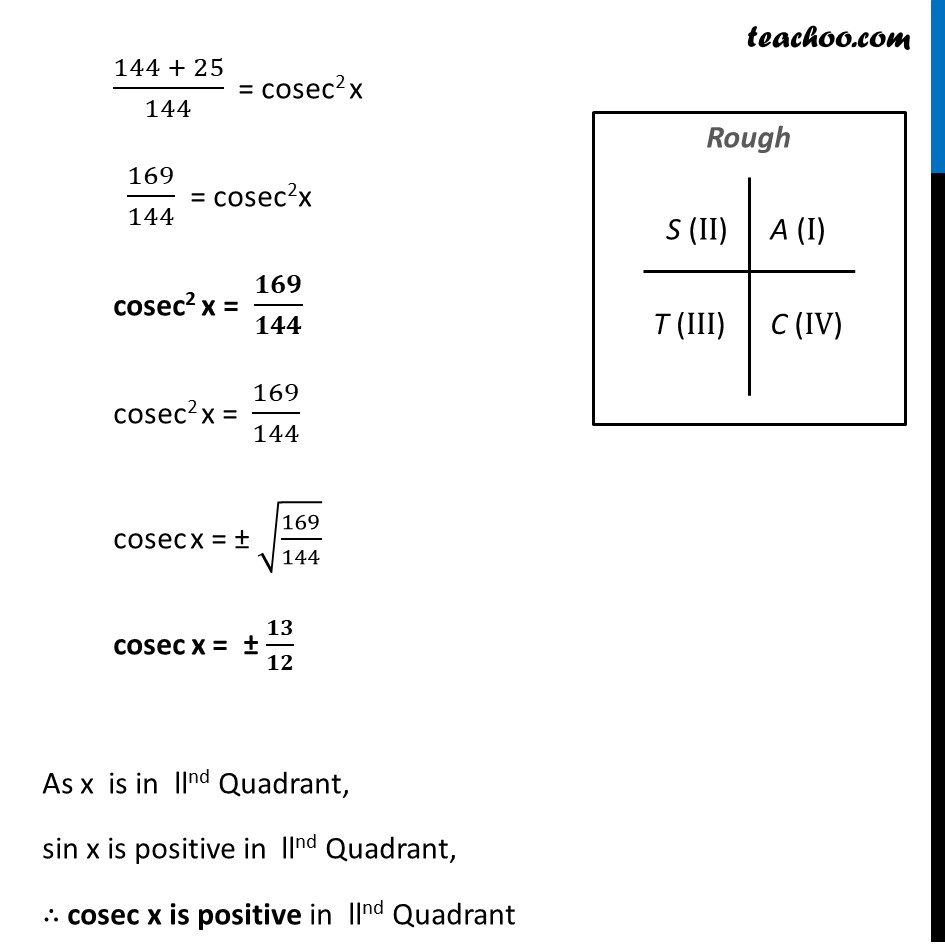
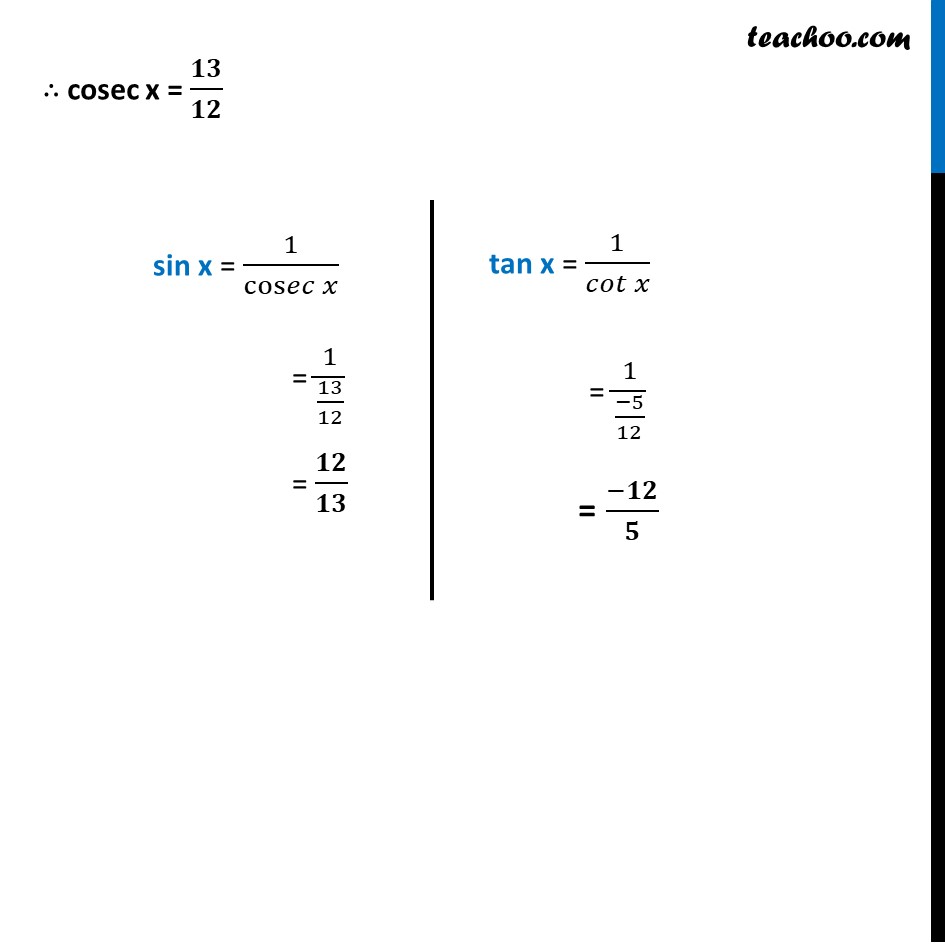
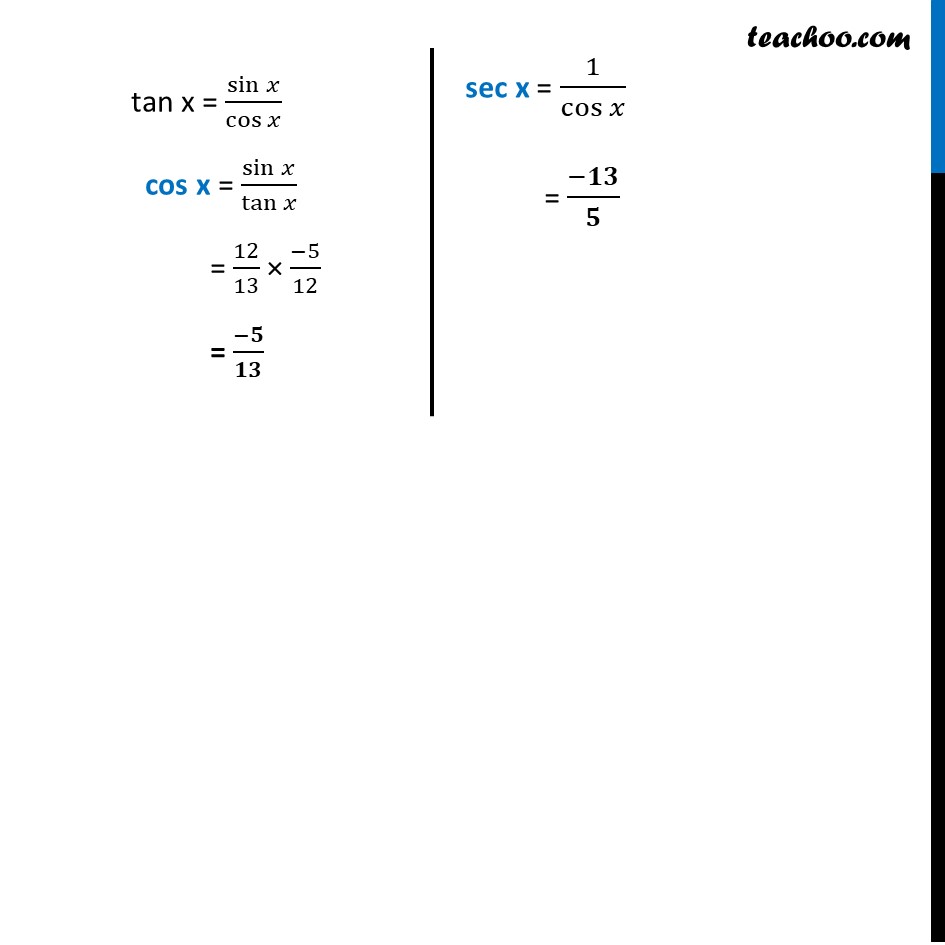
Example 7 If cot𝑥 = – 5/12 , x lies in second quadrant, find the values of other five trigonometric functions. Since x lies in llnd Quadrant Where cos x and tan x will be negative But sin x will be Positive We know that 1 + cot2x = cosec2 x 1 + ((−5)/12)^2 = cosec2 x 1 + 25/144 = cosec2 x (144 + 25)/144 = cosec2 x 169/144 = cosec2x cosec2 x = 169/144 cosec2 x = 169/144 cosec x = ± √(169/144) cosec x = ± 13/12 As x is in llnd Quadrant, sin x is positive in llnd Quadrant, ∴ cosec x is positive in llnd Quadrant ∴ cosec x = 𝟏𝟑/𝟏𝟐 sin x = 1/cos𝑒𝑐𝑥 = 1/(13/12) = 𝟏𝟐/𝟏𝟑 tan x = 1/(𝑐𝑜𝑡 𝑥) = 1/((−5)/12) = (−𝟏𝟐)/𝟓 tan x = sin𝑥/cos𝑥 cos x = sin𝑥/tan𝑥 = 12/13 × (−5)/12 = (−𝟓)/𝟏𝟑
