Ex 4.5, 16 - Chapter 4 Class 12 Determinants (Term 1)

Transcript

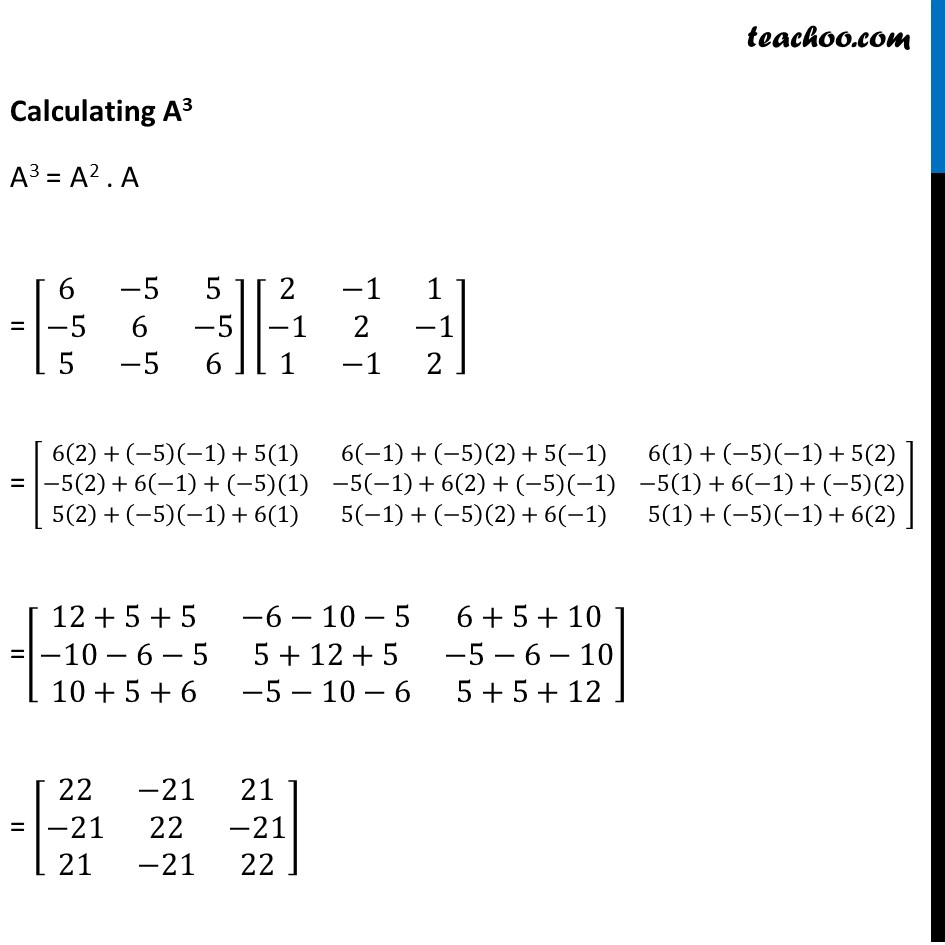
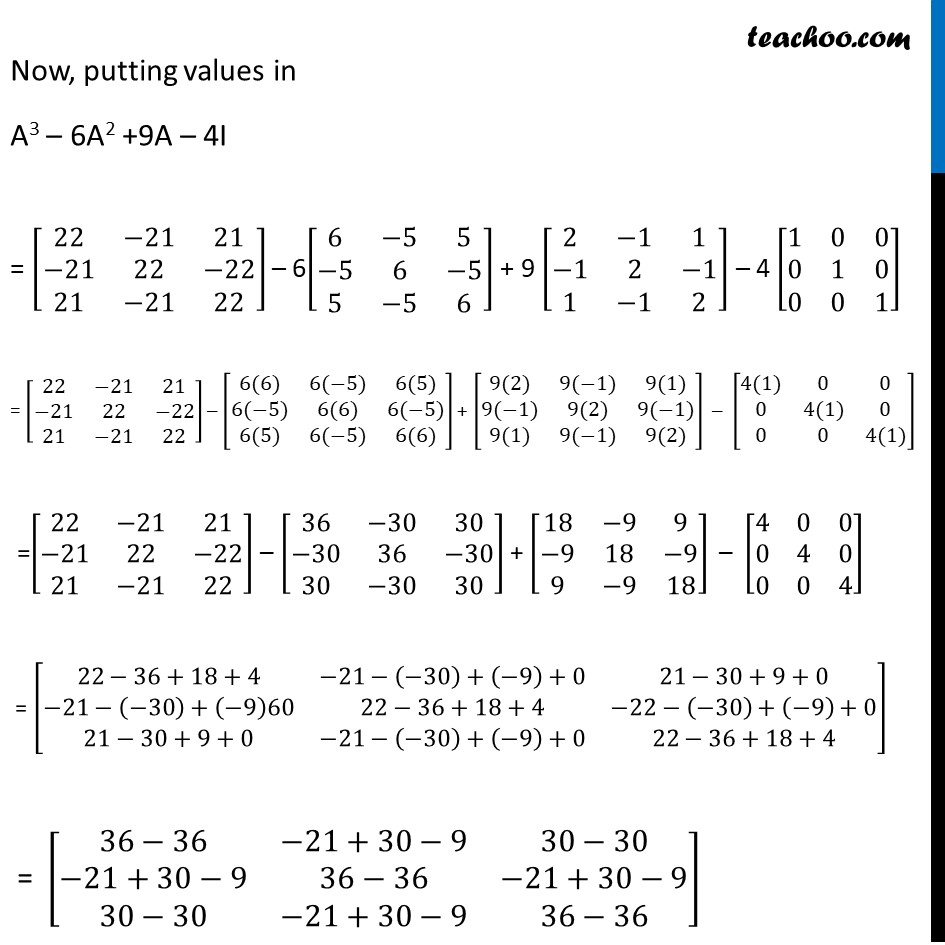
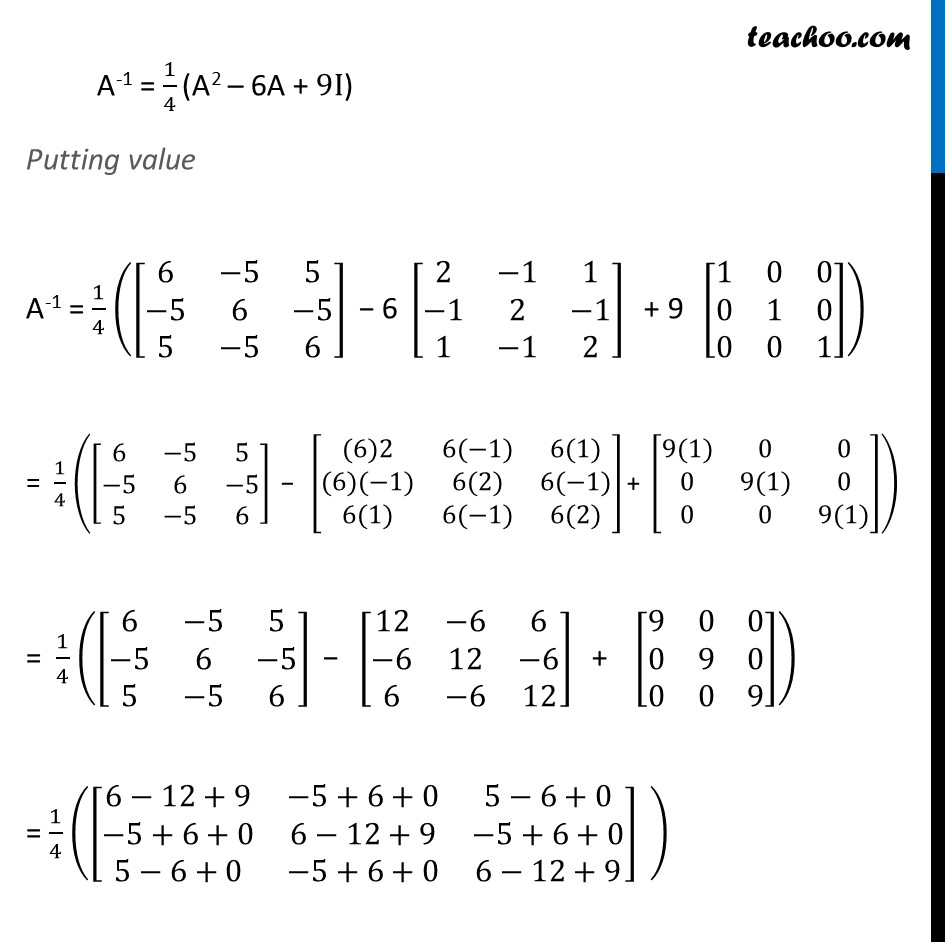
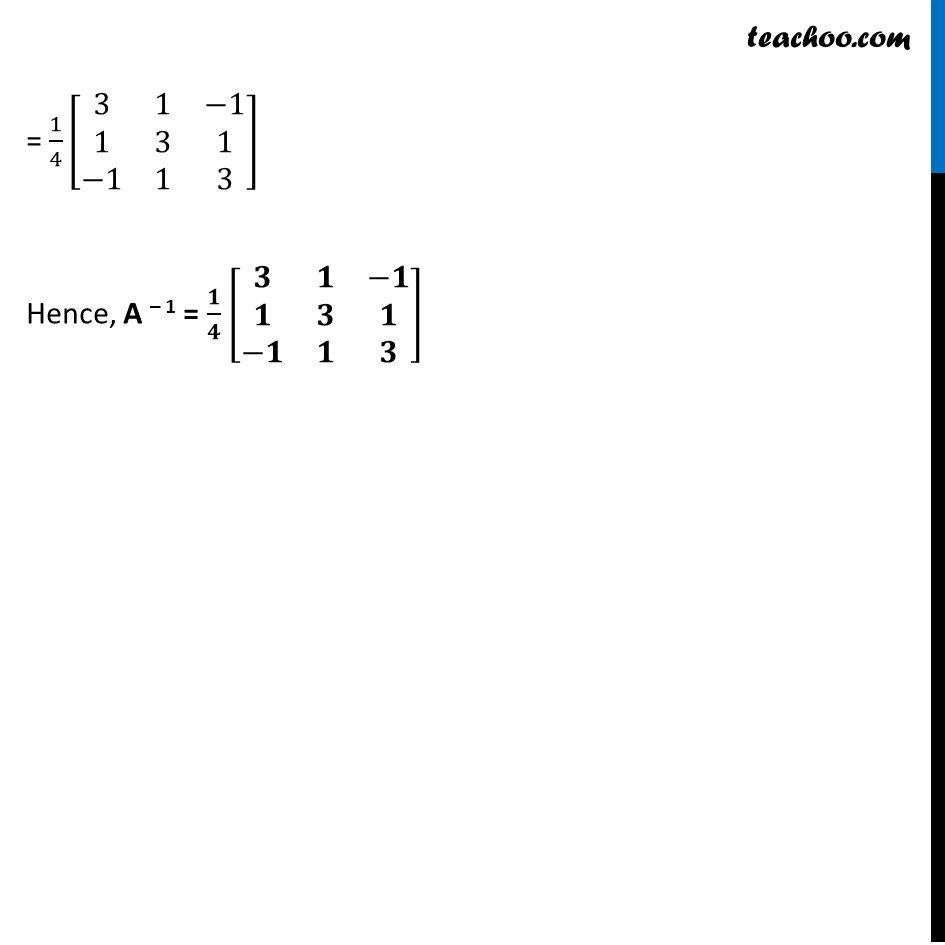
Ex4.5, 16 If A = 2﷮−1﷮1﷮−1﷮2﷮−1﷮1﷮−1﷮2﷯﷯ verify that A3 − 6A2 + 9A − 4I = O and hence find A−1 Calculating A2 A2 = A.A = 2﷮−1﷮1﷮−1﷮2﷮−1﷮1﷮−1﷮2﷯﷯ 2﷮−1﷮1﷮−1﷮2﷮−1﷮1﷮−1﷮2﷯﷯ = 2 2﷯+ −1﷯ −1﷯+1(1)﷮2 −1﷯+ −1﷯ 2﷯+1(−1)﷮2 1﷯+ −1﷯ −1﷯+1(2)﷮−1 2﷯+2 −1﷯+(−1)(1)﷮−1 −1﷯+2 2﷯+(−1)(−1)﷮−1 1﷯+2 −1﷯+(−1)(2)﷮1 2﷯+ −1﷯ −1﷯+2(1)﷮1 −1﷯+ −1﷯ 2﷯+2(−1)﷮1 1﷯+ −1﷯ −1﷯+2(2)﷯﷯ = 4+1+1﷮−2−2−1﷮2+1+2﷮−2−2−1﷮1+4+1﷮−1−2−2﷮2+1+2﷮−1−2−2﷮1+1+4﷯﷯ = 6﷮−5﷮5﷮−5﷮6﷮−5﷮5﷮−5﷮6﷯﷯ Hence A2 = 6﷮−5﷮5﷮−5﷮6﷮−5﷮5﷮−5﷮6﷯﷯ Calculating A3 A3 = A2 . A = 6﷮−5﷮5﷮−5﷮6﷮−5﷮5﷮−5﷮6﷯﷯ 2﷮−1﷮1﷮−1﷮2﷮−1﷮1﷮−1﷮2﷯﷯ = 6 2﷯+ −5﷯ −1﷯+5(1)﷮6 −1﷯+ −5﷯ 2﷯+5(−1)﷮6 1﷯+ −5﷯ −1﷯+5(2)﷮−5 2﷯+6 −1﷯+(−5)(1)﷮−5 −1﷯+6 2﷯+(−5)(−1)﷮−5 1﷯+6 −1﷯+(−5)(2)﷮5 2﷯+ −5﷯ −1﷯+6(1)﷮5 −1﷯+ −5﷯ 2﷯+6(−1)﷮5 1﷯+ −5﷯ −1﷯+6(2)﷯﷯ = 12+5+5﷮−6−10−5﷮6+5+10﷮−10−6−5﷮5+12+5﷮−5−6−10﷮10+5+6﷮−5−10−6﷮5+5+12﷯﷯= 22﷮−21﷮21﷮−21﷮22﷮−21﷮21﷮−21﷮22﷯﷯ Hence, A3 = 22﷮−21﷮21﷮−21﷮22﷮−21﷮21﷮−21﷮22﷯﷯ Now, putting values in A3 – 6A2 +9A – 4I = 22﷮−21﷮21﷮−21﷮22﷮−22﷮21﷮−21﷮22﷯﷯ – 6 6﷮−5﷮5﷮−5﷮6﷮−5﷮5﷮−5﷮6﷯﷯ + 9 2﷮−1﷮1﷮−1﷮2﷮−1﷮1﷮−1﷮2﷯﷯ – 4 1﷮0﷮0﷮0﷮1﷮0﷮0﷮0﷮1﷯﷯ = 22﷮−21﷮21﷮−21﷮22﷮−22﷮21﷮−21﷮22﷯﷯ – 6(6)﷮6(−5)﷮6(5)﷮6(−5)﷮6(6)﷮6(−5)﷮6(5)﷮6(−5)﷮6(6)﷯﷯ + 9(2)﷮9(−1)﷮9(1)﷮9(−1)﷮9(2)﷮9(−1)﷮9(1)﷮9(−1)﷮9(2)﷯﷯ – 4(1)﷮0﷮0﷮0﷮4(1)﷮0﷮0﷮0﷮4(1)﷯﷯ = 22﷮−21﷮21﷮−21﷮22﷮−22﷮21﷮−21﷮22﷯﷯ – 36﷮−30﷮30﷮−30﷮36﷮−30﷮30﷮−30﷮30﷯﷯ + 18﷮−9﷮9﷮−9﷮18﷮−9﷮9﷮−9﷮18﷯﷯ – 4﷮0﷮0﷮0﷮4﷮0﷮0﷮0﷮4﷯﷯ = 22−36+18+4﷮−21− −30﷯+ −9﷯+0﷮21−30+9+0﷮−21− −30﷯+ −9﷯60﷮22−36+18+4﷮−22− −30﷯+ −9﷯+0﷮21−30+9+0﷮−21− −30﷯+ −9﷯+0﷮22−36+18+4﷯﷯ = 36−36﷮−21+30−9﷮30−30﷮−21+30−9﷮36−36﷮−21+30−9﷮30−30﷮−21+30−9﷮36−36﷯﷯ = 0﷮0﷮0﷮0﷮0﷮0﷮0﷮0﷮0﷯﷯ = O Hence proved Now calculating A-1 using A3 – 6A2 + 9A – 4I = 0 Post multiplying by A-1 both side (A3 – 6A2 + 9A – 4I ) A-1 = 0.A-1 A3 . A-1 – 6A2 . A + 9AA-1 – 4IA-1 = 0 A2 . AA-1 – 6A. A-1 A + 9AA-1 – 4IA-1 = 0 A2 I – 6AI + 9I – 4IA-1 = 0 A2 – 6A + 9I – 4A-1 = 0 4A-1 = A2 – 6A + 9I A-1 = 1﷮4﷯ (A2 – 6A + 9I) Putting value A-1 = 1﷮4﷯ 6﷮−5﷮5﷮−5﷮6﷮−5﷮5﷮−5﷮6﷯﷯ − 6 2﷮−1﷮1﷮−1﷮2﷮−1﷮1﷮−1﷮2﷯﷯ + 9 1﷮0﷮0﷮0﷮1﷮0﷮0﷮0﷮1﷯﷯﷯ = 1﷮4﷯ 6﷮−5﷮5﷮−5﷮6﷮−5﷮5﷮−5﷮6﷯﷯ − (6)2﷮6(−1)﷮6(1)﷮(6)(−1)﷮6(2)﷮6(−1)﷮6(1)﷮6(−1)﷮6(2)﷯﷯+ 9(1)﷮0﷮0﷮0﷮9(1)﷮0﷮0﷮0﷮9(1)﷯﷯﷯ = 1﷮4﷯ 6﷮−5﷮5﷮−5﷮6﷮−5﷮5﷮−5﷮6﷯﷯ − 12﷮−6﷮6﷮−6﷮12﷮−6﷮6﷮−6﷮12﷯﷯ + 9﷮0﷮0﷮0﷮9﷮0﷮0﷮0﷮9﷯﷯﷯ = 1﷮4﷯ 6−12+9﷮−5+6+0﷮5−6+0﷮−5+6+0﷮6−12+9﷮−5+6+0﷮5−6+0﷮−5+6+0﷮6−12+9﷯﷯ ﷯ = 1﷮4﷯ 3﷮1﷮−1﷮1﷮3﷮1﷮−1﷮1﷮3﷯﷯ Hence, A – 1 = 𝟏﷮𝟒﷯ 𝟑﷮𝟏﷮−𝟏﷮𝟏﷮𝟑﷮𝟏﷮−𝟏﷮𝟏﷮𝟑﷯﷯