![Ex 4.5, 3 - Verify A (adj A) = (adj A) A = AI [2 3 -4 -6]](https://d1avenlh0i1xmr.cloudfront.net/ff540729-a8db-42e8-bf4c-8f511bfbd3e3/slide7.jpg)
Ex 4.5, 3 - Chapter 4 Class 12 Determinants (Term 1)
Last updated at Jan. 22, 2020 by Teachoo
Ex 4.5
Ex 4.5, 1
Ex 4.5, 2
Ex 4.5, 3 Important You are here
Ex 4.5, 4 Important
Ex 4.5, 5
Ex 4.5, 6 Important
Ex 4.5, 7
Ex 4.5, 8
Ex 4.5, 9
Ex 4.5, 10 Important
Ex 4.5, 11 Important
Ex 4.5, 12
Ex 4.5, 13
Ex 4.5, 14 Important
Ex 4.5, 15 Important
Ex 4.5, 16
Ex 4.5, 17 (MCQ) Important
Ex 4.5, 18 (MCQ) Important
Chapter 4 Class 12 Determinants (Term 1)
Serial order wise
Transcript
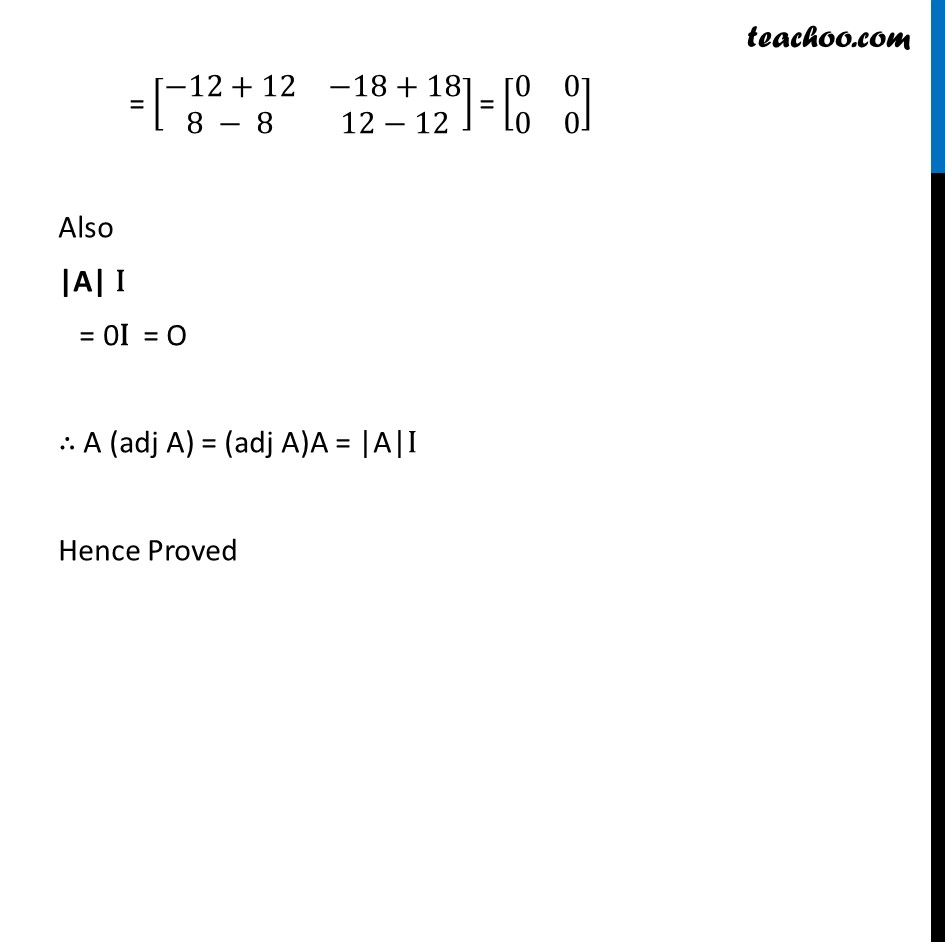
Ex 4.5, 3 Verify A (adj A) = (adj A) A = |𝐴| I, where A = [■8(2&3@−4&−6)] Let A = [■8(2&3@−4&−6)] adj A = [■8(2&3@−4&−6)] = [■8(−6&−3@4&2)] |𝐴| = |■8(2&3@−4&−6)| = 2 × (–6) – 3 × (–4) = –12 + 12 = 0 Calculating A (adj A) = [■8(2&3@−4&−6)] [■8(−6&−3@4&2)] = [■8(2 ×(−6)+3 ×4&2 ×−3+3×2@−4 ×(−6)+(−6) ×4&−4 ×(−3)+(−6) ×2)] = [■8(−12+12&−6+6@+ 24 −24&12−12)] = [■8(0&0@0&0)] Similarly (adj A)A = [■8(−6&−3@4&2)] [■8(2&3@−4&−6)] = [■8(− 6(2)+(−3) (−4)&−6(3)+(−3)(−6)@4(2)+2(−4)&4 (3)+2 (−6))] = [■8(−12+12&−18+18@8 − 8&12−12)] = [■8(0&0@0&0)] Also |A| I = 0I = O ∴ A (adj A) = (adj A)A = |A|I Hence Proved
