Ex 3.2, 21
Last updated at Dec. 8, 2016 by Teachoo
Transcript
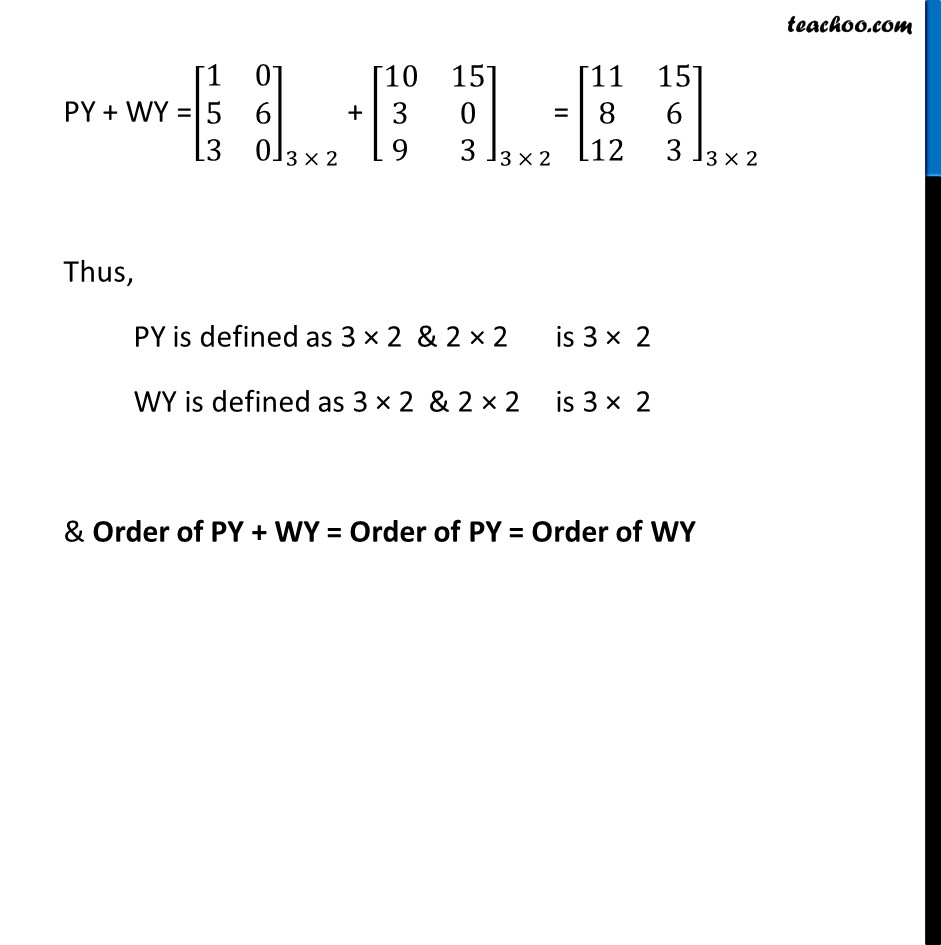
Ex 3.2, 21 (Introduction) Assume X, Y, Z, W and P are matrices of order 2 × n, 3 × k, 2 × p, n × 3 , and p × k respectively. The restriction on n, k and p so that PY +WY will be defined are: (A) k = 3, p = n (B) k is arbitrary, p = 2 (C) p is arbitrary, k = 3 (D) k = 2, p = 3 Ex 3.2, 21 Assume X, Y, Z, W and P are matrices of order 2 × n, 3 × k, 2 × p, n × 3 , and p × k respectively. The restriction on n, k and p so that PY +WY will be defined are: (A) k = 3, p = n (B) k is arbitrary, p = 2 (C) p is arbitrary, k = 3 (D) k = 2, p = 3 Order of P is p × k Order of Y is 3 × k PY = [P]_(p × k) [Y]_(3 × 𝑘) This is possible only if k = 3 So, PY_(𝑝 × 𝑘) Now, PY_(𝑝 × 𝑘) + WY_(𝑛 × 𝑘) is possible if p × k = n × k p = n Thus p = n and k = 3 Hence, correct answer is A
Ex 3.2
About the Author
Davneet Singh
Davneet Singh is a graduate from Indian Institute of Technology, Kanpur. He has been teaching from the past 7 years. He provides courses for Mathematics and Science from Class 6 to 12. You can learn personally from here https://www.teachoo.com/premium/maths-and-science-classes/.
-
A trust fund has Rs 30,000 that must be invested in two different types of bonds. The first bond pays 5% interest per year, and the second bond pays 7% interest per year. Using matrix multiplication, determine how to divide Rs 30,000 among the two types of bonds. If the trust fund must obtain an annual total interest of:
A) Rs 1800.
B) Rs 2000