![Ex 3.2, 17 - Find k so that A2 = kA - 2I, if A = [3 -2 4 -2]](https://d1avenlh0i1xmr.cloudfront.net/45271a36-43cc-4b92-9292-8fda58fff6c9/slide58.jpg)
Now learn Economics at Teachoo for Class 12
Ex 3.2, 17 - Chapter 3 Class 12 Matrices (Term 1)
Last updated at Jan. 17, 2020 by Teachoo
Ex 3.2
Ex 3.2, 1
Ex 3.2, 2 (i)
Ex 3.2, 2 (ii) Important
Ex 3.2, 2 (iii)
Ex 3.2, 2 (iv)
Ex 3.2, 3 (i)
Ex 3.2, 3 (ii) Important
Ex 3.2, 3 (iii)
Ex 3.2, 3 (iv) Important
Ex 3.2, 3 (v)
Ex 3.2, 3 (vi) Important
Ex 3.2, 4
Ex 3.2, 5
Ex 3.2, 6
Ex 3.2, 7 (i)
Ex 3.2, 7 (ii) Important
Ex 3.2, 8
Ex 3.2, 9
Ex 3.2, 10
Ex 3.2, 11
Ex 3.2, 12 Important
Ex 3.2, 13 Important
Ex 3.2, 14
Ex 3.2, 15
Ex 3.2, 16 Important
Ex 3.2, 17 Important You are here
Ex 3.2, 18
Ex 3.2, 19 Important
Ex 3.2, 20 Important
Ex 3.2, 21 (MCQ) Important
Ex 3.2, 22 (MCQ) Important
Chapter 3 Class 12 Matrices (Term 1)
Serial order wise
Transcript
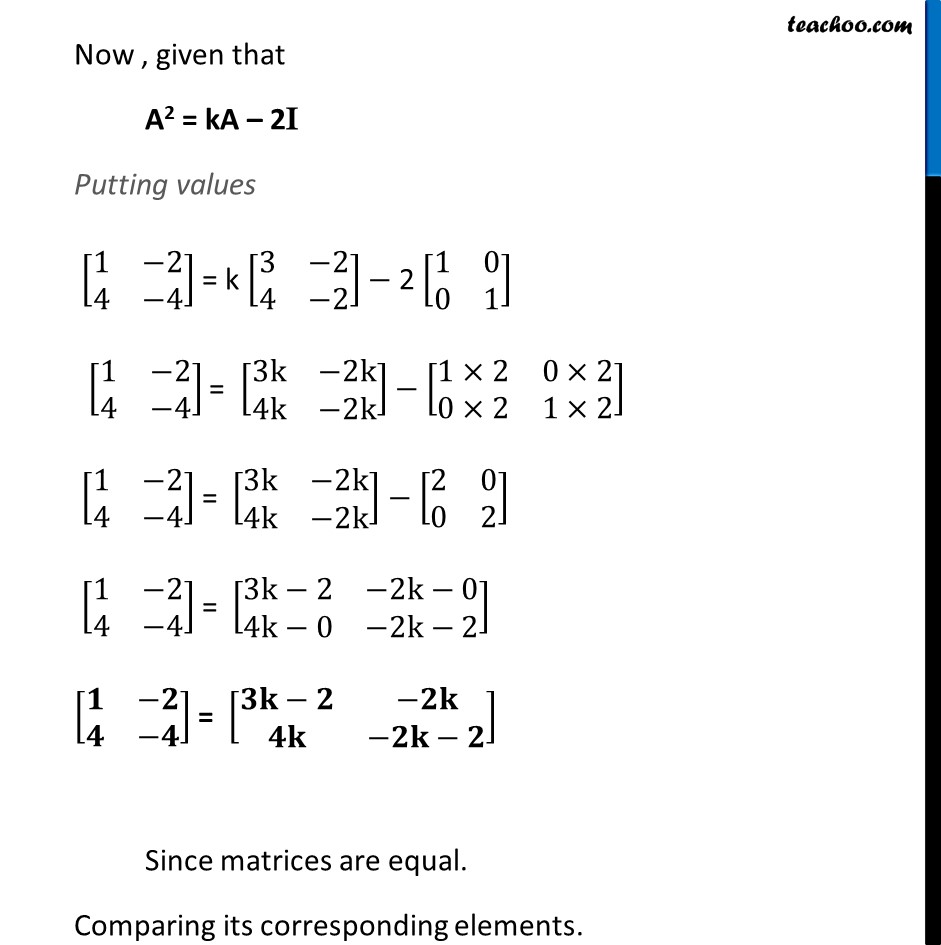
Ex 3.2, 17 If A = [■8(3&−2@4&−2)] and I= [■8(1&0@0&1)] , find k so that A2 = kA – 2I Finding A2 A2 = A × A = [■8(3&−2@4&−2)][■8(3&−2@4&−2)] = [■8(3(3)+(−2)(4)&3(−2)+(−2)(−2)@4(3)+(−2)(4)&4(−2)+(−2)(−2))] = [■8(9−8&−6+4@12−8&−8+4)] = [■8(1&−2@4&−4)] ∴ A2 = [■8(1&−2@4&−4)] Now , given that A2 = kA – 2I Putting values [■8(1&−2@4&−4)] = k [■8(3&−2@4&−2)] − 2 [■8(1&0@0&1)] [■8(1&−2@4&−4)] = [■8(3k&−2k@4k&−2k)] − [■8(1×2&0×2@0×2&1×2)] [■8(1&−2@4&−4)] = [■8(3k&−2k@4k&−2k)] − [■8(2&0@0&2)] [■8(1&−2@4&−4)] = [■8(3k−2&−2k−0@4k−0&−2k−2)] [■8(1&−2@4&−4)] = [■8(3k−2&−2k@4k&−2k−2)] Since matrices are equal. Comparing its corresponding elements. 1 = 3k – 2 1 + 2 = 3k 3 = 3k 3/3 = k 1 = k k = 1 Thus, k = 1
