Ex 3.2, 18 - Chapter 3 Class 12 Matrices

Transcript

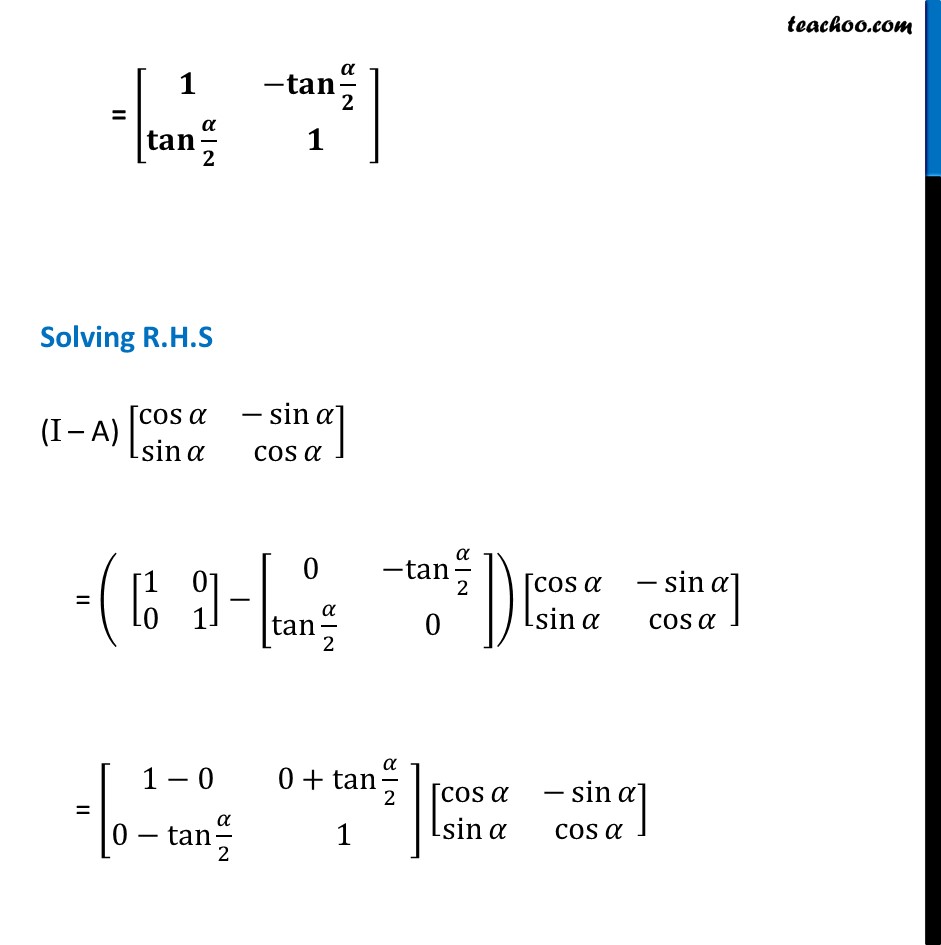
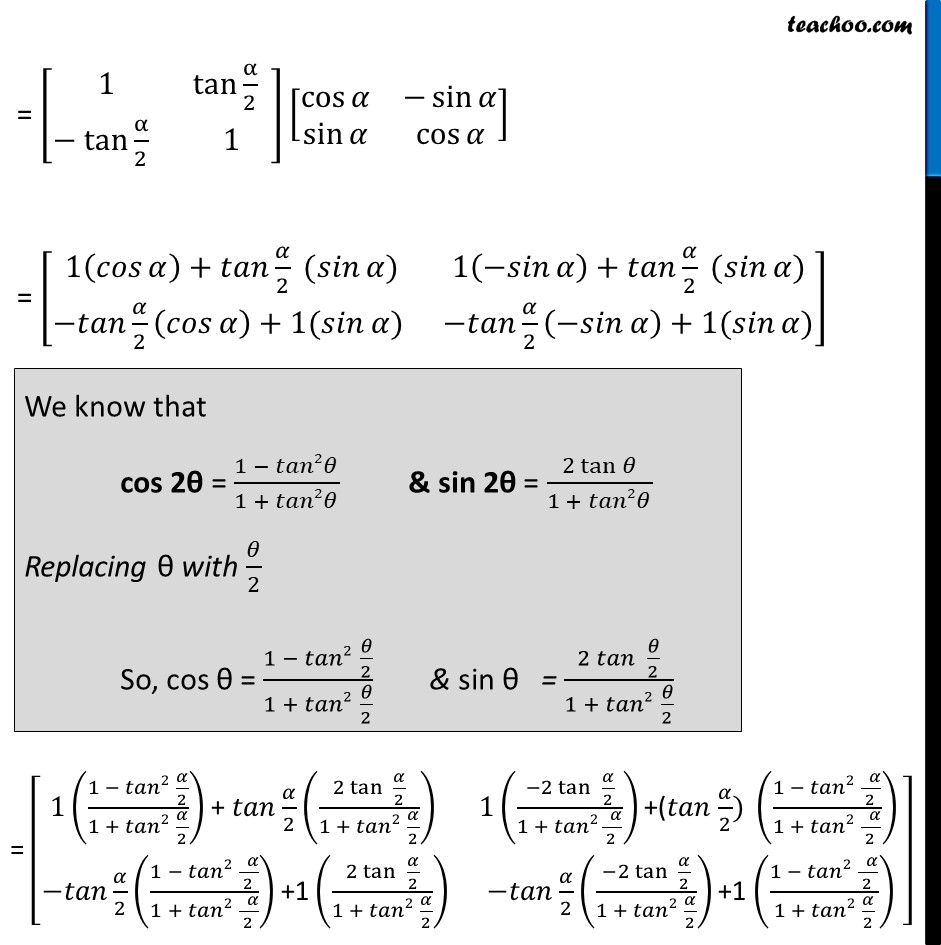
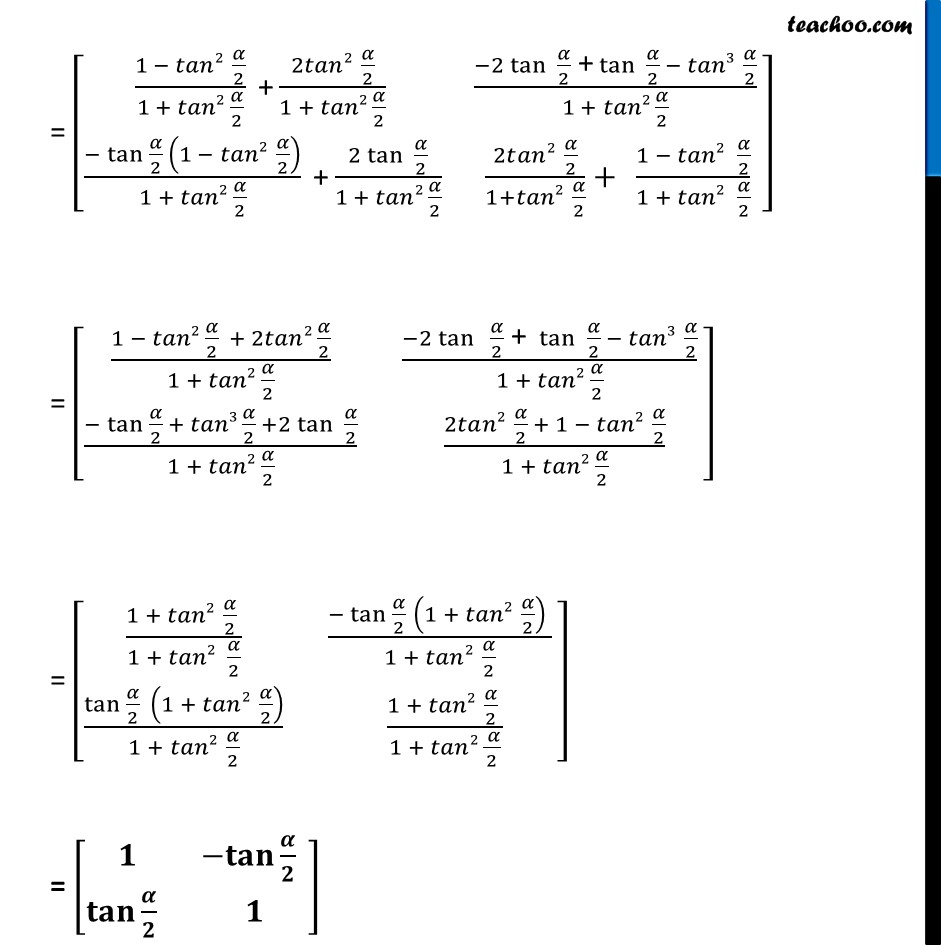
Ex 3.2, 18 If A =[■8(0&−tan 𝛼/2 " " @tan 𝛼/2 " " &0)] and I is the identity matrix of order 2, Show that I + A = ( I – A)[■8(cos⁡𝛼&−sin⁡𝛼@sin⁡𝛼&cos⁡𝛼 )] Given I the identity matrix of order 2 i.e. I = [■8(1&0@0&1)] Solving L.H.S. I + A = [■8(1&0@0&1)] + [■8(0&−tan 𝛼/2 " " @tan 𝛼/2 " " &0)] = [■8(1+0&0−tan 𝛼/2 " " @0+tan 𝛼/2 " " &1+0)] = [■8(𝟏&−𝐭𝐚𝐧 𝜶/𝟐 " " @𝐭𝐚𝐧 𝜶/𝟐 " " &𝟏)] Solving R.H.S (I – A) [■8(cos⁡𝛼&−sin⁡𝛼@sin⁡𝛼&cos⁡𝛼 )] = (" " [■8(1&0@0&1)]−[■8(0&−tan 𝛼/2 " " @tan 𝛼/2 " " &0)]) [■8(cos⁡𝛼&−sin⁡𝛼@sin⁡𝛼&cos⁡𝛼 )] = [■8(1−0&0+tan 𝛼/2 " " @0−tan 𝛼/2 " " &1)][■8(cos⁡𝛼&−sin⁡𝛼@sin⁡𝛼&cos⁡𝛼 )] = [■8(1&tan α/2 " " @− tan α/2 " " &1)][■8(cos⁡𝛼&−sin⁡𝛼@sin⁡𝛼&cos⁡𝛼 )] = [■8(1(𝑐𝑜𝑠⁡𝛼 )+𝑡𝑎𝑛 𝛼/2 (𝑠𝑖𝑛⁡𝛼)&1(〖−𝑠𝑖𝑛〗⁡𝛼 )+𝑡𝑎𝑛 𝛼/2 (𝑠𝑖𝑛⁡𝛼)@−𝑡𝑎𝑛 𝛼/2 (𝑐𝑜𝑠⁡𝛼 )+1(𝑠𝑖𝑛⁡𝛼) &−𝑡𝑎𝑛 𝛼/2 (〖−𝑠𝑖𝑛〗⁡𝛼 )+1(𝑠𝑖𝑛⁡𝛼))] = [■8(1((1 − 𝑡𝑎𝑛2 𝛼/2)/(1 + 𝑡𝑎𝑛2 𝛼/2))"+ " 𝑡𝑎𝑛 𝛼/2 ((2 tan⁡〖 𝛼/2〗)/(1 + 𝑡𝑎𝑛2 𝛼/2))" " &1((−2 tan⁡〖 𝛼/2〗)/(1 + 𝑡𝑎𝑛2 ( 𝛼)/2))"+(" 𝑡𝑎𝑛 𝛼/2) ((1 − 𝑡𝑎𝑛2 ( 𝛼)/2)/(1 + 𝑡𝑎𝑛2 ( 𝛼)/2))" " @−𝑡𝑎𝑛 𝛼/2 ((1 − 𝑡𝑎𝑛2 ( 𝛼)/2)/(1 + 𝑡𝑎𝑛2 ( 𝛼)/2))" +1" ((2 tan⁡〖 𝛼/2〗)/(1 + 𝑡𝑎𝑛2 𝛼/2))&−𝑡𝑎𝑛 𝛼/2 ((−2 〖tan 〗⁡〖𝛼/2〗)/(1 + 𝑡𝑎𝑛2 𝛼/2))" +1" ((1 − 𝑡𝑎𝑛2 ( 𝛼)/2)/(1 + 𝑡𝑎𝑛2 𝛼/2)) )] We know that cos 2θ = (1 − 𝑡𝑎𝑛2𝜃)/(1 + 𝑡𝑎𝑛2𝜃) & sin 2θ = (2 tan⁡𝜃)/(1 + 𝑡𝑎𝑛2𝜃) Replacing θ with 𝜃/2 So, cos θ = (1 − 𝑡𝑎𝑛2 𝜃/2)/(1 + 𝑡𝑎𝑛2 𝜃/2) & sin θ = (2 𝑡𝑎𝑛⁡〖 𝜃/2〗)/(1 + 𝑡𝑎𝑛2 𝜃/2) = [■8((1 − 𝑡𝑎𝑛2 𝛼/2)/(1 + 𝑡𝑎𝑛2 𝛼/2) " +" (2𝑡𝑎𝑛2 𝛼/2)/(1 + 𝑡𝑎𝑛2 𝛼/2) " " &(−2 tan⁡〖 𝛼/2〗 " +" tan⁡〖 𝛼/2〗 − 𝑡𝑎𝑛3 𝛼/2)/(1 + 𝑡𝑎𝑛2 𝛼/2) " " @(−〖tan 〗⁡〖𝛼/2〗 (1 − 𝑡𝑎𝑛2 𝛼/2))/(1 + 𝑡𝑎𝑛2 𝛼/2) " +" (2 tan⁡〖 𝛼/2〗)/(1 + 𝑡𝑎𝑛2 𝛼/2)&(2𝑡𝑎𝑛2 𝛼/2)/(1+𝑡𝑎𝑛2 𝛼/2)+ " " (1 − 𝑡𝑎𝑛2 𝛼/2)/(1 + 𝑡𝑎𝑛2 𝛼/2))] = [■8((1 − 𝑡𝑎𝑛2 𝛼/2 + 2𝑡𝑎𝑛2 𝛼/2)/(1 + 𝑡𝑎𝑛2 𝛼/2) " " &(−2 〖tan 〗⁡〖 𝛼/2〗 " + " tan⁡〖 𝛼/2〗 − 𝑡𝑎𝑛3 𝛼/2)/(1 + 𝑡𝑎𝑛2 𝛼/2) " " @(−〖tan 〗⁡〖𝛼/2〗 + 𝑡𝑎𝑛3 𝛼/2 +2 tan⁡〖 𝛼/2〗)/(1 + 𝑡𝑎𝑛2 𝛼/2) " " &" " (2𝑡𝑎𝑛2 𝛼/2 + 1 − 𝑡𝑎𝑛2 𝛼/2)/(1 + 𝑡𝑎𝑛2 𝛼/2))] = [■8((1 + 𝑡𝑎𝑛2 𝛼/2 )/(1 + 𝑡𝑎𝑛2 𝛼/2) " " &(−〖tan 〗⁡〖𝛼/2〗 " " (1 + 𝑡𝑎𝑛2 𝛼/2) )/(1 + 𝑡𝑎𝑛2 𝛼/2) " " @(〖tan 〗⁡〖𝛼/2〗 (1 + 𝑡𝑎𝑛2 𝛼/2))/(1 + 𝑡𝑎𝑛2 𝛼/2) " " &" " (1 + 𝑡𝑎𝑛2 𝛼/2 )/(1 + 𝑡𝑎𝑛2 ( 𝛼)/2))] = [■8(𝟏&−𝐭𝐚𝐧 𝜶/𝟐 " " @𝐭𝐚𝐧 𝜶/𝟐 " " &𝟏)] = R.H.S. Hence proved