CBSE Class 12 Sample Paper for 2025 Boards
You are here
CBSE Class 12 Sample Paper for 2025 Boards
Last updated at Feb. 13, 2025 by Teachoo
This question is similar to Chapter 1 Class 12 Relation and Functions - Examples
Please check the question here
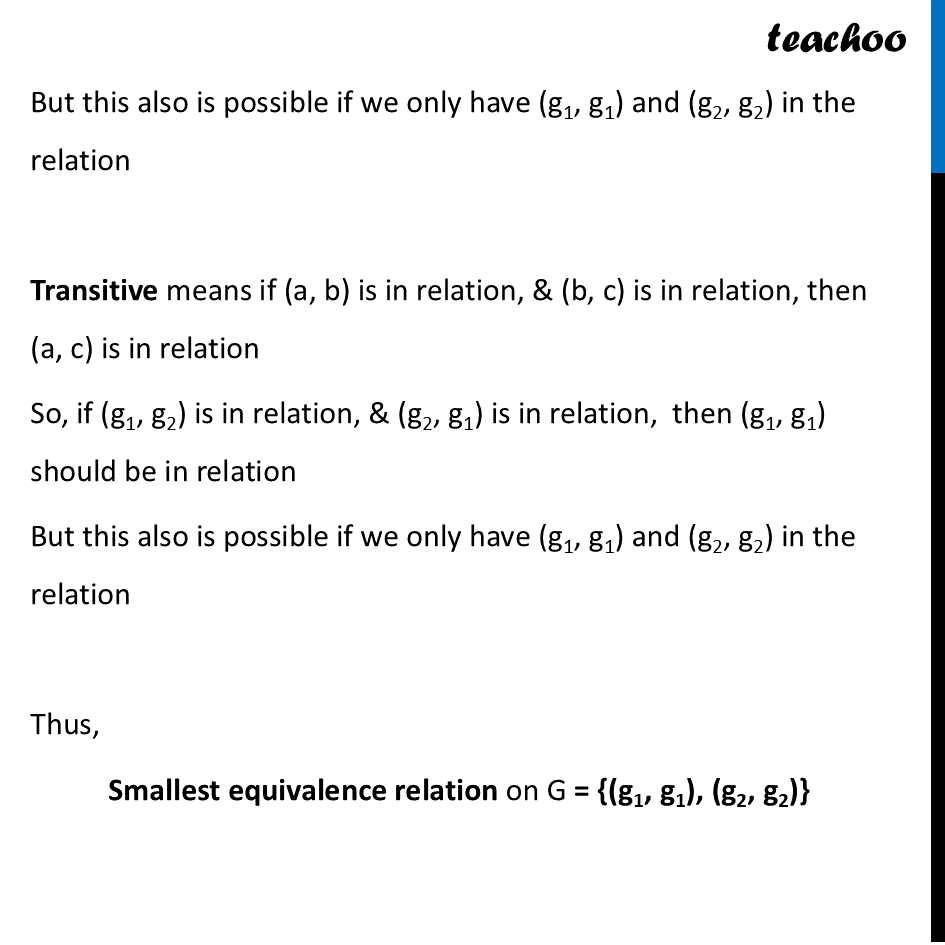
Transcript
Question 37 (ii) Write the smallest equivalence relation on π. Given G = {g1, g2} We need to find smallest equivalence relation on π. Total possible pairs = {(g1, g1) , (g1, g2), (g2, g1), (g2, g2)} Reflexive means (a, a) should be in relation . So, (g1, g1) , (g2, g2) should be in a relation. Itβs important that both are elements of the relation Symmetric means if (a, b) is in relation, then (b, a) should be in relation . So, since (g1, g2) is in relation, (g2, g1) should also be in relation But this also is possible if we only have (g1, g1) and (g2, g2) in the relation Transitive means if (a, b) is in relation, & (b, c) is in relation, then (a, c) is in relation So, if (g1, g2) is in relation, & (g2, g1) is in relation, then (g1, g1) should be in relation But this also is possible if we only have (g1, g1) and (g2, g2) in the relation Thus, Smallest equivalence relation on G = {(g1, g1), (g2, g2)}
