[Video] A person standing at O(0,0,0) is watching an aeroplane which

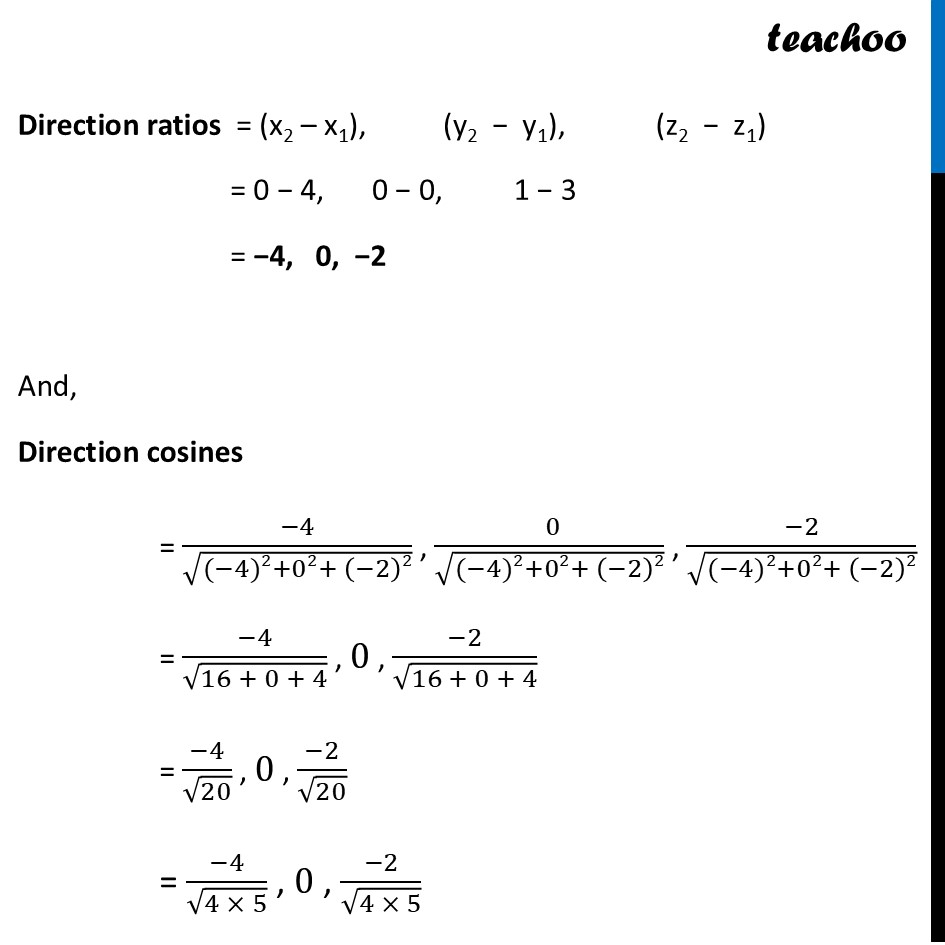
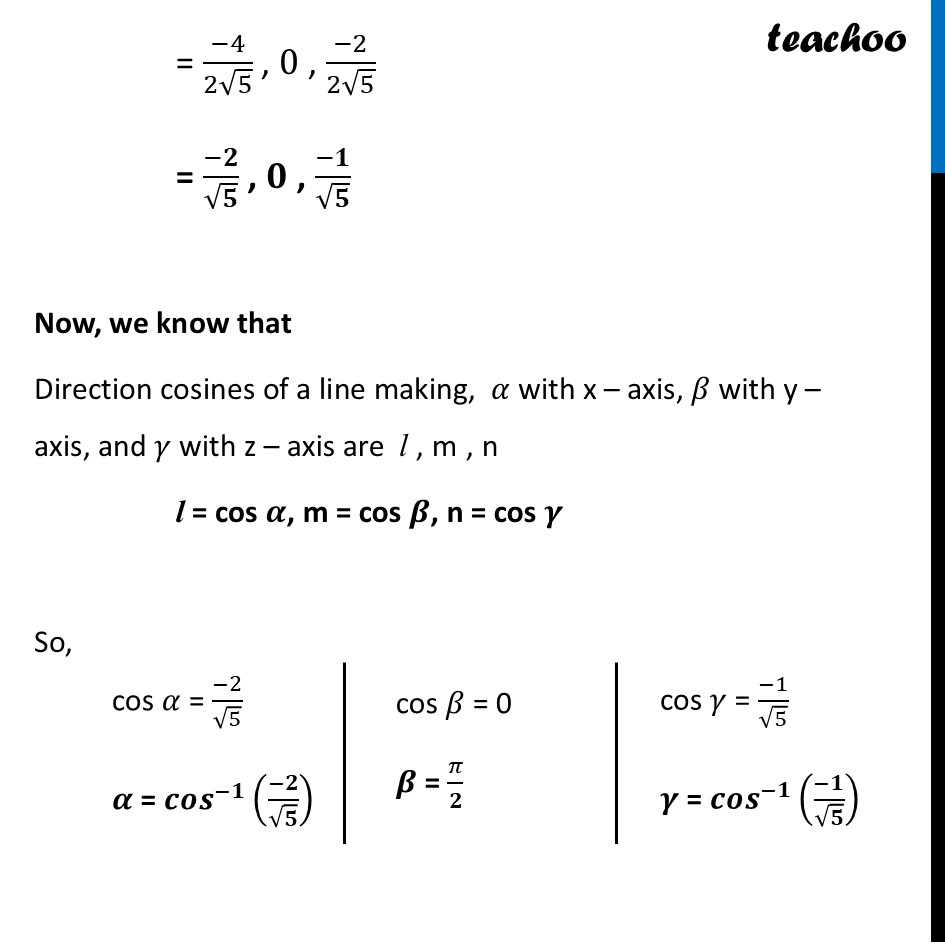
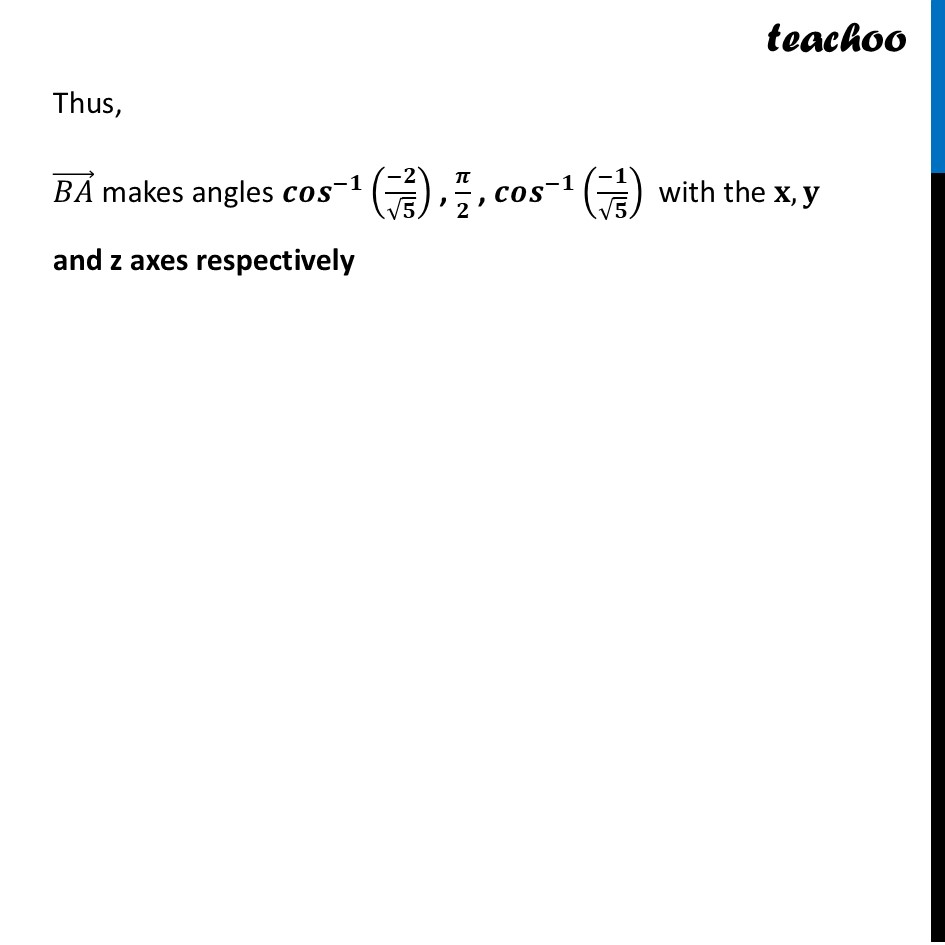
Question 24 (B) A person standing at 𝑶(𝟎,𝟎,𝟎) is watching an aeroplane which is at the coordinate point 𝑨(𝟒,𝟎,𝟑). At the same time he saw a bird at the coordinate point 𝑩(𝟎,𝟎,𝟏). Find the angles which (𝐵𝐴) ⃗ makes with the x,y and z axes.Now, Angles which (𝑩𝑨) ⃗ makes with the x,y and z axes = Direction cosines of (𝑩𝑨) ⃗ Given A (4, 0, 3) & B (0, 0, 1) So, x1 = 4, y1 = 0, z1 = 3 & x2 = 0, y2 = 0, z2 = 1 Direction ratios = (x2 – x1), (y2 − y1), (z2 − z1) = 0 − 4, 0 − 0, 1 − 3 = −4, 0, −2 And, Direction cosines = (−4)/√((−4)2+02+ (−2)2) , ( 0)/√((−4)2+02+ (−2)2) , (−2)/√((−4)2+02+ (−2)2) = (−4)/√(16 + 0 + 4) , 0 , (−2)/√(16 + 0 + 4) = (−4)/√20 , 0 , (−2)/√20 = (−4)/√(4 × 5) , 0 , (−2)/√(4 × 5) = (−4)/(2√5) , 0 , (−2)/(2√5) = (−𝟐)/√𝟓 , 𝟎 , (−𝟏)/√𝟓 Now, we know that Direction cosines of a line making, 𝛼 with x – axis, 𝛽 with y – axis, and 𝛾 with z – axis are l,m,n l = cos 𝜶, m = cos 𝜷, n = cos 𝜸 So, cos 𝛼 = (−2)/√5 𝜶 = 〖𝒄𝒐𝒔〗^(−𝟏)⁡((−𝟐)/√𝟓) cos 𝛽 = 0 𝜷 = 𝜋/𝟐 cos 𝛾 = (−1)/√5 𝜸 = 〖𝒄𝒐𝒔〗^(−𝟏)⁡((−𝟏)/√𝟓) Thus, (𝐵𝐴) ⃗ makes angles 〖𝒄𝒐𝒔〗^(−𝟏)⁡((−𝟐)/√𝟓) , 𝝅/𝟐 , 〖𝒄𝒐𝒔〗^(−𝟏)⁡((−𝟏)/√𝟓) with the 𝐱,𝐲 and z axes respectively

Question 24 (B) - CBSE Class 12 Sample Paper for 2025 Boards - Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Davneet Singh

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular