Question 37 (iii) (A) - CBSE Class 12 Sample Paper for 2025 Boards - Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Transcript

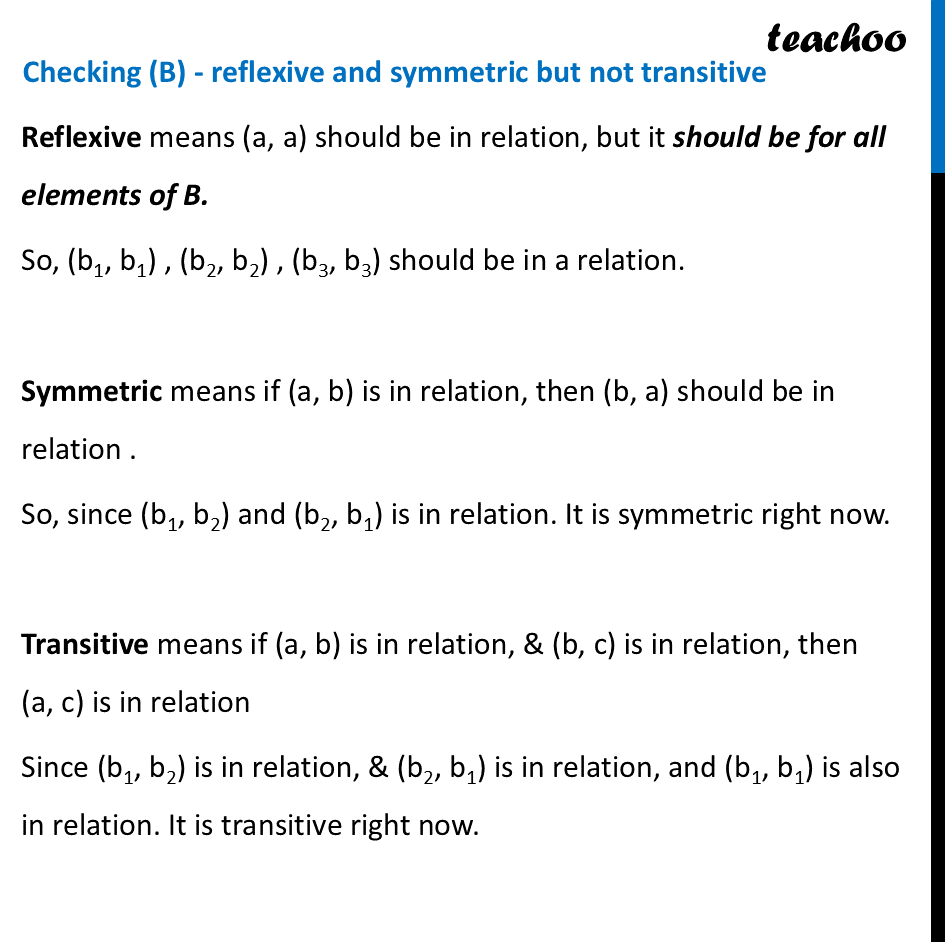
Question 37 (iii) (A) Ravi defines a relation from B to B as 𝑹_𝟏={(𝒃_𝟏,𝒃_𝟐 ),(𝒃_𝟐,𝒃_𝟏 )}. Write the minimum ordered pairs to be added in 𝑹_𝟏 so that it becomes (A) reflexive but not symmetric, (B) reflexive and symmetric but not transitive.Given, 𝑅_1={(𝑏_1,𝑏_2 ),(𝑏_2,𝑏_1 )} and B = {b1, b2, b3}, Checking (A) - reflexive but not symmetric Reflexive means (a, a) should be in relation, but it should be for all elements of B. So, (b1, b1) , (b2, b2) , (b3, b3) should be in a relation. Symmetric means if (a, b) is in relation, then (b, a) should be in relation . So, since (b1, b2) and (b2, b1) is in relation. It is symmetric right now. Thus, we should add one more element which makes not symmetric. Let’s add (b2, b3) in the relation ∴ Required Relation = {(b1, b2), (b2, b1) ,(b1, b1) , (b2, b2) , (b3, b3), (b2, b3) } Thus, minimum 4 ordered pairs are added Checking (B) - reflexive and symmetric but not transitive Reflexive means (a, a) should be in relation, but it should be for all elements of B. So, (b1, b1) , (b2, b2) , (b3, b3) should be in a relation. Symmetric means if (a, b) is in relation, then (b, a) should be in relation . So, since (b1, b2) and (b2, b1) is in relation. It is symmetric right now. Transitive means if (a, b) is in relation, & (b, c) is in relation, then (a, c) is in relation Since (b1, b2) is in relation, & (b2, b1) is in relation, and (b1, b1) is also in relation. It is transitive right now. So, let’s add (b2, b3) in the relation Now, to make it symmetric, we need to add (b3, b2) in the relation Let’s check transitive – Since (b1, b2) is in relation, & (b2, b3) is in relation, but (b1, b3) is not in relation. ∴ Relation is not transitive Thus, ∴ Required Relation = {(b1, b2), (b2, b1) ,(b1, b1) , (b2, b2) , (b3, b3), (b2, b3), (b3, b2) } Thus, minimum 5 ordered pairs are added