CBSE Class 12 Sample Paper for 2023 Boards
You are here
CBSE Class 12 Sample Paper for 2023 Boards
Last updated at Dec. 13, 2024 by Teachoo
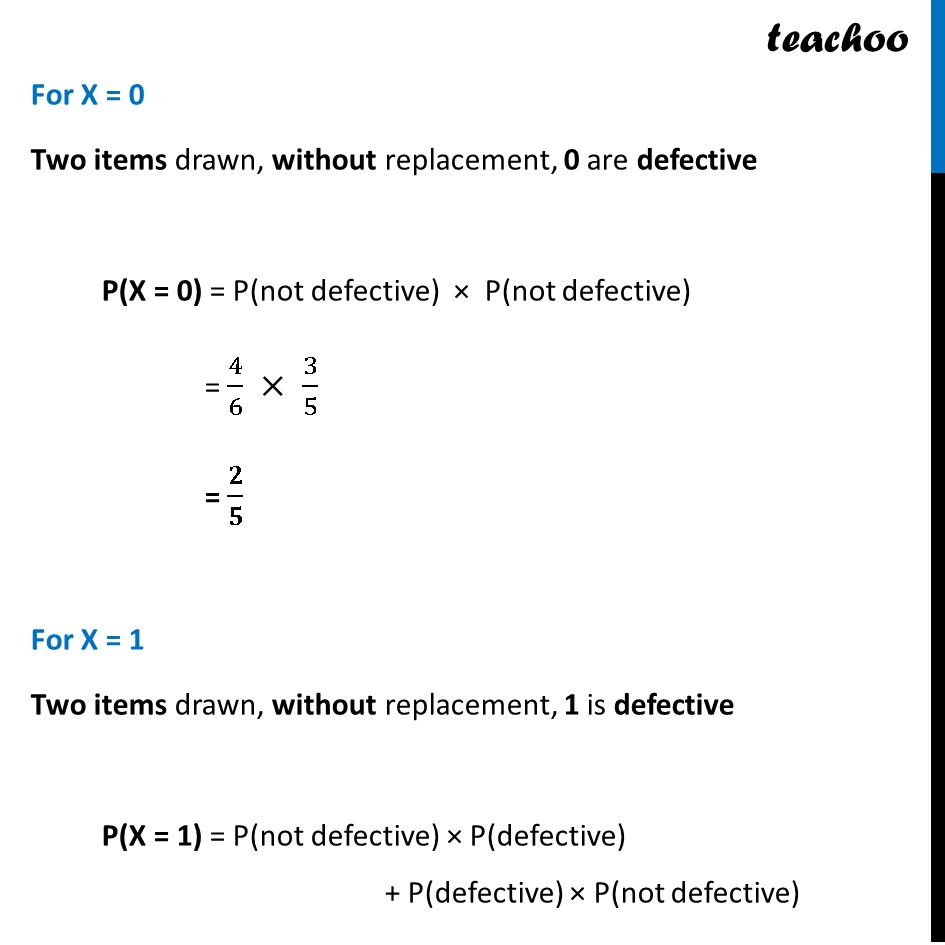
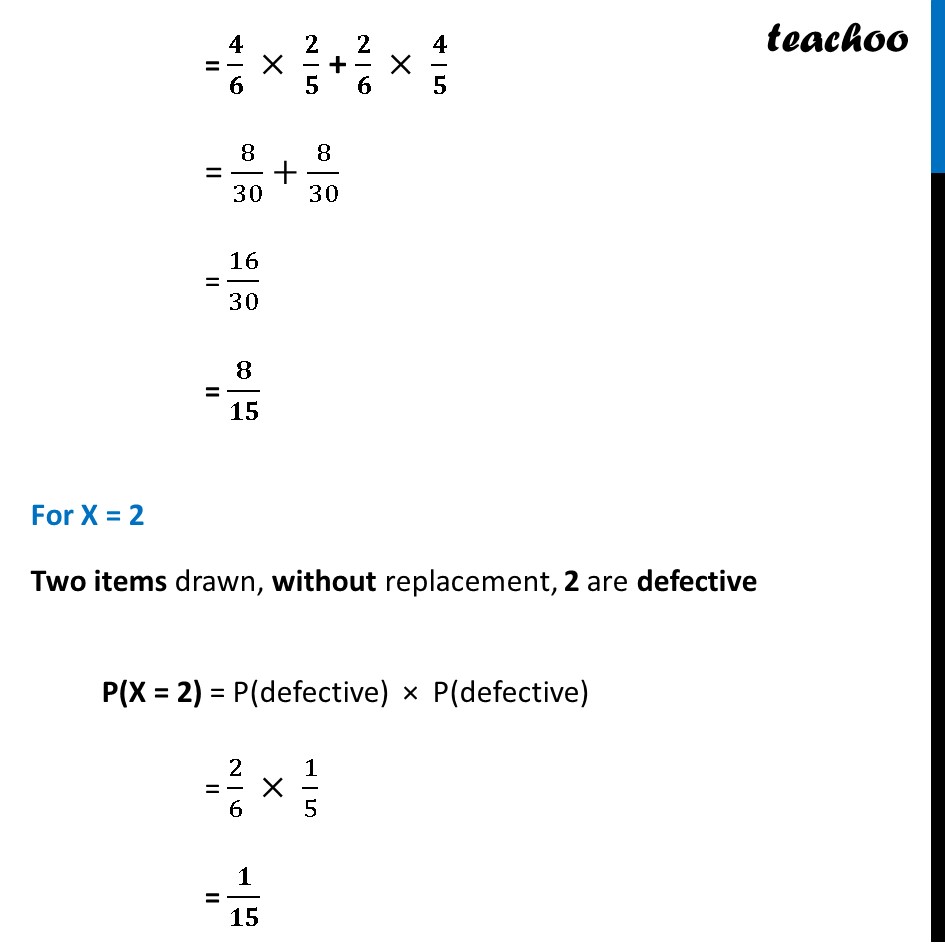
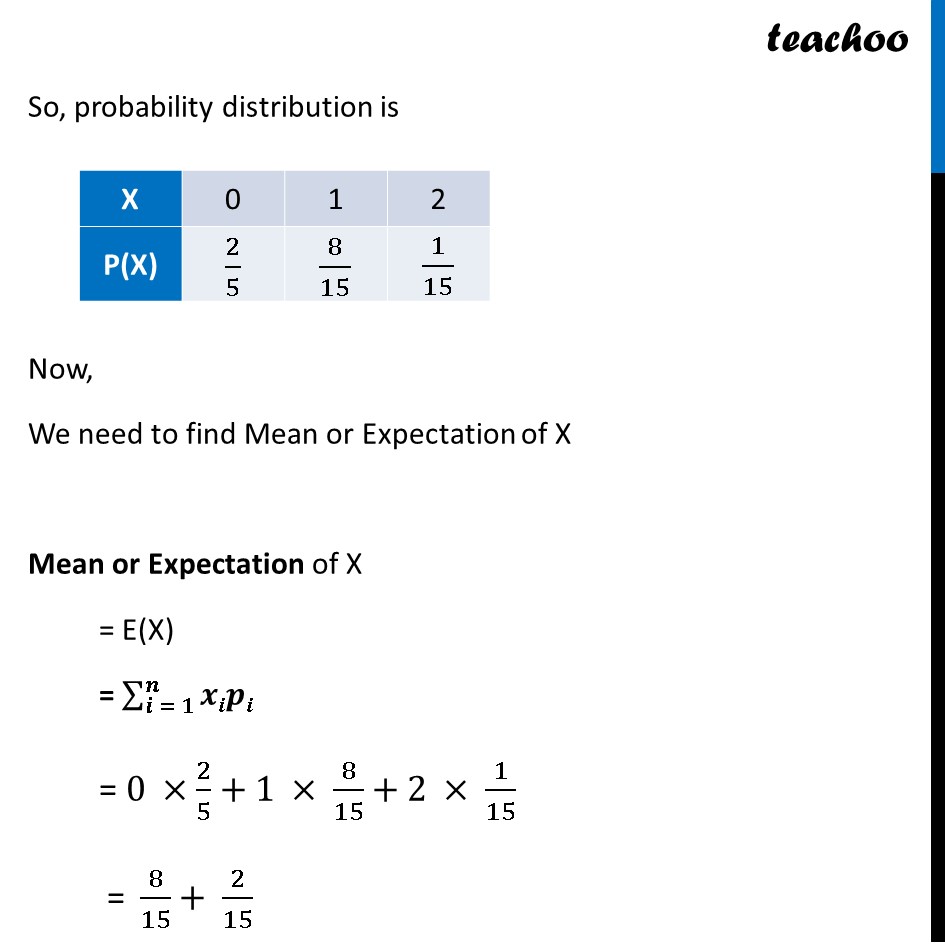
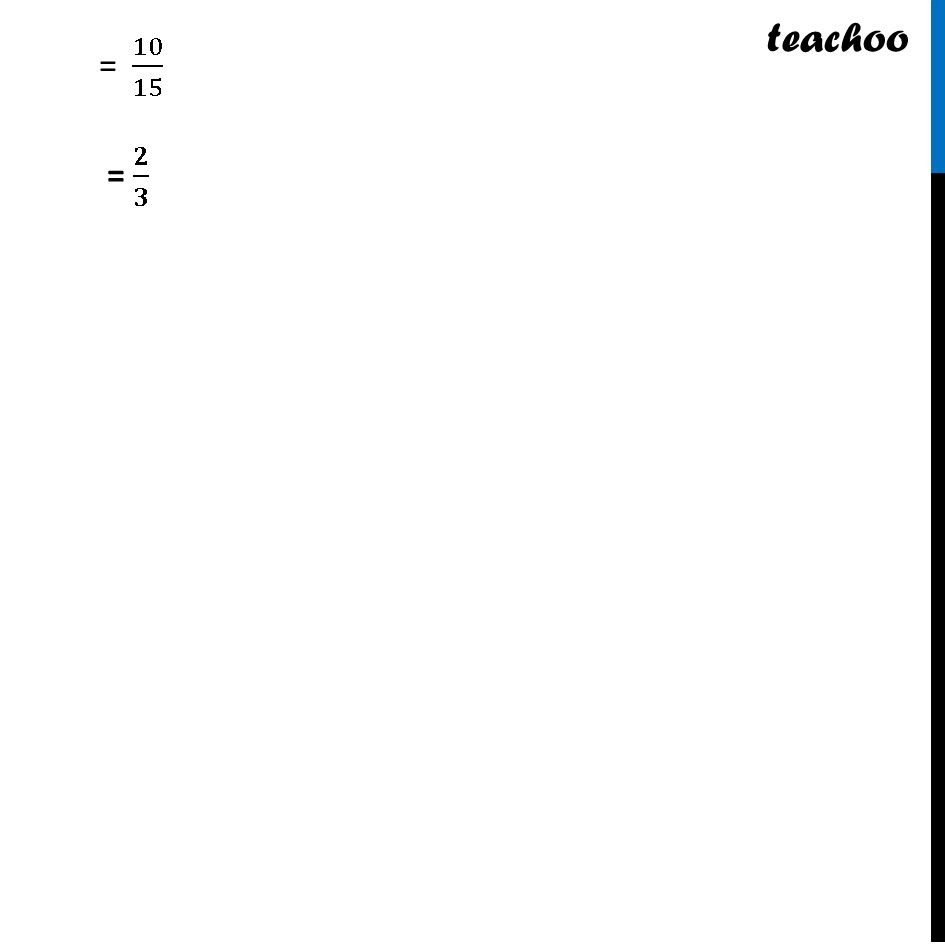
Question 27 (Choice 2) Find the mean number of defective items in a sample of two items drawn one-by-one without replacement from an urn containing 6 items, which include 2 defective items. Assume that the items are identical in shape and size. Let X : Number of defective items drawn Since there are maximum of 2 defective items We can get 0, 1 or 2 defective items So, X = 0 or X = 1 or X = 2 Finding probabilities separately For X = 0 Two items drawn, without replacement, 0 are defective P(X = 0) = P(not defective) × P(not defective) = 4/6 × 3/5 = 𝟐/𝟓 For X = 1 Two items drawn, without replacement, 1 is defective P(X = 1) = P(not defective) × P(defective) + P(defective) × P(not defective) = 𝟒/𝟔 × 𝟐/𝟓 + 𝟐/𝟔 × 𝟒/𝟓 = 8/30+8/30 = 16/30 = 𝟖/𝟏𝟓 For X = 2 Two items drawn, without replacement, 2 are defective P(X = 2) = P(defective) × P(defective) = 2/6 × 1/5 = 𝟏/𝟏𝟓 So, probability distribution is Now, We need to find Mean or Expectation of X Mean or Expectation of X = E(X) = ∑_(𝒊 = 𝟏)^𝒏▒𝒙𝒊𝒑𝒊 = 0 ×2/5+1 × 8/15+2 × 1/15 = 8/15+ 2/15 = 10/15 = 𝟐/𝟑
