Introducing your new favourite teacher - Teachoo Black, at only ₹83 per month
Ex 2.2, 10 - Chapter 2 Class 12 Inverse Trigonometric Functions (Term 1)
Last updated at May 12, 2021 by Teachoo
Ex 2.2
Ex 2.2,1
Ex 2.2, 2 Important
Ex 2.2, 3
Ex 2.2, 4 Important
Ex 2.2, 5 Important
Ex 2.2, 6
Ex 2.2, 7 Important
Ex 2.2, 8 Important
Ex 2.2, 9
Ex 2.2, 10 Important You are here
Ex 2.2, 11
Ex 2.2, 12 Important
Ex 2.2, 13 Important
Ex 2.2, 14 Important
Ex 2.2, 15 Important
Ex 2.2, 16
Ex 2.2, 17
Ex 2.2, 18 Important
Ex 2.2, 19 (MCQ) Important
Ex 2.2, 20 (MCQ) Important
Ex 2.2, 21 (MCQ)
Chapter 2 Class 12 Inverse Trigonometric Functions
Serial order wise
Transcript
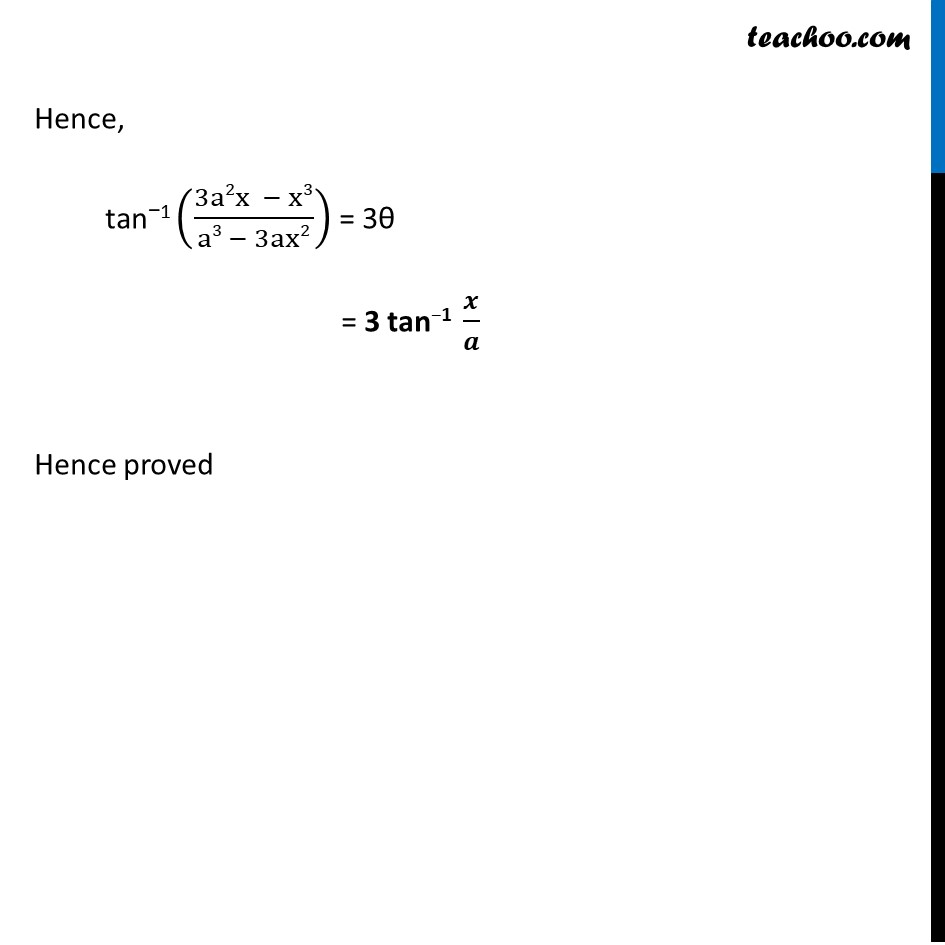
Ex 2.2, 10 Write the function in the simplest form: tan−1 ((3a2x −x3)/(a3 −3ax2)), α > 0; (−a)/√3 ≤ x ≤ a/√3 tan−1 ((3a2x − x3)/(a3 − 3ax2)) Putting x = a tan θ = tan−1 ((3a^2 (a tan θ) − (a tan θ)^3)/(a^3 − 3a (a tan θ)^2 )) = tan−1 ((3a^3 tan θ − a^3 tan^3 θ)/(a^3 − 3 a.a^2 tan^2 θ)) = tan−1 ((a^3 (3 tan θ − tan^3 θ))/(a^3 (1 − 3 tan^2 θ))) = tan−1 ((3 tan θ − tan^3 θ)/(1 − 3tan^2 θ)) = tan−1 (tan 3θ) = 3θ We know x = a tan θ 𝑥/𝑎 = tan θ tan θ = 𝑥/𝑎 θ = tan−1 (𝒙/𝒂) ("Using tan 3x = " (3 tan〖𝑥 − tan3𝑥 〗)/(1 − 3𝑡𝑎𝑛2𝑥)) Hence, tan−1 ((3a2x − x3)/(a3 − 3ax2)) = 3θ = 3 tan−1 𝒙/𝒂 Hence proved
