Ex 8.2, 26 - Chapter 8 Class 11 Sequences and Series

Last updated at Dec. 16, 2024 by

Ex 8.2, 26 - Insert two numbers between 3 and 81 so that - Ex 8.2

part 2 - Ex 8.2, 26 - Ex 8.2 - Serial order wise - Chapter 8 Class 11 Sequences and Series

Share on WhatsApp

Transcript

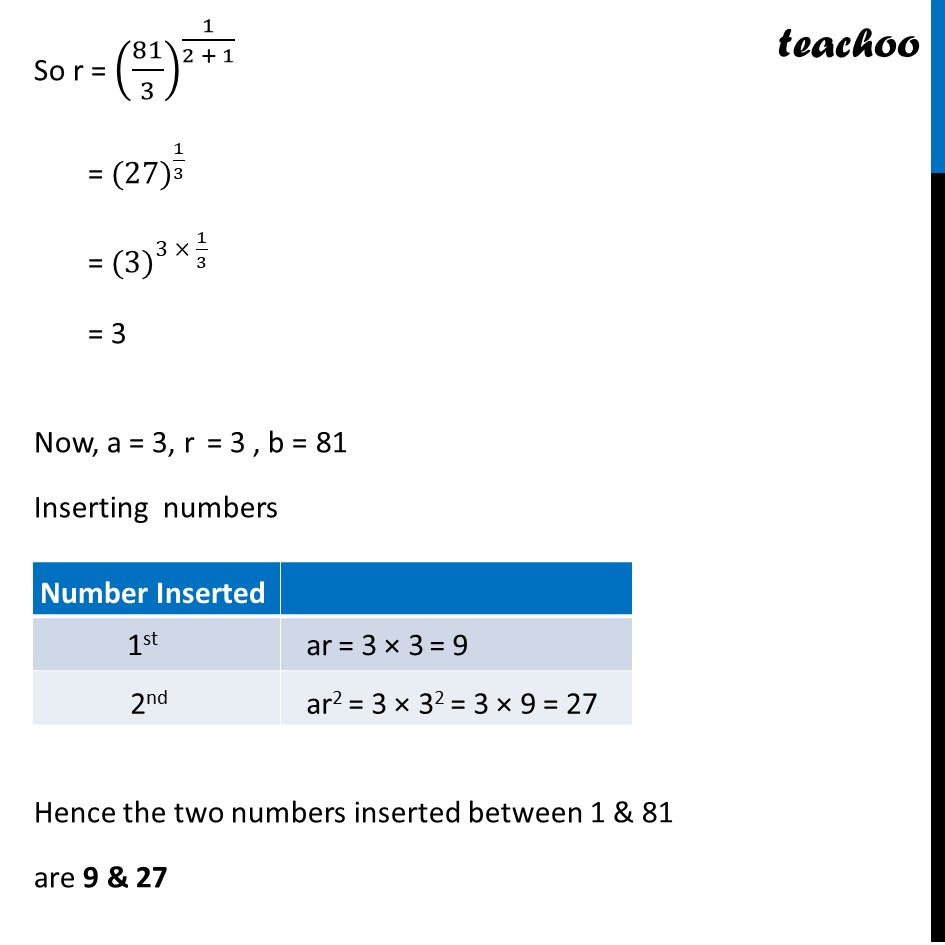
Ex 8.2, 26 Insert two numbers between 3 and 81 so that the resulting sequence is G.P. We know that to insert n numbers between a & b Common ratio r = ( / )^(1/( +1)) We need to insert 2 numbers between 3 & 81 Here a = 3 & b = 81 & n = number of terms to be inserted = 2 So r = (81/3)^(1/(2+1)) = (27) ^(1/3) = (3) ^(3 1/3 ) = 3 Ex 8.2, 26 Insert two numbers between 3 and 81 so that the resulting sequence is G.P. We know that to insert n numbers between a & b Common ratio r = ( / )^(1/( +1)) We need to insert 2 numbers between 3 & 81 Here a = 3 & b = 81 & n = number of terms to be inserted = 2 So r = (81/3)^(1/(2+1)) = (27) ^(1/3) = (3) ^(3 1/3 ) = 3 Thus, a = 3 , r = 3, b = 81 Inserting numbers Hence the two numbers inserted between 1 & 81 are 9 & 27 Thus, a = 3 , r = 3, b = 81 Inserting numbers Hence the two numbers inserted between 1 & 81 are 9 & 27

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo