Ex 8.2, 20 - Chapter 8 Class 11 Sequences and Series

Last updated at April 16, 2024 by

Slide88.JPG

Slide89.JPG
Slide90.JPG

Transcript

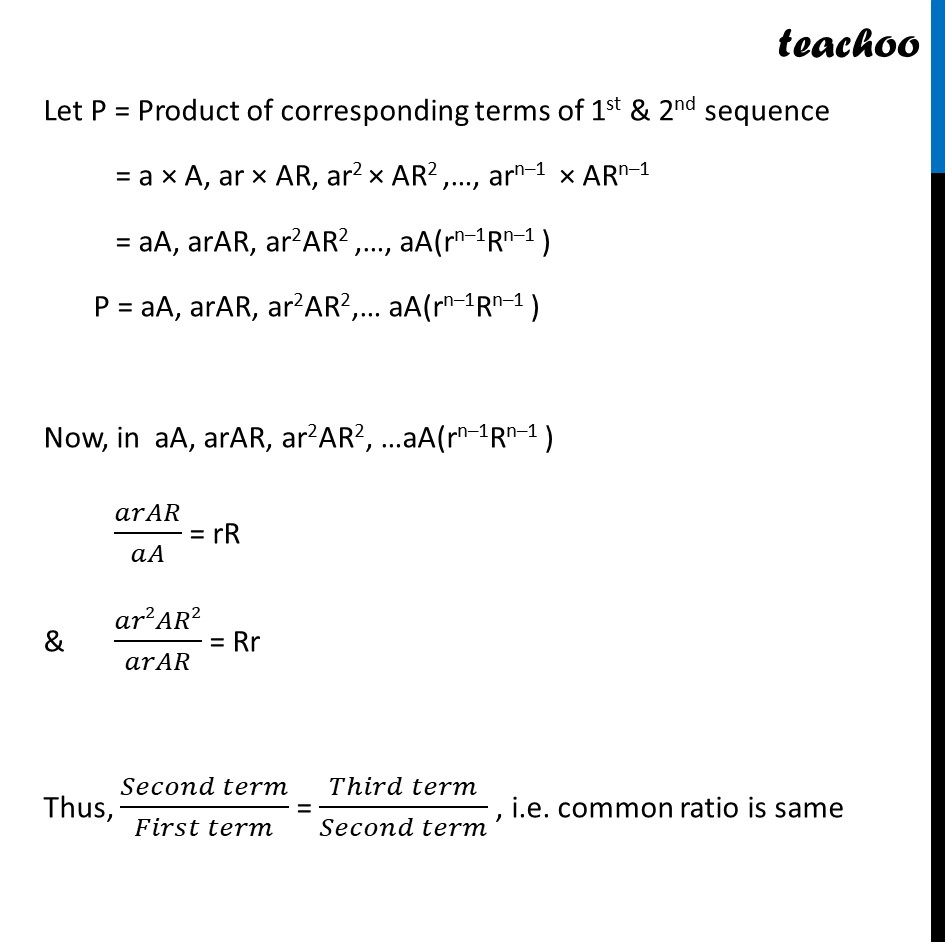
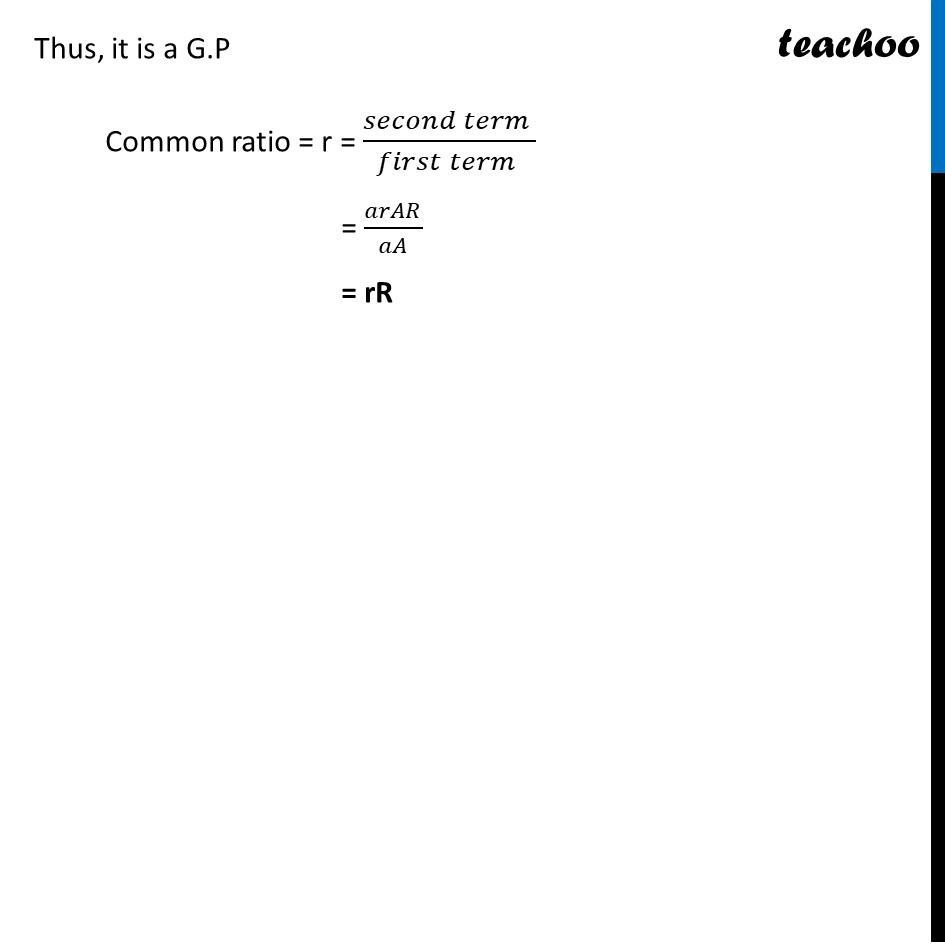
Ex 8.2, 20 Show that the products of the corresponding terms of the sequences a, ar, ar2, ar3… arn – 1 & A, AR, AR2, AR3…… ARn - 1 form a G.P, and find the common ratio. 1st sequence is a, ar, ar2, ar3… arn – 1 2nd sequence is A, AR, AR2, AR3…… ARn – 1 Let P = Product of corresponding terms of 1st & 2nd sequence = a × A , ar × AR , ar2 × AR2 , … , arn–1 × ARn–1 = aA , arAR , ar2AR2 , …, aA(rn–1Rn–1 ) P = aA, arAR, ar2AR2, …aA(rn–1Rn–1 ) Now, in aA, arAR, ar2AR2, …aA(rn–1Rn–1 ) 𝑎𝑟𝐴𝑅/𝑎𝐴 = rR & 𝑎𝑟2𝐴𝑅2/𝑎𝑟𝐴𝑅 = Rr Thus, (𝑆𝑒𝑐𝑜𝑛𝑑 𝑡𝑒𝑟𝑚)/(𝐹𝑖𝑟𝑠𝑡 𝑡𝑒𝑟𝑚) = (𝑇ℎ𝑖𝑟𝑑 𝑡𝑒𝑟𝑚)/(𝑆𝑒𝑐𝑜𝑛𝑑 𝑡𝑒𝑟𝑚) , i.e. common ratio is same Thus, it is a G.P Common ratio = r = (𝑠𝑒𝑐𝑜𝑛𝑑 𝑡𝑒𝑟𝑚 )/(𝑓𝑖𝑟𝑠𝑡 𝑡𝑒𝑟𝑚 ) = 𝑎𝑟𝐴𝑅/𝑎𝐴 = rR

Go Ad-free
Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.