Example 42 - Chapter 7 Class 12 Integrals

Transcript

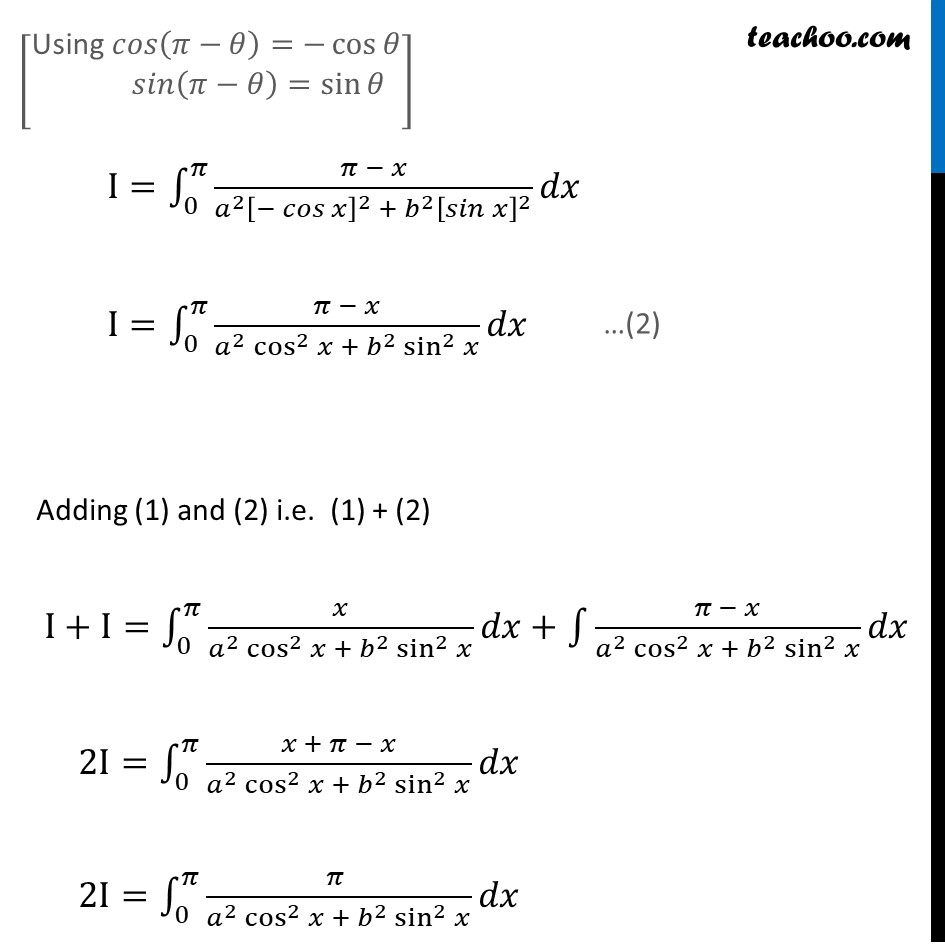
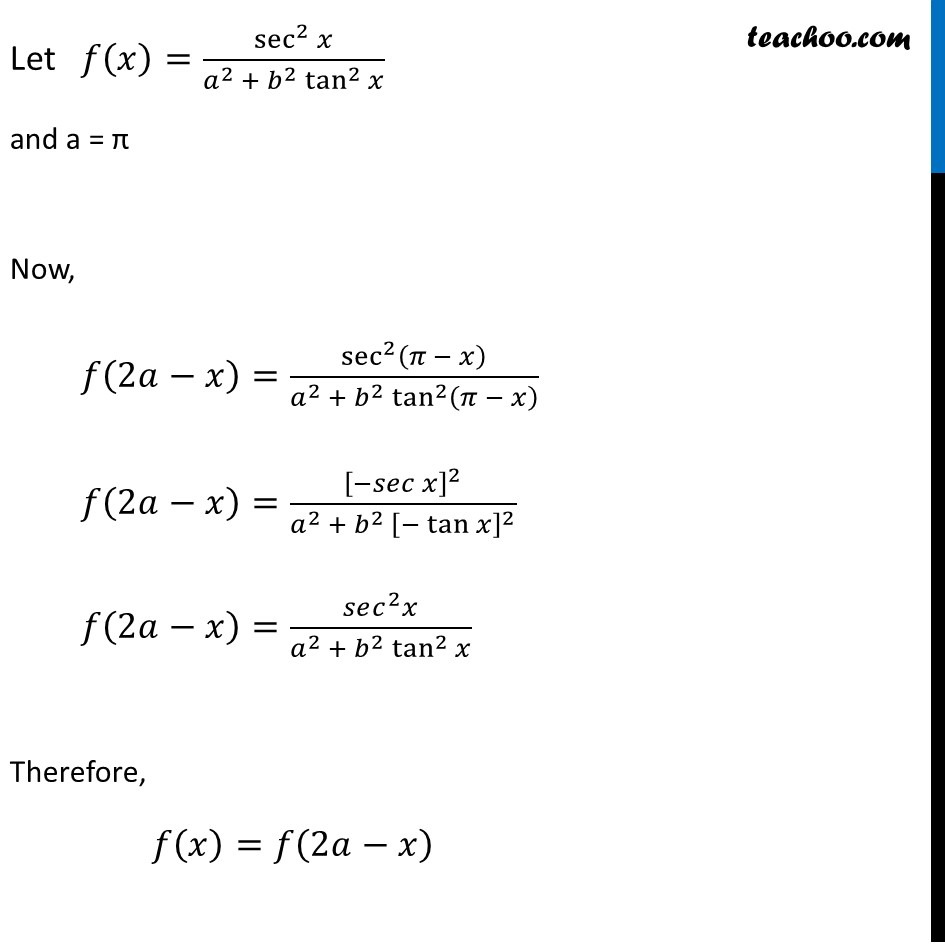
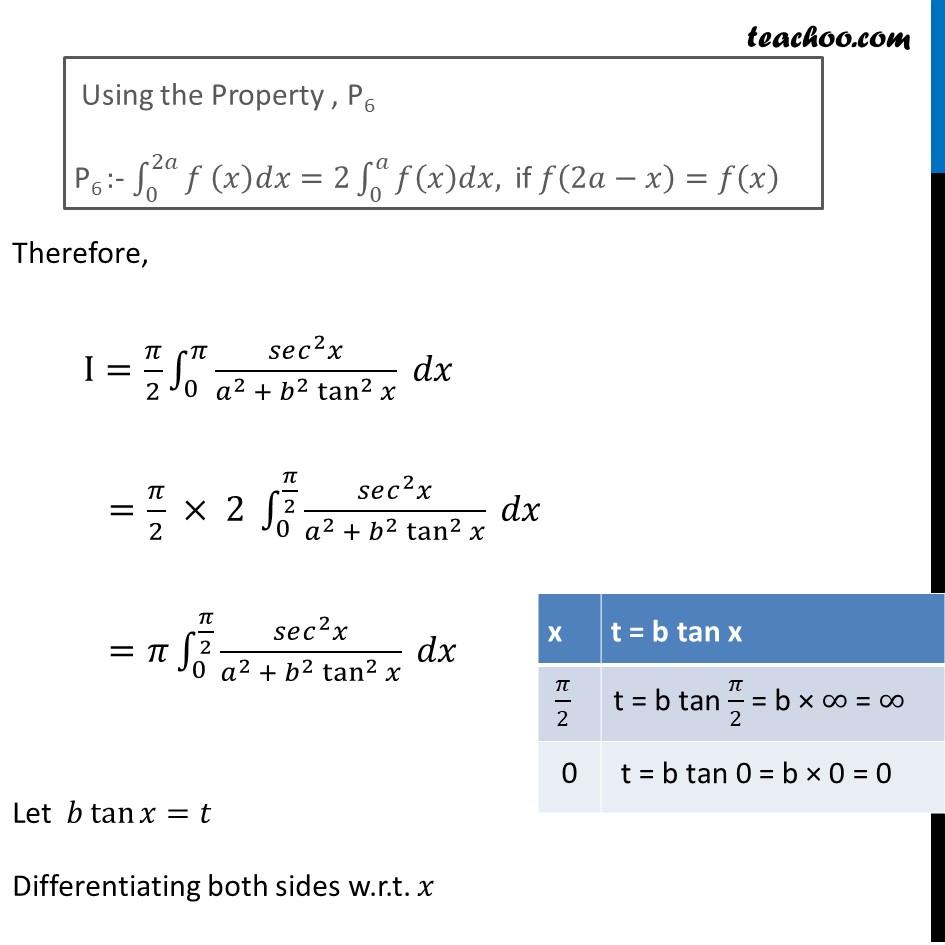
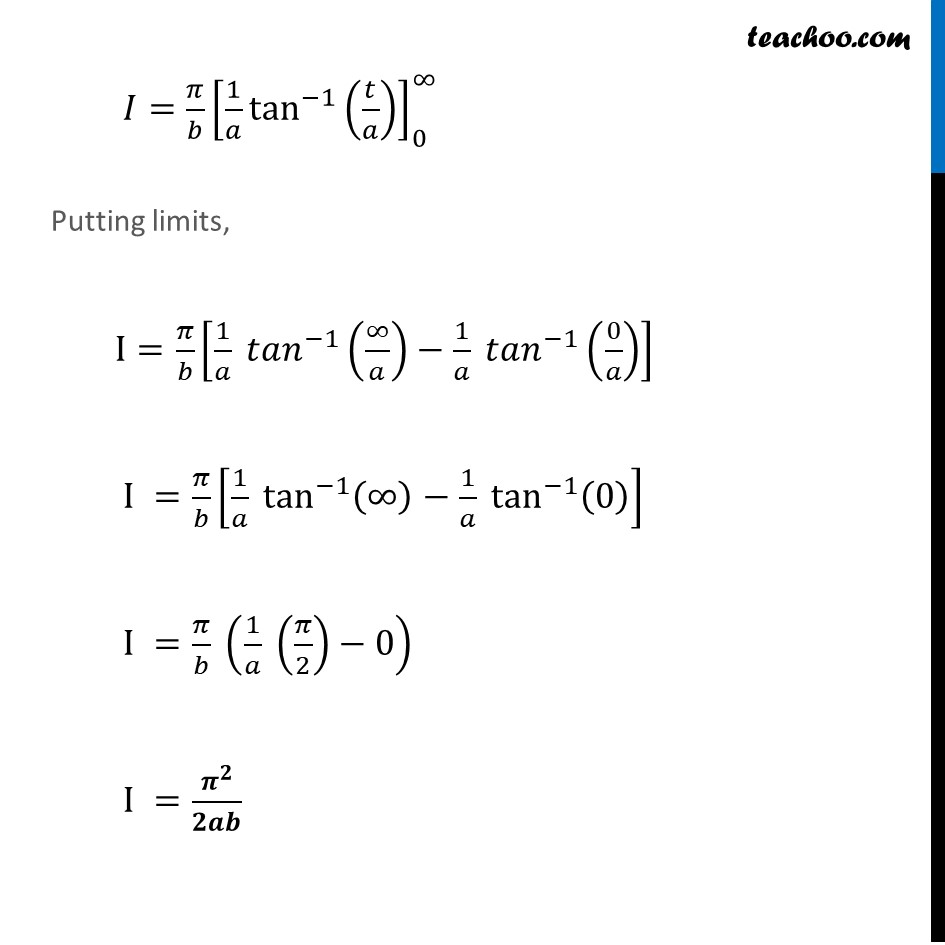
Example 42 Evaluate ∫_0^𝜋▒(𝑥 𝑑𝑥)/(𝑎^2 cos^2⁡〖𝑥 + 𝑏^2 〗 sin^2⁡𝑥 )Let I= ∫_0^𝜋▒〖𝑥/(𝑎^2 𝑐𝑜𝑠^2 𝑥 + 𝑏^2 𝑠𝑖𝑛^2 𝑥) 𝑑𝑥〗 ∴ I=∫_0^𝜋▒〖((𝜋 − 𝑥))/(𝑎^2 𝑐𝑜𝑠^2 (𝜋 − 𝑥) + 𝑏^2 𝑠𝑖𝑛^2 (𝜋 − 𝑥) ) 𝑑𝑥〗 I=∫_0^𝜋▒〖(𝜋 − 𝑥)/(𝑎^2 [𝑐𝑜𝑠(𝜋 − 𝑥)]^2 + 𝑏^2 [𝑠𝑖𝑛(𝜋 − 𝑥)]^2 ) 𝑑𝑥〗 I=∫_0^𝜋▒〖(𝜋 − 𝑥)/(𝑎^2 [− 𝑐𝑜𝑠 𝑥]^2 + 𝑏^2 [𝑠𝑖𝑛 𝑥]^2 ) 𝑑𝑥〗 I=∫_0^𝜋▒〖(𝜋 − 𝑥)/(𝑎^2 cos^2⁡𝑥 + 𝑏^2 sin^2⁡𝑥 ) 𝑑𝑥〗 Adding (1) and (2) i.e. (1) + (2) I+I=∫_0^𝜋▒〖𝑥/(𝑎^2 cos^2⁡𝑥 + 𝑏^2 sin^2⁡𝑥 ) 𝑑𝑥〗+∫1▒(𝜋 − 𝑥)/(𝑎^2 cos^2⁡𝑥 + 𝑏^2 sin^2⁡𝑥 ) 𝑑𝑥 2I=∫_0^𝜋▒(𝑥 + 𝜋 − 𝑥)/(𝑎^2 cos^2⁡𝑥 + 𝑏^2 sin^2⁡𝑥 ) 𝑑𝑥 2I=∫_0^𝜋▒(𝜋 )/(𝑎^2 cos^2⁡𝑥 + 𝑏^2 sin^2⁡𝑥 ) 𝑑𝑥 I=𝜋/2 ∫_0^𝜋▒〖1/(𝑎^2 cos^2⁡𝑥 + 𝑏^2 sin^2⁡𝑥 ) 𝑑𝑥〗 Dividing numerator and denominator by 𝑐𝑜𝑠^2 𝑥, we get I=𝜋/2 ∫_0^𝜋▒〖(1/cos^2⁡𝑥 )/((𝑎^2 cos^2⁡〖𝑥 + 𝑏^2 sin^2⁡𝑥 〗)/cos^2⁡𝑥 ) 𝑑𝑥〗 I=𝜋/2 ∫_0^𝜋▒〖(𝑠𝑒𝑐^2 𝑥)/((𝑎^2 cos^2⁡𝑥)/cos^2⁡𝑥 + (𝑏^2 sin^2⁡𝑥)/cos^2⁡𝑥 ) 𝑑𝑥〗 I=𝜋/2 ∫_0^𝜋▒〖(𝑠𝑒𝑐^2 𝑥)/(𝑎^2 + 𝑏^2 tan^2⁡𝑥 ) 𝑑𝑥〗 Let 𝑓(𝑥)=sec^2⁡𝑥/(𝑎^2 + 𝑏^2 tan^2⁡𝑥 ) and a = π Now, 𝑓(2𝑎−𝑥)=sec^2⁡(𝜋 − 𝑥)/(𝑎^2 + 𝑏^2 tan^2⁡(𝜋 − 𝑥) ) 𝑓(2𝑎−𝑥)=[−𝑠𝑒𝑐 𝑥]^2/(𝑎^2 + 𝑏^2 [−tan⁡𝑥 ]^2 ) 𝑓(2𝑎−𝑥)=(𝑠𝑒𝑐^2 𝑥)/(𝑎^2 + 𝑏^2 tan^2⁡𝑥 ) Therefore, 𝑓(𝑥)=𝑓(2𝑎−𝑥) Therefore, I=𝜋/2 ∫_0^𝜋▒〖(𝑠𝑒𝑐^2 𝑥)/(𝑎^2 + 𝑏^2 tan^2⁡𝑥 ) 𝑑𝑥〗 =𝜋/2 × 2 ∫_0^(𝜋/2)▒〖(𝑠𝑒𝑐^2 𝑥)/(𝑎^2 + 𝑏^2 tan^2⁡𝑥 ) 𝑑𝑥〗 =𝜋∫_0^(𝜋/2)▒〖(𝑠𝑒𝑐^2 𝑥)/(𝑎^2 + 𝑏^2 tan^2⁡𝑥 ) 𝑑𝑥〗 Let 𝑏 tan⁡〖𝑥=𝑡〗 Differentiating both sides w.r.t. 𝑥 𝑏 𝑠𝑒𝑐^2 𝑥 𝑑𝑥=𝑑𝑡 𝑑𝑡=𝑑𝑡/(𝑏^2 𝑠𝑒𝑐^2 𝑥) Putting the values of tan 𝑥 and 𝑑𝑥 , we get 𝐼=𝜋∫1_0^(𝜋/2)▒〖(𝑠𝑒𝑐^2 𝑥)/(𝑎^2 + 𝑡^2 ) . 𝑑𝑥〗 𝐼=𝜋 ∫1_0^∞▒〖(𝑠𝑒𝑐^2 𝑥)/(𝑎^2 + 𝑡^2 ) .𝑑𝑡/(𝑏 𝑠𝑒𝑐^2 𝑥)〗 𝐼=𝜋/𝑏 ∫1_0^∞▒𝑑𝑡/(𝑎^2 + 𝑡^2 ) 𝐼= 〖𝜋/𝑏 [1/𝑎 tan^(−1)⁡(𝑡/𝑎) ]〗_0^∞ Putting limits, I=𝜋/𝑏 [1/𝑎 〖𝑡𝑎𝑛〗^(−1) (∞/𝑎)−1/𝑎 〖𝑡𝑎𝑛〗^(−1) (0/𝑎)] I =𝜋/𝑏 [〖1/𝑎 tan^(−1)〗⁡〖(∞)−1/𝑎 tan^(−1)⁡(0) 〗 ] I =𝜋/𝑏 (1/𝑎 (𝜋/2)−0) I =𝝅^𝟐/𝟐𝒂𝒃