Maths Crash Course - Live lectures + all videos + Real time Doubt solving!
Ex 10.2, 20 - Chapter 10 Class 11 Straight Lines (Term 1)
Last updated at May 29, 2018 by Teachoo
Ex 10.2
Ex 10.2, 1
Important
Ex 10.2, 2
Ex 10.2, 3
Ex 10.2, 4 Important
Ex 10.2, 5
Ex 10.2, 6 Important
Ex 10.2, 7
Ex 10.2, 8 Important
Ex 10.2, 9 Important
Ex 10.2, 10
Ex 10.2, 11 Important
Ex 10.2, 12
Ex 10.2, 13
Ex 10.2, 14 Important
Ex 10.2, 15 Important
Ex 10.2, 16
Ex 10.2, 17 Important
Ex 10.2, 18 Important
Ex 10.2, 19 Important
Ex 10.2, 20 You are here
Transcript
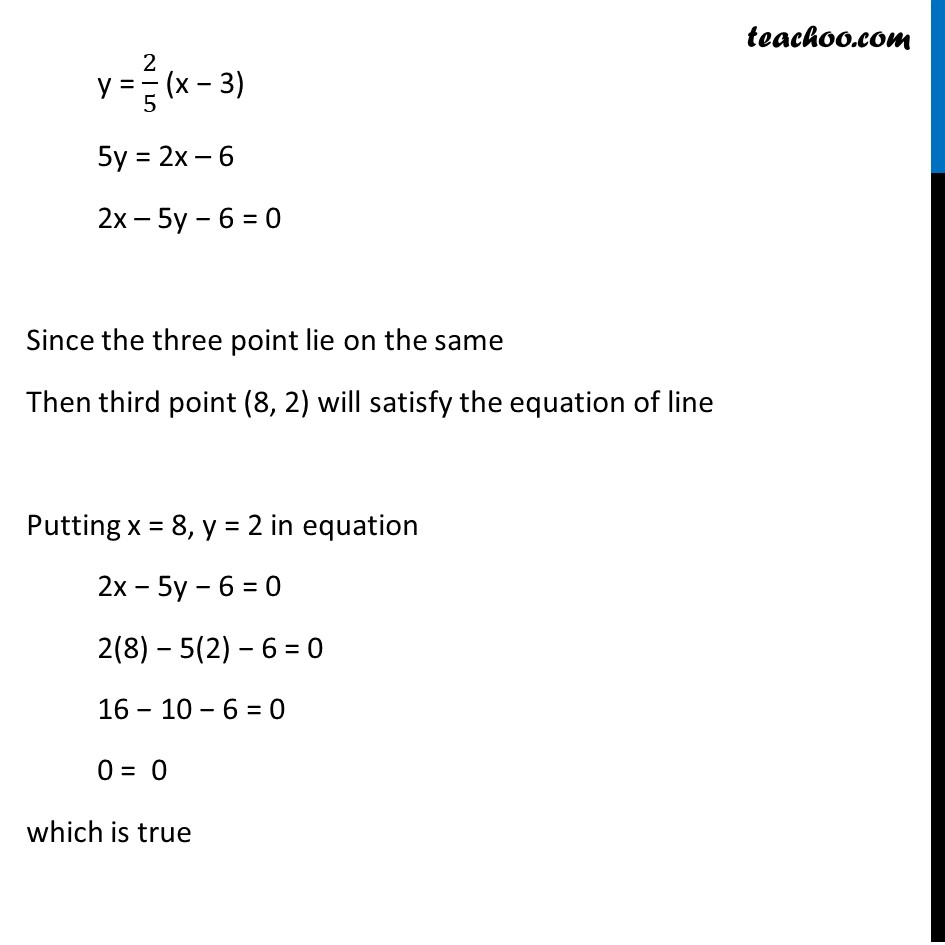
Ex 10.2, 20 By using the concept of equation of a line, prove that the three points (3, 0), ( 2, 2) and (8, 2) are collinear. We need to prove that the three point (3, 0), ( 2, 2), and (8, 2) are collinear i.e. these three points lie on the same line We check if (8, 2) lies on the line made by the points (3, 0) & ( 2, 2) We know that equation of line passing through (x1, y1) & (x2, y2) (y y1) = 2 1 2 1 (x x1) Equation of line passing though (3, 0) & ( 2, 2) (y 0) = ( 2 0) ( 2 3) (x 3) y = 2 5 (x 3) y = 2 5 (x 3) 5y = 2x 6 2x 5y 6 = 0 Since the three point lie on the same Then third point (8, 2) will satisfy the equation of line Putting x = 8, y = 2 in equation 2x 5y 6 = 0 2(8) 5(2) 6 = 0 16 10 6 = 0 0 = 0 which is true Hence point (8, 2) lie on line 2x 5y 6 = 0 Hence points (8, 2), (3, 0) & ( 2, 2) on the same line Hence (3, 0), ( 2, 2)& (8, 2) are collinear
