Ex 9.2, 4 - Chapter 9 Class 11 Straight Lines

Transcript

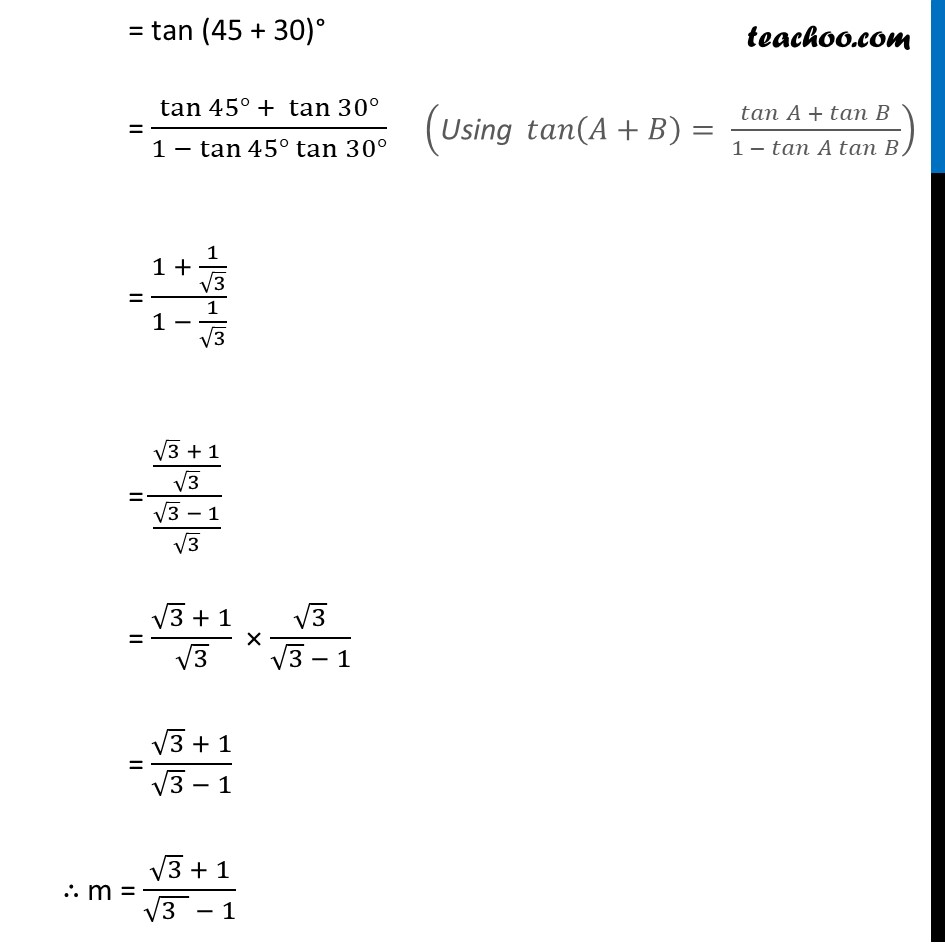
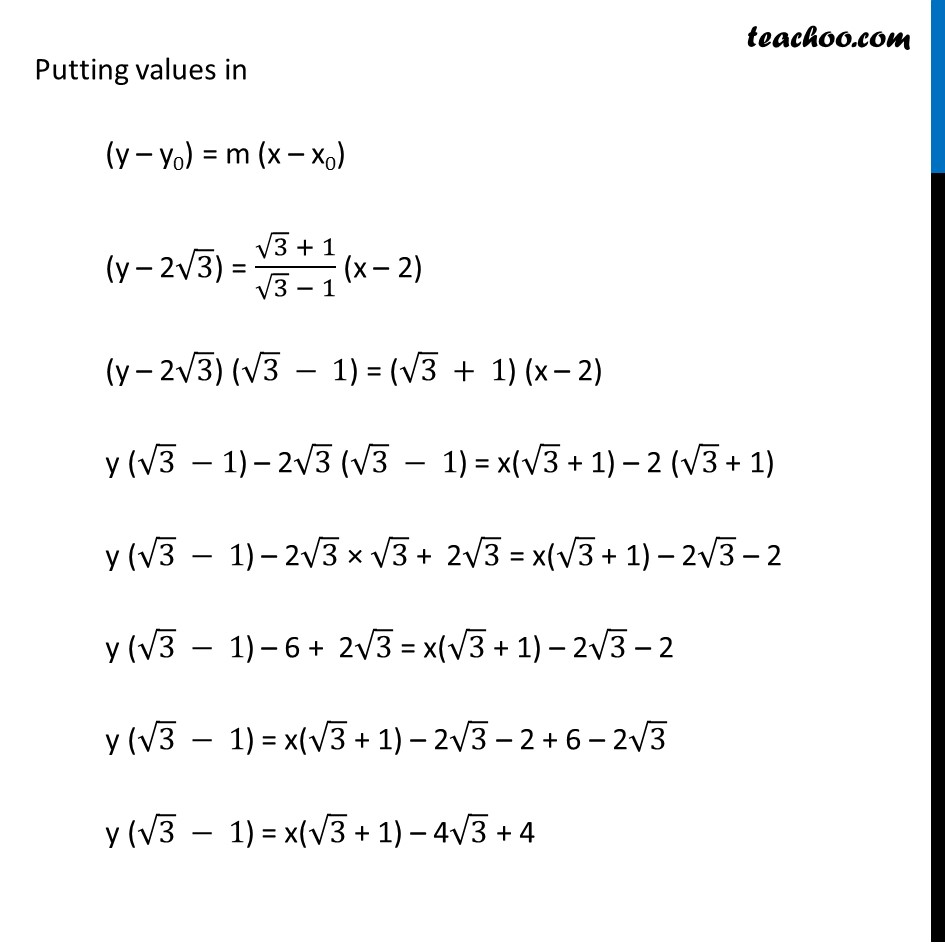
Ex 9.2, 4 Find the equation of the line which passes though (2, 2√3) and is inclined with the x-axis at an angle of 75°. We know that equation of line passing through point (x0, y0) with slope m is y – y0 = m(x – x0) Here Point (x0, y0) = (2, 2√3) Hence x0 = 2, y0 = 2√3 And slope = m = tan θ Given θ = 75° ∴ m = tan(75°) = tan (45 + 30)° = tan⁡〖45° + 〖 tan〗⁡〖30°〗 〗/(1 − tan⁡〖45°tan⁡〖30°〗 〗 ) = (1 + 1/√3)/(1 − 1/√3) = ((√3 + 1)/√3)/((√3 − 1)/√3) = (√3 + 1)/√3 × √3/(√3 − 1) = (√3 + 1)/(√3 − 1) ∴ m = (√3 + 1)/(√(3 ) − 1) ("Using " 𝑡𝑎𝑛⁡〖(𝐴+𝐵)= 𝑡𝑎𝑛⁡〖𝐴 + 𝑡𝑎𝑛⁡𝐵 〗/(1 − 𝑡𝑎𝑛⁡〖𝐴 𝑡𝑎𝑛⁡𝐵 〗 )〗 ) Putting values in (y – y0) = m (x – x0) (y – 2√3) = (√3 + 1)/(√3 − 1) (x – 2) (y – 2√3) (√3 − 1) = (√3 + 1) (x – 2) y (√3 −1) – 2√3 (√3 − 1) = x(√3 + 1) – 2 (√3 + 1) y (√3 − 1) – 2√3 × √3 + 2√3 = x(√3 + 1) – 2√3 – 2 y (√3 − 1) – 6 + 2√3 = x(√3 + 1) – 2√3 – 2 y (√3 − 1) = x(√3 + 1) – 2√3 – 2 + 6 – 2√3 y (√3 − 1) = x(√3 + 1) – 4√3 + 4 y (√3 − 1) – x (√3 + 1) = –4√3 + 4 y (√3 − 1) – x (√3 + 1) = 4(–√3 + 1) x (√𝟑 + 1) – y(√𝟑 – 1) = 4(√𝟑 − 1)