

Ex 1.1
Ex 1.1, 1 (ii)
Ex 1.1, 1 (iii) Important
Ex 1.1, 1 (iv)
Ex 1.1, 1 (v)
Ex 1.1, 2
Ex 1.1, 3
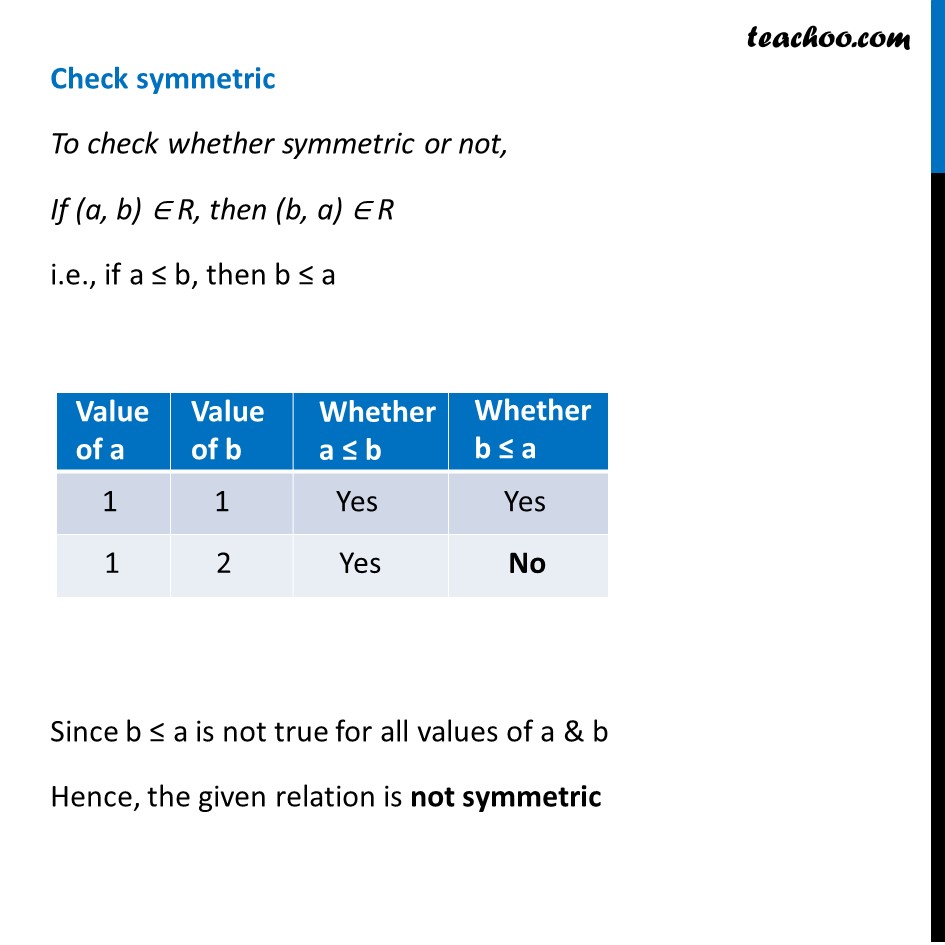
Ex 1.1, 4 You are here
Ex 1.1, 5 Important
Ex 1.1, 6
Ex 1.1, 7
Ex 1.1, 8
Ex 1.1, 9 (i)
Ex 1.1, 9 (ii)
Ex 1.1, 10 (i)
Ex 1.1, 10 (ii)
Ex 1.1, 10 (iii) Important
Ex 1.1, 10 (iv)
Ex 1.1, 10 (v)
Ex 1.1, 11
Ex 1.1, 12 Important
Ex 1.1, 13
Ex 1.1, 14
Ex 1.1, 15 (MCQ) Important
Ex 1.1, 16 (MCQ)
Last updated at April 16, 2024 by Teachoo



Ex 1.1, 4 Show that the relation R in R defined as R = {(a, b) : a ≤ b}, is reflexive and transitive but not symmetric. R = { (a, b) : a ≤ b } Here R is set of real numbers Hence, both a and b are real numbers Check reflexive We know that a = a ∴ a ≤ a ⇒ (a, a) ∈ R ∴ R is reflexive. Check symmetric To check whether symmetric or not, If (a, b) ∈ R, then (b, a) ∈ R i.e., if a ≤ b, then b ≤ a Since b ≤ a is not true for all values of a & b Hence, the given relation is not symmetric Check transitive If a ≤ b, & b ≤ c , then a ≤ c ∴ If (a, b) ∈ R & (b, c) ∈ R , then (a, c) ∈ R Hence, the given relation is transitive Hence, R = {(a, b) : a ≤ b}, is reflexive and transitive but not symmetric