Ex 1.1, 1 (iv) - Chapter 1 Class 12 Relation and Functions
Last updated at April 16, 2024 by Teachoo
Ex 1.1
Ex 1.1, 1 (i)
Ex 1.1, 1 (ii)
Ex 1.1, 1 (iii) Important
Ex 1.1, 1 (iv) You are here
Ex 1.1, 1 (v)
Ex 1.1, 2
Ex 1.1, 3
Ex 1.1, 4
Ex 1.1, 5 Important
Ex 1.1, 6
Ex 1.1, 7
Ex 1.1, 8
Ex 1.1, 9 (i)
Ex 1.1, 9 (ii)
Ex 1.1, 10 (i)
Ex 1.1, 10 (ii)
Ex 1.1, 10 (iii) Important
Ex 1.1, 10 (iv)
Ex 1.1, 10 (v)
Ex 1.1, 11
Ex 1.1, 12 Important
Ex 1.1, 13
Ex 1.1, 14
Ex 1.1, 15 (MCQ) Important
Ex 1.1, 16 (MCQ)
Transcript
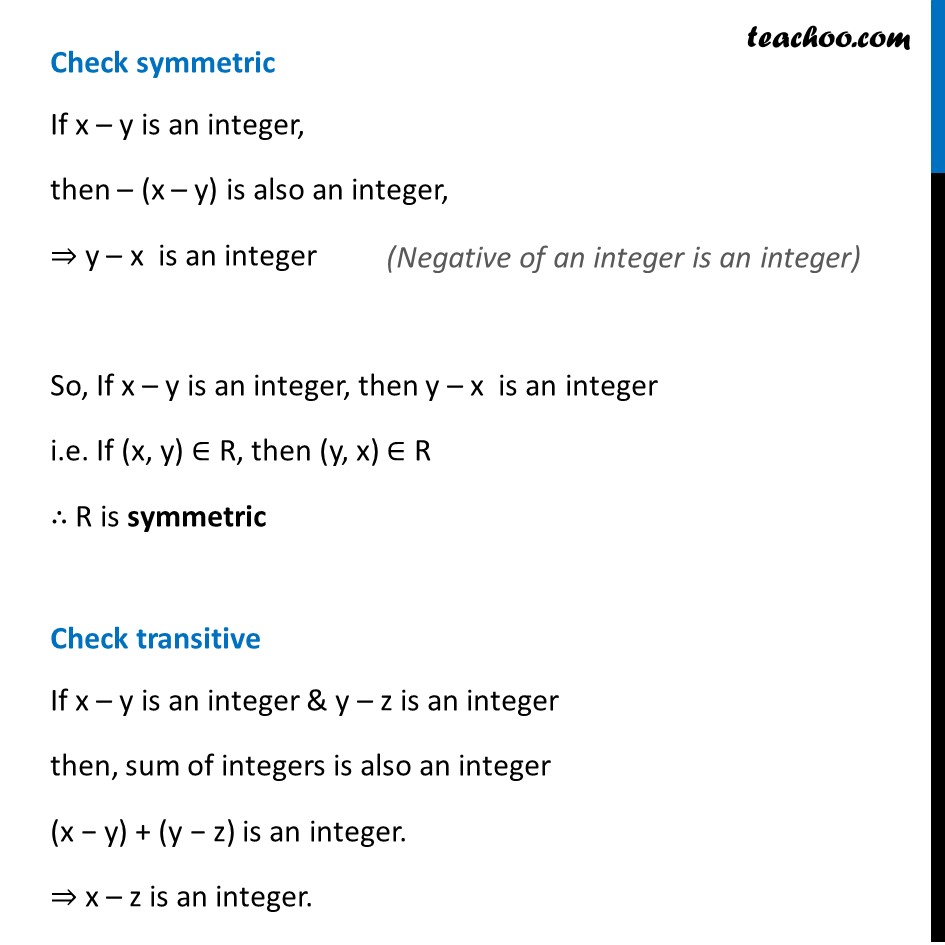
Ex 1.1, 1 Determine whether each of the following relations are reflexive, symmetric and transitive: (iv) Relation R in the set Z of all integers defined as R = {(x, y): x − y is as integer} R = {(x, y): x − y is as integer} Check Reflexive Since, x – x = 0 & 0 is an integer ∴ x – x is an integer ⇒ (x, x) ∈ R ∴ R is reflexive Check symmetric If x – y is an integer, then – (x – y) is also an integer, ⇒ y – x is an integer So, If x – y is an integer, then y – x is an integer i.e. If (x, y) ∈ R, then (y, x) ∈ R ∴ R is symmetric Check transitive If x – y is an integer & y – z is an integer then, sum of integers is also an integer (x − y) + (y − z) is an integer. ⇒ x – z is an integer. So, If x – y is an integer & y – z is an integer then, x – z is an integer. ∴ If (x, y) ∈ R & (y, z) ∈ R , then (x, z) ∈ R ∴ R is transitive Hence, R is reflexive, symmetric, and transitive.
