Example 27 - Chapter 5 Class 12 Continuity and Differentiability

Transcript

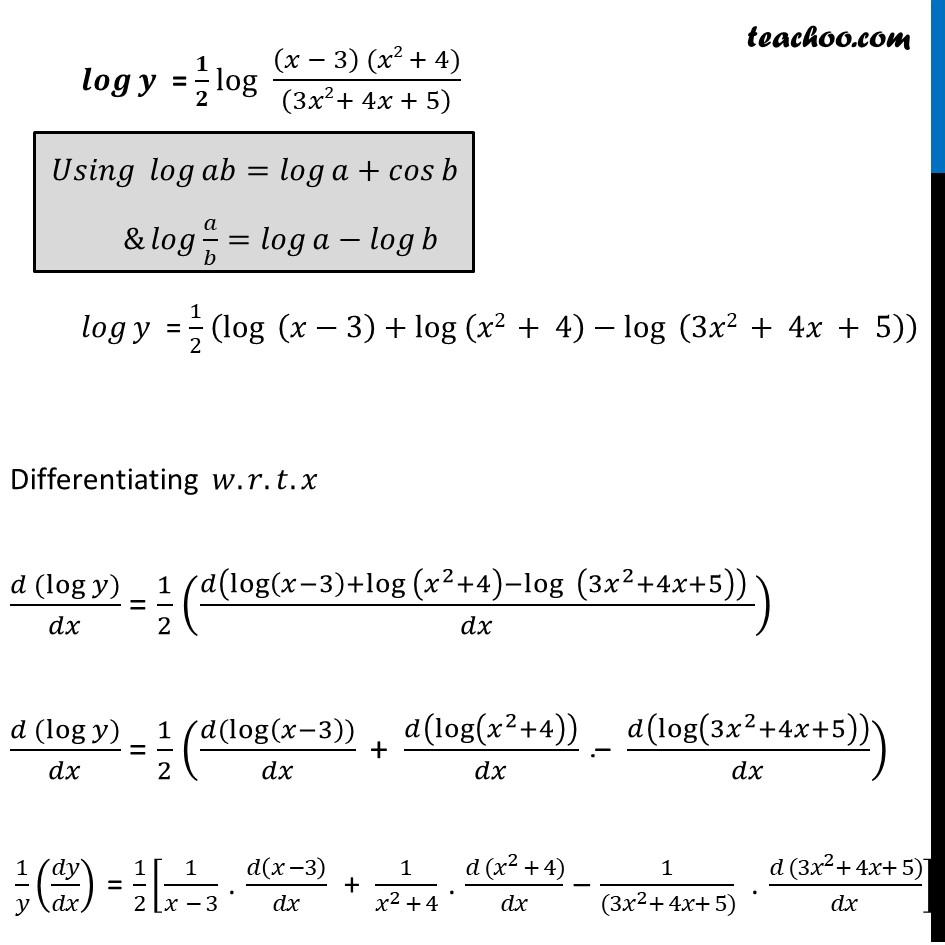
Example 27 Differentiate √(((𝑥−3) (𝑥2+4))/( 3𝑥2+ 4𝑥 + 5)) 𝑤.𝑟.𝑡. 𝑥. Let y =√(((𝑥 − 3) (𝑥^2 + 4))/( 3𝑥2+ 4𝑥 + 5)) Taking log on both sides 𝒍𝒐𝒈⁡𝒚 = 𝒍𝒐𝒈 √(((𝒙 − 𝟑) (𝒙𝟐 + 𝟒))/( 𝟑𝒙𝟐+ 𝟒𝒙 + 𝟓)) log⁡𝑦 = log⁡ (((𝑥 − 3) (𝑥2 + 4))/( 3𝑥2+ 4𝑥 + 5))^(1/2) 𝒍𝒐𝒈⁡𝒚 = 𝟏/𝟐 log ((𝑥 − 3) (𝑥2 + 4))/((3𝑥2+ 4𝑥 + 5) ) (𝑈𝑠𝑖𝑛𝑔 log⁡〖𝑎^𝑏 〗=𝑏 log⁡𝑎) 𝒍𝒐𝒈⁡𝒚 = 𝟏/𝟐 log ((𝑥 − 3) (𝑥2 + 4))/((3𝑥2+ 4𝑥 + 5) ) 𝑙𝑜𝑔⁡𝑦 = 1/2 (log⁡〖 (𝑥−3)〗+〖log 〗⁡(𝑥2 + 4)−log⁡〖 (3𝑥2 + 4𝑥 + 5)〗 ) Differentiating 𝑤.𝑟.𝑡.𝑥 (𝑑 (log⁡𝑦))/𝑑𝑥 = 1/2 ((𝑑(log⁡(𝑥−3)+〖log 〗⁡〖(𝑥^2+4)−log⁡〖 (3𝑥^2+4𝑥+5)〗 〗 ) )/𝑑𝑥) (𝑑 (log⁡𝑦))/𝑑𝑥 = 1/2 ((𝑑(log⁡(𝑥−3)))/𝑑𝑥 " + " 𝑑(log⁡(𝑥^2+4) )/𝑑𝑥 " − " 𝑑(log⁡(3𝑥^2+4𝑥+5) )/𝑑𝑥) 1/𝑦 (𝑑𝑦/𝑑𝑥) = 1/2 [1/(𝑥 − 3) " . " 𝑑(𝑥 −3)/𝑑𝑥 " + " 1/(𝑥^2 + 4) " . " (𝑑 (𝑥^2 + 4))/𝑑𝑥 " – " 1/((3𝑥^2+ 4𝑥+ 5)) " . " (𝑑 (3𝑥^2+ 4𝑥+ 5))/(𝑑𝑥 )] 𝑈𝑠𝑖𝑛𝑔 𝑙𝑜𝑔⁡𝑎𝑏=𝑙𝑜𝑔⁡𝑎+𝑐𝑜𝑠⁡𝑏 &𝑙𝑜𝑔⁡〖𝑎/𝑏〗=𝑙𝑜𝑔⁡〖𝑎−𝑙𝑜𝑔⁡𝑏 〗 1/𝑦 (𝑑𝑦/𝑑𝑥) = 1/2 [1/((𝑥 − 3) ) " " (1−0) "+ " 1/(𝑥^2+ 4) " " (2𝑥 + 0)" − " 1/(3𝑥^2+ 4𝑥 + 5) " " (6𝑥 +4+0)" " ] 1/𝑦 (𝑑𝑦/𝑑𝑥) = 1/2 (1/((𝑥 − 3) )+2𝑥/(𝑥^2+ 4)−(6𝑥 + 4)/(3𝑥^2 + 4𝑥 + 5)) 𝑑𝑦/𝑑𝑥 = 𝑦/2 (1/((𝑥 − 3) )+2𝑥/(𝑥^2+ 4)−(6𝑥 + 4)/(3𝑥^2 + 4𝑥 + 5)) 𝒅𝒚/𝒅𝒙 = 𝟏/𝟐 √(((𝒙 − 𝟑)(𝒙^𝟐+ 𝟒))/(𝟑𝒙^𝟐+ 𝟒𝒙 + 𝟓)) (𝟏/((𝒙 − 𝟑) )+𝟐𝒙/(𝒙^𝟐+ 𝟒)−(𝟔𝒙 + 𝟒)/(𝟑𝒙^𝟐 + 𝟒𝒙 + 𝟓))